Nucleon sigma term from lattice QCD

1
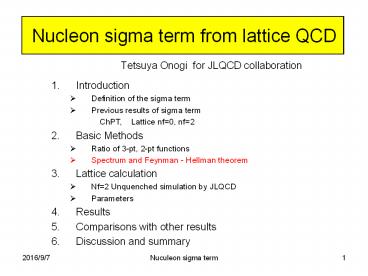
Nucleon sigma term from lattice QCD

• Tetsuya Onogi for JLQCD collaboration

• Introduction
• Definition of the sigma term
• Previous results of sigma term
• ChPT, Lattice nf0, nf2
• Basic Methods
• Ratio of 3-pt, 2-pt functions
• Spectrum and Feynman - Hellman theorem
• Lattice calculation
• Nf2 Unquenched simulation by JLQCD
• Parameters
• Results
• Comparisons with other results
• Discussion and summary

2
Members of JLQCD Collaboration

• KEK S. Hashimoto, T. Kaneko, H.
  Matsufuru, J. Noaki,
• E. Shintani, N. Yamada
• RIKEN/Niels Bohr H. Fukaya
• Tsukuba S. Aoki, T. Kanaya, N. Ishizuka, Y.
  Taniguchi,
• A. Ukawa, T. Yoshie
• Hiroshima K.-I. Ishikawa, M. Okawa
• YITP H. Ohki, T. Onogi

3
Appologies

• All the results are still very preliminary.
• Theoretical interpretation of the descrepancies
  between our lattice results and previous ones
  came out only recently, therefore if there is any
  misintepretation in my talk it is mostly due to
  my fault.

4
1. Introduction

• Definitions of the nucleon sigma term
• sigma term scalar form factor of the
  nucleon at zero recoil
• other related quantities

5

• Why sigma term is important?
• A crucial parameter for the dark matter detection
  rate
• dark matter interaction with nucleon
• by higgs exchange in the t-channel
• It is related to the chromo-electric
  contributions to neutron EDM

6

• Previous results

7
Our goal

• Determine the nucleon sigma term in
  unquenced QCD
• using the dynamical quark is overlap
  fermion,
• which has an exact chiral symmetry on the
  lattice
• The advantage of the exact chiral symmetry
• Theoretically much cleaner ? no additive mass
  shift
• No power divergence ? subtraction of the vacuum
  condensate
• is
  numerical much more stable
• - No unwanted operator mixing
• In this study, we work in nf2 unquenched QCD
• nf21 will be studied very soon
• We exploit mass spectrum method ( explained later
  )

8
Basic Methods

• Method 1 Ratio of 3-pt, 2-pt functions
• Define the following ratio of 3-pt,2pt
  functions
• Sigma term can be extracted
• from the contribution linear in as

9

Proof Insert the complete set of states and
look at the lowest state
10
Basic Methods

• Method 2 Nucleon mass spectrum
• Feynman - Hellman theorem

11
Proof differentiate 2pt function

• Differentiate the 2-pt function
• Then take the ratio with 2-pt function
• Extracting the term linear in
• gives the Feynman-Hellman theorem

12
Ratio method vs spectrum method

• They treat identical quantities the t-linear
  term of R(t).
• The only difference is that one take the
  derivative with respect quark mass before or
  after the path-integral. No fundamental
  advantage or disadvantage.
• In practice, the contamination from excited
  states is the source of systematic error
• Spectrum method is automatically gives
• the measurement of S for all spacetime points.

13

• From now on we will denote as
• for referring
• for the sake of brevity.

14
3. Lattice calculation

• Many unquenched simulations are performed or
  starting now.
• In addition to rooted staggered by MILC collab.,
• Wilson-type fermions and Ginsparg-Wilson fermions
  are in progress.
• Important for cross-check and theoretically clean
  

15
Ginsparg-Wilson fermion

• Ginsparg-Wilson relation
• Ginsparg and Wilson, Phys.Rev.D 25(1982)
  2649.
• Exact chiral symmetry on the lattice (index
  theorem)
• Hasenfratz, Laliena and Niedermayer,
  Phys.Lett. B427(1998) 125
• Luscher, Phys.Lett.B428(1998)342.
• Overlap fermion ( explicit construction )

16

• Problems(all related to the zeros of Hw)
• We make rational approximation
• with completely controlled error
• except near zero mode.
• Dov makes a discontinous jump when an eigenmode
  of Hw crosses zero. Hybrid Monte Carlo breaks
  down.
• A method to cure this problem has been developed.
  One has to monitor the zero crossing at
  much higher precision and include correction
  terms at the exact point of crossing. (
  Hopelessly huge numerical cost)

17

• JLQCDs strategy
• Topology conserving Det(Hw) term
• Fukaya, Vranas, Fukaya et al.
  hep-lat/0607020
  
• Introduce negative heavy mass wilson
  fermion as a UV regulator field, whose mass is
  exactly the same as that appears in Dov. Infrared
  physics is unchanged.
• This term should kill the breakdown of
  locality topology change, and blow-up of
  numerical cost simultaneouly.

18
Status of JLQCD2 GW project

• KEK BlueGene (10 racks, 57.3 TFlops)
• Started on March 1, 2006
• 1rack1024 nodes2048CPU
• PowerPC440(700MHz,2.8Gflops)
• 1node2CPU, 4MB L3 cache,
• 512MB memory
• network 3D torus(half-rack)
• (8x8x8) global tree
• 243x48 Wilson fermion inversion
• sustained speed 28 of the peak speed
• 163x32 slightly lower sustained speed

19
Numerical simulation

• Dynamical simulation with Nf2 overlap fermion
• Run1 (epsilon-regime)
• 163 x 32 , 0.11 fm
• quark mass around 3MeV!!
• Fixed topology
• Run2 (normal regime)
• 163 x 32, a0.12 fm
• quark mass 6 values in the range of ms/6-ms
• fixed topology
• At Q0 accumulated 10,000 trajectories

20
QCD in regime (Run1)

• Eigenmode distribution is consistent with Chiral
  Random Matrix model up to finite volume
  corrections.
• 
  Fukaya et al. hep-lat/0702003

Cumulative distribution of low eigenvalues
Low Eigenvalue ratios
21
QCD in normal regime (Run2)

• Nf2

Quark mass dependence of the pion mass
Quark mass dependence of the decay const
22
Parameters for our study

• We have 6 and 9 quark masses
• for the sea and valences quarks, respectively.
• We only use data with

23
4. Results

• Nucleon masses from 2-pt functions

Nice plateau for t gt4 We fit the 2-pt function
with a single expoential function with fitting
range t5-10
Effective mass plot for amq0.035 Solid lines
are the mass from the fit
24
Sea and valence quark mass dependences

• The valence quark mass dependence is very clear,
• while the sea quark mass dependence is small.

25
Fit of the quark mass dependence

• global fit
• fit 1
• fit 2

26
Fit of the quark mass dependence

• fit of diagonal (unitary) points
• fit3 (ChPT)
• This analysis is similar to Procula et al (2004).

27
Nucleon mass (fit 2)

• Nucleon mass in the chiral limit is
• 10 larger than the experimental value.
• But, consistent with CP-PACS nf2 result
• Possible source of deviation
• Finite size effect
• nf2 effect
• Chiral extrapolation error
• (ChPT analysis is necessary)

28
Nucleon mass (fit 3)

Nucleon mass in the chiral limit is Consistent
with the experimental value.
29
Connected contribution to (fit2)

30
Disconnected contribution to (fit2)

31
Total sigma term

• Fit 2 (Polynomial)
• Fit 3 (ChPT)

32
Disconnected contribution to (fit2)

33
Comparison with other results

• ChPT results and previous results are
  consistent.
• Our results with fit3 (ChPT) is consistent
• Previous lattice calculation Disc/Conn. Is larger
  than 1
• Our lattice calculation Disc/Conn is about 0.1
• ChPT predicts
• Previous lattice results due
  to large disconnected
• contribution
• Our results with fit3 (ChPT) gives

34
Why is y so different ?

• ChPT
• Large uncertainty from LEC
• Previous lattice caculation
• Why disconnected contribution is so large?
• mixing with wrong chirality?

35
Operator mixing due to Wilson fermion artifact

• If there is an additive mass shift there can
  be operator mixing which should be subtracted
  (but not subtracted except for UKQCD) for
  disconnected diagram.
• Subtracting this mixing effect by using the sea
  quark mass dependence of the quark mass shift,
  the disconnected contribution becomes tiny (
  consistent with zero).

36
Discussion and summary

• We studied the nucleon mass spectrum
• for nf2 unquenched QCD using exactly chirally
  symmetric dynamical fermion.
• It is expected that our calculation is free from
  dangerous lattice artifacts ( power divergence,
  operator mixing )
• Our result is consistent with ChPT prediction.
• We found disconnected (strange quark content )
  part is tiny.
• We pointed out that the descrepancies from
  previous lattice calculation can come from
  artifact in Wilson fermion.

37
Back up slides
38
Sea and valence quark mass dependences

• The valence quark mass dependence is very clear,
• while the sea quark mass dependence is small.

39
Nucleon mass

• Nucleon mass in the chiral limit is
• 7 larger than the experimental value.
• But, consistent with CP-PACS nf2 result
• Possible source of deviation
• Finite size effect
• nf2 effect
• Chiral extrapolation error
• (ChPT analysis is necessary)

40
Connected contribution to

41
Disconnected contribution to

42
Disconnected contribution to

43
Disconnected contribution to