Kinetics of Elementary Reactions

1
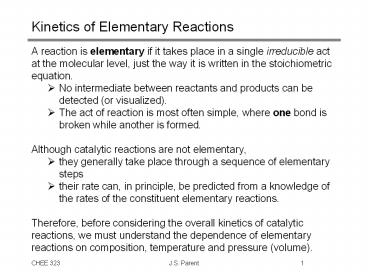
Kinetics of Elementary Reactions

• A reaction is elementary if it takes place in a
  single irreducible act at the molecular level,
  just the way it is written in the stoichiometric
  equation.
• No intermediate between reactants and products
  can be detected (or visualized).
• The act of reaction is most often simple, where
  one bond is broken while another is formed.
• Although catalytic reactions are not elementary,
• they generally take place through a sequence of
  elementary steps
• their rate can, in principle, be predicted from a
  knowledge of the rates of the constituent
  elementary reactions.
• Therefore, before considering the overall
  kinetics of catalytic reactions, we must
  understand the dependence of elementary reactions
  on composition, temperature and pressure (volume).

2
Elementary Reactions

• Since an elementary reaction represents a
  molecular event, its equation may not be written
  arbitrarily, but the way it takes place.
• With this restriction, the molecularity of the
  reaction is identical to its stoichiometry.

3
Theories of Elementary Reaction Kinetics

• The rates of even the simplest reactions are very
  difficult to calculate from first principles. In
  engineering practice, you will rely on
  experimental data.
• While the basic science of reaction kinetics is
  not sufficiently developed for design purposes,
  existing models of reaction dynamics provide a
  means of understanding reaction phenomena,
  analyzing experimental data, and extrapolating
  knowledge to other systems.
• Atkins details three approaches to the
  calculation of rate constants
• Collision Theory
• Transition State Theory
• Molecular Reaction Dynamics
• We will examine the collision and transition
  state theories.

4
Transition State Theory - Elementary Reactions

• Transition state theory is founded on the
  expectation that during the transition from
  initial reagents to final products, an activated
  complex of higher energy is formed.
• This transition state is not an intermediate,
  but a unique configuration of the system in
  transit from one state to another.
• Although this activated complex is inherently
  unstable, we often assume that it possesses
  thermodynamic properties (albeit ill-defined),
  and propose molecular structures.

5
Transition State of an SN2 Reaction

• You have likely seen the concept of a transition
  state in CHEM 288, where nucleophilic
  substitution reactions were introduced.
• In the example below, the alkoxide ion is the
  nucleophile (Lewis base) displaces iodide, the
  weaker base.
• The reaction is believed to be bimolecular,
  passing through a transition state as drawn
  below
• Clearly this transition state is not a stable
  compound, and therefore is not a reaction
  intermediate, but an activated complex.

6
Potential Energy Surface for Hydrogen Exchange

• Owing to the complexity of potential energy
  calculations, one of the only systems to be
  analyzed is that of collinear hydrogen exchange.

7
Potential Energy of the Reaction Coordinate

8
Transition State Theory - Thermodynamic
Formulation

• The Rate of an Elementary Step
• The number of elementary acts per unit time is
  determined the number of systems passing through
  the activated complex configuration.
• We express the elementary reaction as
• At equilibrium, the activated complex Xy will be
  in equilibrium with the reactants and products,
  and the concentration can be calculated from
  thermodynamic principles.
• Where q is the reference concentration, usually 1
  mole/litre.
• Transition state theory assumes that even when
  the system is not at equilibrium, activated
  complexes are at equilibrium with the reactants.

9
Transition State Theory - Thermodynamic
Formulation

• Based on this assumption, the concentration of
  the activated complex is derived from a
  thermodynamic treatment
• q unit concn
• which, can be expressed in terms of the relative
  Gibbs energy of the activated complex,
• DGy represents the free energy of activation.
• The difference between the Gibbs energy of the
  activated complex, and the Gibbs energies of the
  reactants at the reference state
• This represents the free energy barrier to
  reaction that includes both potential energy (DH)
  and conformational restrictions (DS).

10
Transition State Theory - Thermodynamic
Formulation

• The rate of the forward elementary reaction
• is expressed as
• q unit concn
• where n is the frequency of vibration of the
  activated complex in the mode that corresponds to
  decomposition into products.
• This is the frequency of the molecular vibration
  which leads the complex to dissociate into
  products C and D.
• For this diatomic reaction, statistical mechanics
  assigns
• sec-1
• where kb Boltzmanns constant 1.3806610-23
  J/K
• T reaction temperature, K
• h Plancks constant 6.626210-34 J s

11
Transition State Theory - Thermodynamic
Formulation

• With a measure of the decomposition frequency,
  the rate of our elementary reaction takes the
  form
• Given our elementary rate expression for the
  reaction,
• The rate constant, k, for the reaction is
  identifiable as
• q unit concn
• which ends our development of transition state
  theory. It correctly predicts the orders of the
  reaction, provides a means of interpreting the
  observed rate in terms of enthalpic and entropic
  contributions, and provides guidelines into the
  temperature dependence of k.

12
Temperature Dependence of Elementary Reactions

• The variation of elementary reaction rate
  constants with temperature is almost always
  expressed as
• The term Ea is usually called the activation
  energy, although interpretations of this quantity
  differ between specific theories of reaction
  rate. The temperature exponent, m, does
  likewise.
• m 0 corresponds to classical Arrhenius theory
• m 1/2 is predicted by collision theory
• m 1 is generated by transition state theory
• In practice, the dependence of the
  pre-exponential factor on temperature is usually
  much weaker than that of the activation energy.
• If gathered under kinetic control, reaction rate
  data plotted as ln(k) versus 1/T or ln(k/T)
  versus 1/T is usually linear.

13
Temperature Dependence of Elementary Reactions