The%20General%202k%20Factorial%20Design

1
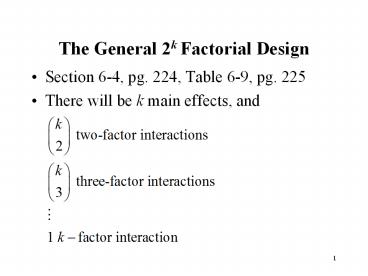
The General 2k Factorial Design

• Section 6-4, pg. 224, Table 6-9, pg. 225
• There will be k main effects, and

2
General Procedure for a 2k Factorial Design

• Estimate factor effects (sign magnitude)
• From initial model (usually the full model)
• Perform statistical testing (ANOVA)
• Refine model (removing nonsignificant variables)
• Analyze residuals (model adequacy/assumptions
  checking)
• Refine model if its necessary
• Interpret results (main/interaction effects
  plots, contour plots, response surfaces)

3
Unreplicated 2k Factorial Designs

• These are 2k factorial designs with one
  observation at each corner of the cube
• An unreplicated 2k factorial design is also
  sometimes called a single replicate of the 2k
• These designs are very widely used
• Risksif there is only one observation at each
  corner, is there a chance of unusual response
  observations spoiling the results?
• Modeling noise?

4
Spacing of Factor Levels in the Unreplicated 2k
Factorial Designs
If the factors are spaced too closely, it
increases the chances that the noise will
overwhelm the signal in the data More aggressive
spacing is usually better
5
Unreplicated 2k Factorial Designs

• Lack of replication causes potential problems in
  statistical testing
• Replication admits an estimate of pure error (a
  better phrase is an internal estimate of error)
• With no replication, fitting the full model
  results in zero degrees of freedom for error
• Potential solutions to this problem
• Pooling high-order interactions to estimate error
• Normal probability plotting of effects (Daniels,
  1959)
• Other methodssee text, pp. 234

6
Example of an Unreplicated 2k Design

• A 24 factorial was used to investigate the
  effects of four factors on the filtration rate of
  a resin
• The factors are A temperature, B pressure, C
  mole ratio/concentration, D stirring rate
• Experiment was performed in a pilot plant

7
The Resin Plant Experiment
8
The Resin Plant Experiment
9
Estimates of the Effects
Term Effect SumSqr Contribution Model
Intercept Error A 21.625 1870.56 32.6397 Er
ror B 3.125 39.0625 0.681608 Error
C 9.875 390.062 6.80626 Error
D 14.625 855.563 14.9288 Error
AB 0.125 0.0625 0.00109057 Error
AC -18.125 1314.06 22.9293 Error
AD 16.625 1105.56 19.2911 Error
BC 2.375 22.5625 0.393696 Error
BD -0.375 0.5625 0.00981515 Error
CD -1.125 5.0625 0.0883363 Error
ABC 1.875 14.0625 0.245379 Error
ABD 4.125 68.0625 1.18763 Error
ACD -1.625 10.5625 0.184307 Error
BCD -2.625 27.5625 0.480942 Error
ABCD 1.375 7.5625 0.131959
10
The Normal Probability Plot of Effects
11
The Half-Normal Probability Plot
12
ANOVA Summary for the Model
Response Filtration Rate ANOVA for
Selected Factorial Model Analysis of variance
table Partial sum of squares Sum
of Mean F Source Squares DF Square Value Prob
gtF Model 5535.81 5 1107.16 56.74 lt
0.0001 A 1870.56 1 1870.56 95.86 lt
0.0001 C 390.06 1 390.06 19.99 0.0012 D 855.56 1
855.56 43.85 lt 0.0001 AC 1314.06 1 1314.06 67.34
lt 0.0001 AD 1105.56 1 1105.56 56.66 lt
0.0001 Residual 195.12 10 19.51 Cor
Total 5730.94 15 Std. Dev. 4.42 R-Squared 0.966
0 Mean 70.06 Adj R-Squared 0.9489 C.V. 6.30 Pr
ed R-Squared 0.9128 PRESS 499.52 Adeq
Precision 20.841
13
The Regression Model
Final Equation in Terms of Coded Factors
Filtration Rate 70.06250 10.81250
Temperature 4.93750 Concentration 7.31250
Stirring Rate -9.06250 Temperature
Concentration 8.31250 Temperature Stirring
Rate
14
Model Residuals are Satisfactory
15
Model Interpretation Interactions
16
Model Interpretation Cube Plot
If one factor is dropped, the unreplicated 24
design will project into two replicates of a 23 A
unreplicated 2k design, if h (hltk) factors are
negligible, then the original data -gt a full
two-level factorial 2k-h with 2h
replicates. Design projection is an extremely
useful property, carrying over into fractional
factorials
17
The Resin Plant Experiment
18
The Resin Plant Experiment Projected Design
Analysis
19
Model Interpretation Response Surface Plots
With concentration at either the low or high
level, high temperature and high stirring rate
results in high filtration rates