Length contraction

1
Length contraction

• Length measured differs from frame to frame
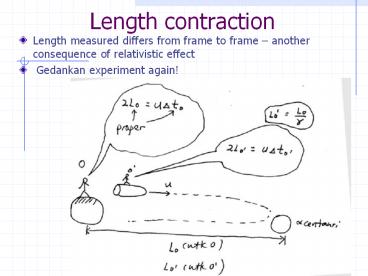
  another consequence of relativistic effect
• Gedankan experiment again!

2

• Two observers O on Earth, O traveling to and
  fro from Earth and alpha centauri with speed u
• Total distance between Earth - alpha centauri
  Earth, according to O (Earth observer), L0
• O sees O return to Earth after Dt0
• Observer O in a spaceship is heading AC with
  speed u and returns to Earth after Dt according
  to his clock

3
Use some simple logics

• In O 2L0 uDt0
• In O 2L0 uDt0
• Due to time dilation effect, Dt0 is shorter
  than Dt0 , i.e. Dt0 gt Dt0
• Dt0 is related to Dt0 via a time dilation
  effect, Dt0 Dt0 /g , hence
• L0 / L0 Dt0 /Dt0 1 / g , or

4

• L0 L0 / g
• L0 is defined as the proper length length of
  object measured in the frame in which the object
  (in this case, the distance btw Earth and AC) is
  at rest
• L0 is the length measured in the O frame, which
  is moving wrp to the object
• The length of a moving objected is measured to be
  shorter than the proper length length
  contraction

5

• If an observer at rest wrp to an object measures
  its length to be L0 , an observer moving with a
  relative speed u wrp to the object will find the
  object to be shorter than its rest length by a
  foctor 1 / g .

6

• A stick moves to the right with a speed u. (a)
  The stick as viewed by a frame attached to it (b)
  The stick as seen by an observer in a frame at
  rest relative to the stick. The length measured
  in the rest frame is shorter than the proper
  length by a factor 1/ g

7

• Length contraction only happens along the
  direction of motion
• In 3-D, the length contraction effect is a
  shortening of length plus a rotation

8
(No Transcript)
9
An observer on Earth sees a spaceship at an
altitude of 435 moving downward toward the Earth
with a speed of 0.97c. What is the altitude of
the spaceship as measured by an observer in the
spaceship?

• Solution
• One can consider the altitude seen by
  thestationary (Earth) observer as the proper
  length (say, L'). The observer in the spaceship
  should sees a contracted length, L, as compared
  to the proper length. Hence the moving observer
  in the ship finds the altitude to be
• L L' / g 435 m x 1- (0.97)2-1/2 106 m

10
Relativistic kinematics
11
Lorentz Transformation

• All inertial frames are equivalent
• All physical processes analysed in one frame can
  also be analysed in other inertial frame and
  yield consistent results
• A transformation law is required to related the
  space and time coordinates from one frame to
  another

12
(No Transcript)
13

• O' frame uses x',y',zt to denote the
  coordinates of an event, whereas O frame uses
  x,y,zt
• How to related x',y',z',t to x,y,zt
• In Newtonian mechanics, we use Galilean
  transformation
• But GT must not be valid when u ? c because it is
  not consistent with the constancy of the light
  speed postulate
• The relativistic version of the transformation
  law is given by Lorentz transformation

14
Derivation of Lorentz transformation
15

• Consider a rocket moving with a speed u (O'
  frame) along the xx' direction wrp to the
  stationary O frame
• A light pulse is emitted at the instant t' t 0
• when the two origins of the two reference frames
  coincide
• the light signal travels as a spherical wave at a
  constant speed c in both frames
• After some times t, the origin of the wave
  centered at O has a radius r ct, where
• r 2 x2 y2 z2

16

• From the view point of O', after some times t'
• the origin of the wave, centered at O' has a
  radius
• r' ct , (r )'2 (x) 2 (y)2 (z)2
• y'y, z' z (because the motion of O' is along
  the xx) axis no change for y,z coordinates
• The transformation from x to x (and vice versa)
  must be linear, i.e. x ? x
• From the view point of O
• x ct corresponds to x 0, so we assume the
  form
• x k(x - ct ) k some proportional constant
• Likewise, from the view point of O,
• x -ct corresponds to x 0, so we set
• x k(x ct )

17

• With
• r ct , r ct , x k(x ct ),
• x k(x - ct),
• we solve for x',t' in terms of x,t to
  obtain

18

• the constant k is identified as the Lorentz
  factor, g
• Note that, now, the length and time interval
  measured become dependent of the state of motion
  (in terms of g) in contrast to Newtons
  viewpoint
• Lorentz transformation reduces to Galilean
  transformation when u ltlt c (show this yourself)

19
To recap

• the LT given in the previous analysis

Which relates x , t to x, t , for which O is
moving at velocity u wrp to O
20
(No Transcript)
21
From the view point of O

• Equivalently, we could also perform the analysis
  from the view point of O that O is moving in the
  u direction.

22
From the view point of O
We wish to express x, t in terms of x, t
23
Mathematically, this simply means making the
permutation
The two transformations above are equivalent use
which is appropriate in a given question
24
Length contraction can be recovered from the LT

• Consider the rest length of a ruler as measured
  in frame O is L Dx x2 - x1 (the proper
  length) measured at the same instant in that
  frame, hence t2 t1
• What is the length of the rule as measured by O?
• The length in O, according the LT is
• L Dx x2 - x1 g (x2 - x1) u(t2
  -t1)
• The length of the ruler in O is simply the
  distance btw x2 and x1 measured at the same
  instant in that frame, hence t2 t1, hence L
  g L

25

• How would you recover time dilation from the LT?
• DIY as an exercise during Raya

26
Lorentz velocity transformation

• How to relate the velocity in the O (ux) frame
  to that of the O frame (ux)?

27

• By definition, ux dx/dt, ux dx/dt
• The velocity in the O frame can be obtained by
  taking the differentials of the Lorentz
  transformation,

28

• Combining

where we have made used of the definition ux
dx/dt
29
Compare the Lorentz transformation of velocity
with that of Galilean transformation of velocity
GT reduces to LT in the limit u ltlt c
30

• Please try to understand the definition of ux ,
  ux , u so that you wont get confused

31
LT is consistent with the constancy of speed of
light

• in either O or O frame, the speed of light seen
  must be the same, c
• Say object M is moving with speed of light as
  seen by O, i.e. ux c
• According to LT, the speed of M as seen by O is

32

• That is, in either frame, both observers agree
  that the speed of light they measure is the same,
  c 3 x 108m/s
• In contrast, according to GT, the speed of light
  seen by O would be

Which is inconsistent with constancy of speed of
light postulate
33
To recap

• the LT given in the previous analysis relates ux
  to ux in which O is moving with u wrp to O,

34
From the view point of O

• Equivalently, we could also perform the analysis
  from the view point of O that O is moving in the
  u direction.
• We would be able to express ux in terms of ux in
  the same spirit as we derive ux in terms of ux

35
From the view point of O
36
Mathematically, this simply means making the
permutation
The two transformations above are equivalent use
which is appropriate in a given question
37
Example

• Rocket 1 is approaching rocket 2 on a
• head-on collision course. Each is moving at
  velocity 4c/5 relative to an independent observer
  midway between the two. With what velocity does
  rocket 2 approaches rocket 1?
• C.f. In GT, their relative speed would just be
  4c/5 4c/5 1.6 c which violates constancy of
  speed of light postulate. See how LT handle this
  situation

38
(No Transcript)
39

• The observer in the middle is the stationary
  frame, O
• Choose rocket 1 as the moving frame O
• Call the velocity of rocket 2 as seen from rocket
  1 ux. This is the quantity we are interested in
• Frame O' is moving in the ve direction as seen
  in O, so u 4c/5
• The velocity of rocket 2 as seen from O is in the
  
• -ve direction, so ux - 4c/5
• Now, what is the velocity of rocket 2 as seen
  from frame O', u x ? (intuitively, u x must
  be in the negative direction)

40

• Use the LT

i.e. the velocity of rocket 2 as seen from rocket
1 (the moving frame, O) is 40c/41, which means
that O sees rocket 2 moving in the ve direction
(to the left in the picture), as expected.
41
Read other examples from the texts and also the
lecture notes
42
Selamat Hari Raya Aidilfitri