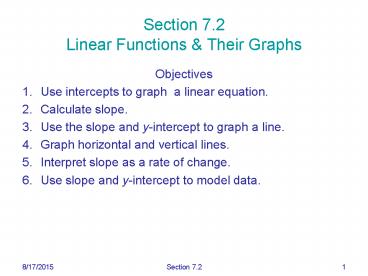
Section 7.2 Linear Functions

1
Section 7.2Linear Functions Their Graphs

• Objectives
• Use intercepts to graph a linear equation.
• Calculate slope.
• Use the slope and y-intercept to graph a line.
• Graph horizontal and vertical lines.
• Interpret slope as a rate of change.
• Use slope and y-intercept to model data.

2
Graphing Using Intercepts

• All equations of the form Ax By C are
  straight lines when graphed, as long as A and B
  are not both zero, and are called linear
  equations in two variables.
• The x-intercept is the point where the graph
  crosses the x-axis, i.e., when y 0.
• The y-intercept is the point where the graph
  crosses the y-axis, i.e., when x 0.
• Example Graph 3x 2y 6 by finding the
  intercepts.

3
Graphing Using InterceptsExample Continued

• Solution
• Find the x-intercept by
• letting y 0 and solving
• for x.
• 3x 2y 6
• 3x 2 0 6
• 3x 6
• x 2

Find the y-intercept by letting x 0 and
solving for y. 3x 2y 6 3 0 2y 6
2y 6 y 3
4
Graphing Using InterceptsExample Continued

• The x-intercept is 2, so the line passes through
  the point (2,0). The y-intercept is 3, so the
  line passes through the point (0,3).

Now, we verify our work by checking for x 1.
Plug in x 1 into the given linear equation. We
leave this to the student. For x 1, the
y-coordinate should be 1.5.
5
Slope

• The slope of the line through the distinct points
  (x1,y1) and (x2,y2) is
• where x2 x1 ? 0.
• Note, we let m denote slope.

6
SlopeUsing the Definition of Slope

• Example Find the slope of the line passing
  through the pair of points (-3,-1) and (-2,4).
• Solution Let (x1,y1) (-3,-1) and (x2,y2)
  (-2,4).
• We obtain the slope such that
• Thus, the slope of the line is 5.

7
The Slope-Intercept Form of the Equation of a Line

• The slope-intercept form of the equation of a
  nonvertical line with slope m and y-intercept b
  is
• y mx b.
• Example

8
The Slope-Intercept Form of the Equation of a Line

• Graphing y mx b using the slope and
  y-intercept
• Plot the point containing the y-intercept on the
  y-axis. This is the point (0,b).
• Obtain a second point using the slope m. Write m
  as a fraction, and use rise over run, starting at
  the point containing the y-intercept, to plot
  this point.
• Use a straightedge to draw a line through the two
  points. Draw arrowheads at the end of the line to
  show that the line continues indefinitely in both
  directions.

9
The Slope-Intercept Form of the Equation of a Line

• Example Graph the linear function y ?x 3 by
  using the slope and y-intercept.
• Solution Since the graph is given in
  slope-intercept form we can easily find the slope
  and y-intercept.

10
The Slope-Intercept Form of the Equation of a
LineExample Continued

• Step 1. Plot the point containing the y-intercept
  on the y-axis. We plot y-intercept is (0,2).
• Step 2. Obtain a second point using
• the slope, m. The slope as a fraction
• is already given
• We plot the second point at (3,4).
• Step 3. Use a straightedge to draw a line through
  the two points.

11
The Slope-Intercept Form of the Equation of a Line

• Example Graph the linear function 2x 5y 0 by
  using the slope and y-intercept.
• Solution We put the equation in slope-intercept
  form by solving for y.

slope-intercept form
12
The Slope-Intercept Form of the Equation of a
LineExample Continued

• Next, we find the slope and y-intercept
• Start at y-intercept (0,0) and obtain a
• second point by using the slope.
• We obtain (5,-2) as the second point and
• use a straightedge to draw the line
• through these points.

13
Equations of Horizontal and Vertical Lines

• The graph of y b or f(x) b is a horizontal
  line. The y-intercept is b.

The graph of x a is a vertical line. The
x-intercept is a.
14
Equations of Horizontal and Vertical
LinesHorizontal Lines

• Example Graph y -4 in the rectangular
  coordinate system.
• Solution All ordered pairs have y-coordinates
  that are -4. Any value can be used for x.
• We graph the three ordered
• pairs in the table (-2,-4), (0,-4),
• (3,-4). Then use a straightedge
• to draw the horizontal line.

15
Equations of Horizontal and Vertical
LinesExample Continued

• The graph of y -4 or f(x) -4.

16
Equations of Horizontal and Vertical
LinesVertical Lines

• Example Graph x 2 in the rectangular
  coordinate system.
• Solution All ordered pairs have the x-coordinate
  2. Any value can be used for y. We
• graph the ordered pairs (2,-2),
• (2,0), (2,3). Drawing a line
• that passes through the three
• points gives the vertical line.

17
Equations of Horizontal and Vertical
LinesExample Continued

• The graph of x 2.

• No vertical line is a function. Why?
• All other lines are graphs of functions.

18
Slope as Rate of Change

• Slope is defined as a ratio of a change in y to a
  corresponding change in x.
• Slope can be interpreted as a rate of change in
  an applied situation.
• Example The graph shows the number
• of U.S. men and women living alone
• from 1990 through 2003. Find the
• slope of the line segment for the
• women. Describe what the slope
• represents.

19
Slope as Rate of Change

• Solution Let x represent a year and y the number
  of women living alone in that year. The two
  points shown on the line segment for women have
  the following coordinates
• Now we compute the slope.

20
Slope as Rate of ChangeExample Continued

• The slope indicates that for the period from 1990
  through 2003, the number of U.S. women living
  alone increases by approximately 0.22 million per
  year or 220,000 per year.
• Thus, the rate of change is about 220,000 women
  per year or 0.22 million women per year.

21
Modeling Data with the Slope-Intercept Form of
the Equation of a Line

• Linear functions are useful for modeling data
  that fall on or near a line.
• Example Find a function in the
• form C(x) mx b that models
• car sales, C(x), in millions, x
• years after 1990 for the graph
• given of the number of cars
• and SUVs sold by automakers.

22
Modeling Data with the Slope-Intercept Form of
the Equation of a Line

• Solution We use the line segment passing through
  the points (0,9) and (13,7.2) to obtain a model.
  We need values for m, the slope, and b, the
  y-intercept.
• Car sales, C(x), in millions, x in years after
  1990 can be modeled by the linear function
• C(x) -0.14x 9.

23
Modeling Data with the Slope-Intercept Form of
the Equation of a Line

• The slope, approximately -0.14, indicates a
  decrease in sales of about 0.14 million cars per
  year or 140,000 cars per year from 1990 through
  2003.