Euler - PowerPoint PPT Presentation
Title:
Euler
Description:
Left Riemann Sums are a Special case of Euler s method. Title: Euler s Method Author: Carol S. Schumacher Last modified by: Library and Information Services – PowerPoint PPT presentation
Number of Views:80
Avg rating:3.0/5.0
Title: Euler
1
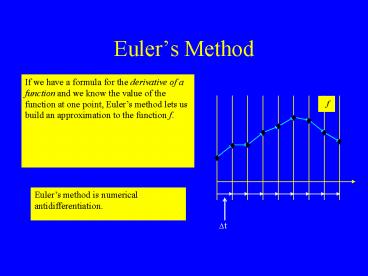
Eulers Method
If we have a formula for the derivative of a
function and we know the value of the function at
one point, Eulers method lets us build an
approximation to the function f.
Eulers method is numerical antidifferentiation.
Dt
2
Point of View
Dt
Dt
3
Total Change
The sum of the areas of these rectangles is a
left Riemann sum for the (signed) area under the
graph of f. It is also the sum of the Dys when
Eulers method finds f !
Adding the Dys to the original y0 in Eulers
method, yields the final y-value. (Why?) Thus
adding up the little changes in y gives us the
total change in f over the interval.
Moreover, the sum of the Dys in Eulers method
is an approximation to the area under the graph
of f over the entire interval
4
The sum of the Dys is a left Riemann sum
approximation to the (signed) area under the
graph of f.
The sum of the Dys in Eulers method is also an
approximation of the total change in the function
f over the interval.
This is the Fundamental Theorem of Calculus
5
Left Riemann Sums are a Special case of Eulers
method.
Suppose the formula for the derivative of y f
(t) is given in terms of t only. (E.g.
) At each stage of Eulers method, we
compute the change in y by multiplying the slope
of the function at the (left) point by Dt.
Adding these up gives us a left endpoint Riemann
sum for f over the interval.