Optics I - PowerPoint PPT Presentation
Title:
Optics I
Description:
Superposition of Light Waves Principle of Superposition: When two waves meet at a particular point in space, the resultant disturbance is simply the algebraic sum of ... – PowerPoint PPT presentation
Number of Views:53
Avg rating:3.0/5.0
Title: Optics I
1
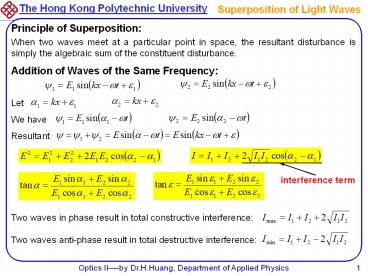
Superposition of Light Waves
Principle of Superposition When two waves meet
at a particular point in space, the resultant
disturbance is simply the algebraic sum of the
constituent disturbance. Addition of Waves of
the Same Frequency Let We have Resultant
interference term Two waves in phase result in
total constructive interference Two waves
anti-phase result in total destructive
interference
2
Superposition of Light Waves
Coherent Initial phase difference ?2-?1 is
constant. Incoherent Initial phase difference
?2-?1 varies randomly with time. Phase difference
for two waves at distance x1 and x2 from their
sources, in a medium Optical Path Difference
(OPD) n(x2-x1) Optical Thickness or Optical
Path Length (OPL) nt
3
Superposition of Light Waves
Phasor Diagram Each wave can be represented by a
vector with a magnitude equal to the amplitude of
the wave. The vector forms between the positive
x-axis an angle equal to the phase angle ?.
Suppose For multiple waves
4
Superposition of Light Waves
Example Find the resultant of adding the sine
waves Example Find, using algebraic
addition, the amplitude and phase resulting from
the addition of the two superposed waves
and
, where ?10, ?2?/2,
E18, E26, and x0.
5
Superposition of Light Waves
Example Two waves
and
are coplanar and overlap. Calculate the
resultants amplitude if E13 and E22.
Example Show that the optical path length, or
more simply the optical path, is equivalent to
the length of the path in vacuum which a beam of
light of wavelength ? would traverse in the same
time.
6
Superposition of Light Waves
Standing Wave Suppose two waves
and having the same
amplitude E0IE0R and zero initial phase angles.
nodes or nodal points
antinodes
Nodes at Antinodes at
7
Superposition of Light Waves
Addition of Waves of Different Frequency Grou
p velocity dispersion relation ??(k)
8
Superposition of Light Waves
Coherence Frequency bandwidth Coherent
time Coherent length
Example (a) How many vacuum wavelengths of
?500 nm will span space of 1 m in a vacuum? (b)
How many wavelengths span the gap when the same
gap has a 10 cm thick slab of glass (ng1.5)
inserted in it? (c) Determine the optical path
difference between the two cases. (d) Verify that
OPD/? is the difference between the answers to
(a) and (b).
9
Superposition of Light Waves
Example In the figure, two waves ?1 and ?2 both
have vacuum wavelengths of 500 nm. The waves
arise from the same source and are in phase
initially. Both waves travel an actual distance
of 1 m but ?2 passes through a glass tank with 1
cm thick walls and a 20 cm gap between the walls.
The tank is filled with water (nw1.33) and the
glass has refractive index ng1.5. Find the OPD
and the phase difference when the waves have
traveled the 1 m distance.
10
Superposition of Light Waves
Example Show that the standing wave ?s(x,t) is
periodic with time. That is, show that ?s(x,t)
?s(x,t?).
Homework 11.1 11.3 11.4 11.5 11.6