Derivatives of Perkowski - PowerPoint PPT Presentation
Title:
Derivatives of Perkowski
Description:
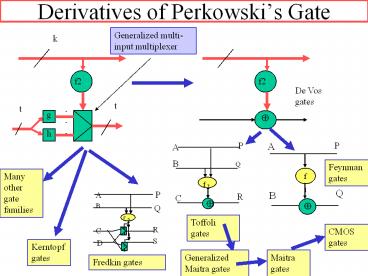
Derivatives of Perkowski s Gate Generalized multi-input multiplexer k f2 De Vos gates f2 t t... g A P h Feynman gates P A Many other gate families f 1 B Q – PowerPoint PPT presentation
Number of Views:35
Avg rating:3.0/5.0
Title: Derivatives of Perkowski
1
Derivatives of Perkowskis Gate
Generalized multi-input multiplexer
f2
De Vos gates
?
A
P
Feynman gates
Many other gate families
f 1
Q
B
P
A
?
B
Q
f 2
R
C
01
CMOS gates
S
D
Kerntopf gates
0
1
Generalized Maitra gates
Maitra gates
Fredkin gates
2
Structure of Wave Cascade
- The definitions presented in this section are
based on and , with some modifications. - Definition. A complex Maitra term is recursively
defined as follows - (1) Constant 0 (1) Boolean function is a Maitra
term. - (2) A literal is a Maitra term.
- (3) If Mi is a Maitra term, a is a literal, and G
is an arbitrary two-input Boolean function, then
Mi1 G( a, Mi1 ) is a Maitra term. - Additionally, it is required that each variable
appears in each Maitra term only once and that
the same variable ordering is used to represent
all Maitra terms. - Previous authors restricted the two-input
functions used in the Maitra terms to only
functions AND, OR, and EXOR. - For the purposes of reversible logic synthesis,
on the other hand, it is better to use the above
more general definition. - In a variation of our algorithm targeting
low-power CMOS implementation, G cannot be an
EXOR function and its complement, NEXOR.
3
Generalized Maitra Gate, Maitra Gate or CMOS Gate
Reversible Wave Cascade
4
Material for exam
- Definition of reversible functions.
- Balanced and Conservative functions. Interaction
and other optically-realizable reversible gates
that are not nn gates. - Toffoli, Feynman, Peres, Fredkin, Miller,
Margolus and Kerntopf gates. Properties. Be able
to synthesize one from another one. SWAP gates. - Convertion of irreversible circuit to reversible
quantum array. - Concepts of garbage, input constants and ancilla
bits. - Mirrors, local mirrors, spies, folding of
constants. - Generalized controlled gates (so-called
Perkowskis gates). - Transposition vector, vs set of cycles, vs Kmap
vs truth table vs BDD notations. Go from one to
another. - MMD algorithm and synthesis without ancilla bits.
- EXOR Lattice for symmetric and non-symmetric
functions. Understanding of symmetry and its
uses. - Naïve methods of converting from irreversible
netlist to reversible quantum cascade. - Billard Ball model.
- KFDD and function graphs with linear and
nonlinear preprocessors. Davio versus Shannon
expansions. - Synthesis of linear reversible circuits.
- Nets versus lattices, single-output versus
multi-output - Multiple-valued reversible logic. Fundamentals.
(no advanced methods).
5
Material for exam
- 17. PPRM, FPRM and ESOP. Synthesis and use in
reversible logic. - 18. Kronecker Products and matrix representation
of logic. - 19. QUIDDs.
- 20. Analyze a circuit with CV, CV and CNOT
gates. - 21. Analyze Mitra cascades or other complex
realizations. No synthesis. - 22. Being able to use symmetry or other
properties such as balancedness, or NPNP
classification classes in problems formulated in
natural language. - 23. Being able to combine several methods to
design a practical quantum oracle, such as a
spectral transform or arithmetic circuit. - 24. Being able to combine several methods, or at
least select one good method to analyze a
reversible circuit. Binary of multi-valued. - 25. Being able to analyze a reversible fuzzy
circuit. - 26. Being able to solve linear equations to solve
some problem in reversible logic. (1) expansions
of non-symmetric functions. (2) inverse gate. (3)
find inputs states from output states. - 27. Testability of simple cascades. EXOR cascade.
Cascade from PPRM.