Part 3. Bravais Lattice and Crystal structures - PowerPoint PPT Presentation
1 / 40
Title:
Part 3. Bravais Lattice and Crystal structures
Description:
Extending the icosahedron by adding ccp layers on each of the 20 triangular faces one kind of quasicrystal (point group symmetry 235) Stable ... – PowerPoint PPT presentation
Number of Views:652
Avg rating:3.0/5.0
Title: Part 3. Bravais Lattice and Crystal structures
1
Part 3. Bravais Lattice and Crystal
structures (From Chapter 3 and 4 of Textbook 1)
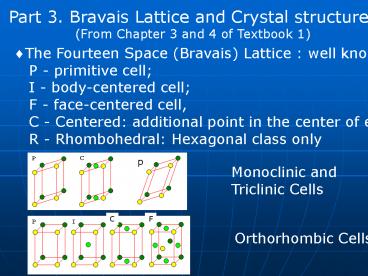
?The Fourteen Space (Bravais) Lattice well
known P - primitive cell I - body-centered
cell F - face-centered cell, C - Centered
additional point in the center of each end R -
Rhombohedral Hexagonal class only
p
Monoclinic and Triclinic Cells
F
C
Orthorhombic Cells
2
Hexagonal and Triagonal Cells
Rhombohedral
Tetragonal Cells
Cubic Cells
3
?32 crystal Classes ? In 2D, there are 10
plane point groups. In 3D, there are 32
space point group and can be divided into 7
crystal systems (link to the 14 Bravais
lattices). The symmetry operations in 2D
include translation, rotation, reflection
(mirror), glide operation. In 3D, two extra
symmetry operations are included
inversion and screw operation. Actually, these
two operations are also the combination of
rotation, reflection, and translation
operation. ? The notation used in 3D point
groups include Schönflies notation and
Hermann-Mauguin notation (major notation in
2D plane groups).
4
? Schönflies notation The Schönflies
convection was conceived primarily to
describe symmetry in optical spectroscopy and
quantum mechanics. (a) The letter O
(for octahedron) indicates that the group
has the symmetry of an octahedron, with (Oh)
or without (O) improper operation
(those that change handedness left
handed or right handed). (b) The letter T
(for tetrahedron) indicates that the group
has the symmetry of a tetrahedron. Td
includes improper operation. Th is T
with the addition of an inversion.
(c) Cn (for cyclic) indicates that the group
has an n-fold rotation axis. Cnh is
Cn with the addition of a mirror
plane perpendicular to the axis of rotation. Cnv
is Cn
5
with the addition of a mirror plane
parallel to the axis of rotation.
(d) Sn (for Spiegel, German for mirror) denotes
a group that contains only an n-fold
rotation-reflection axis. (e) Dn (for
dihedral, or two sided) indicates that the
group has an n-fold rotation axis plus a
two-fold axis perpendicular to that
axis. Dnh has, in addition, a mirror
plane perpendicular to the n-fold axis. Dnv
has, in addition to the elements of Dn,
mirror planes parallel to the n-fold
axis Due to the crystallographic
restriction theorem, n 1, 2, 3,
4, 6.
? Hermann-Mauguin notation (a) A set of
4 symbols. (b) The first one describe the
centering of the Bravais
6
lattice (P, C, I, R, or F). (c)
The next three describe the most prominent
symmetry operation visible when projected
along one of the high symmetry
directions of the crystal (the same
as used in point groups, with the addition
of glide planes and screw axis).
? Point Groups in 3D (Ref. 1 - chapter 1)
Basic symmetry operations in 3D
Rotation Reflection Inversion
7
p p p p p ---------------- b b b b b
Glide plane
In 2D, it is line
3(1/3)1
4(1/4)1
2(1/2)1
3(2/3)2
Screw Operation nT m Notation nm
n fold of rotation m number of cell required to
return to the starting position T fraction of
cell translated before reflection
8
4(2/4)2
4(3/4)3
Total screw Operations 21, 31, 32, 41, 42,
43, 61, 62, 63, 64, 65.
6(1/6)1
6(2/6)2
6(3/6)3
6(4/6)4
6(5/6)5
9
The locations of axes of Hexas 63 in the HCP
structure. Pass Through the unfilled channels.
Hexas 63
10
?Supplement ? Mathematics of symmetry
operation An objects (patterns or unit
cell, etc.) in space after some space
transformation remains the same ? symmetric
functions or symmetry operations. Assume
g is a space transformation function,
transform a coordination (x1, x2, x3) into a new
coordination (x1?, x2?, x3?), i.e. g(x1,
x2, x3) (x1?, x2?, x3?). Let F
representing the property function in
space, g will be a symmetric transformation
function if the following condition is
met.
The symmetric function g has an inverse function
g-1 which is also symmetric function itself.
11
If an object can be represented by groups
of points in it, all the new points
after symmetric transformation is
completely matched to the original ones. The
matched points before and after
transformation are called equipoints
(there will have the same properties).
The symmetric functions includes translation,
rotation, reflection, and the
combinations of the above three.
Translation, rotation, and their combinations
are proper transformation.
Transformations involving reflection are
improper transformation. Using a
coordination with orthogonal axes (unit vector
in three axes,
) (
), a transformation in space
can be represented by
12
t1, t2, and t3 representing the translational
component in x, y, and z axis, respectively
In form of matrix
Translation
unit matrix
Rotation Assume the rotation axis is x3
and rotate an angle ?
13
Reflection Assume the reflection plane
(mirror plane) is x1-x2 plane
Proper transformation detaij 1 and
improper transformation detaij -1
14
? To represent the symmetry of the rotation
group, the stereogram circle is used.
Large circle Stereogram circle. Small circle
(within) Group of atoms
Projection of axis (Top view)
Stereogram circle
Projection of a direction on ac plane
15
? Notation for asymmetric used to represent
point group symmetry (a)
Asymmetric unit in the plane of the page
(b) Asymmetric unit above the plane
of the page (c) Asymmetric unit
below the plane of the page (d)
Apostrophe indicating a left-handed
asymmetric unit. Clear circle
indicating
right-handedness. (e) Two
asymmetric units on top of each other
(f) Two asymmetric units on top of one
another, one left-handed
and the other right-handed.
?
,
?
,
,
and
are mirror images of each other.
16
? Point groups generated by the
Rotoreflection operation
? Point groups generated by the
Rotoinversion operation
17
? Point groups generated by the placement
of Mirror planes normal to the rotation
axes
/m mirror plane normal to the rotational
axis (exactly the same as Rotoreflection)
? Point groups generated by the placement
of Mirror planes parallel to the
rotation axes
Looking at Different direction
18
? Point groups generated by the use of
combined rotation axes
Solid circle above the plane Open circle below
the plane
? Point groups generated by multiple n/m
Axes with rotation
19
? Point groups combining rotoreflection and
rotation
? Animated Gif for 32 point groups
http//neon.mems.cmu.edu/degraef/pg/
? Point groups in Stereographs, 3D objects,
or molecules
http//www.phys.ncl.ac.uk/staff/njpg/symmetry/
? Point groups using simple notation
20
7 Polar Symmetries Using 3 as an example
Originate from wrapping the 1D symmetry on
a cylinder
21
Triclinic, Monoclinic and Orthorhombic Symmetries
?
?
Trigonal Symmetries
?
?
?
22
Hexagonal Symmetries
Tetragonal Symmetries
23
Cubic Symmetry
? Other good websites http//www.cryst.ehu.es/
http//cst-www.nrl.navy.mil/lattice/spcgrp/
24
(No Transcript)
25
? Equipoints a set of symmetry-related
positions. The number of points or
positions in the set is the rank of the
equipoint e.g. rank 4. An example
of equipoint is the corner site in a cell.
Rank 1, there are eight of them 000, 001, 010,
100, 110, 101, 011, 111. Each atom is
shared by eight cells, they all have
identical environment.
General Position and Special Position
the general equipoints are described
by x, y, z and special positions are
described by fixed values ranging from
completely specified (½, ½, ½) to partially
specified (x, y, ½).
Examples Plane group p4 (in the next page)
26
Condition limiting possible reflection (structure
factor)
Symmetry of the equipoints
rank
designation
27
0, 0
0, 1
0, 0
0, 1
y, 1-x
0, 1/2
x, y
01/2, 1
1/2, 0
1, 0
1, 0
1-x, 1-y
1, 1/2
1-y, x
4?1/2
2 c 2
4 d 1
0, 0
0, 1
0, 0
0, 1
1/2, 1/2
1, 0
1, 0
1, 1
1 b 4
1 a 4
28
Rotation Reflection Inversion
Translation
32 point groups
14 Space Lattices
Screw Glide
230 Space Groups
29
? 230 space group
http//www.mkmc.dk/crystal/group.html ?
Example for International Tables for
Crystallography http//www.iucr.org/iucr
-top/it/general/itt.pdf
?Use of the international tables for
crystallography ? First example Space group
No. 139 I4/mmm, (D174h) (1) Left short
Hermann-Mauguin symbol left-center
Schoenflies symbol right-center Crystal class
right crystal system (2) Left
space group number left-center Full Hermann
-Mauguin symbol right Patterson
symmetry (not discussed) (3)
Space group diagram (4) The symmetry of the
site chosen as cell origin
30
(5) and (6) are the symmetry of the site
chosen as cell origin to generate the cell.
31
(No Transcript)
32
?Quasiperiodic crystals or crystalloids ?
Real crystal are finite ? atoms on the surface
have different environment from those
inside. Crystals nucleate and grow not
according to geometrical rules (energy
consideration) but according to the local
requirements of atomic or molecular packing
(kinetics), chemical bonding ?
non-periodic arrangement is possible ?
called quasiperiodic crystals,
quasicrystals, or crystalloids.
Discovered by Shechtman in 1982.
Decagonal (10-sided) prism Al-Ni-Co
Dodecahedron Al-Cu-Fe
Photographs of An Pang Tsai, NRIM, Tsukuba, Japan
33
Zn6Mg3Ho icosahedral quasicrystal.
34
? The close packing of 12 spheres
(cubeoctahedron) around a center atoms ?
eliminating the uneven packing of the above
structure by shifting the 12 atoms to
obtain even distribution ? if the center atom is
small brought together the 12 atoms ? 20
triangular faces of an icosahedron. See
Fig. 4.14. Extending the icosahedron by
adding ccp layers on each of the 20
triangular faces ? one kind of quasicrystal
(point group symmetry 235) Stable
structure, characteristic of many virus
structures, make them so indestructible.
35
Point group crystal system
Space group
Projection in the x-y plane
Origin is on diad axis (it can Be on )
diad
Glide planes
Equipoints
36
(No Transcript)
37
(No Transcript)
38
(No Transcript)
39
(No Transcript)
40
(No Transcript)