Syntax, Semantics and Intended Meaning The 1-slide course

1
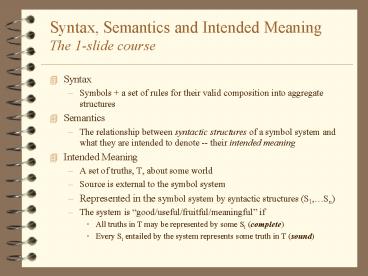
Syntax, Semantics and Intended MeaningThe
1-slide course

• Syntax
• Symbols a set of rules for their valid
  composition into aggregate structures
• Semantics
• The relationship between syntactic structures of
  a symbol system and what they are intended to
  denote -- their intended meaning
• Intended Meaning
• A set of truths, T, about some world
• Source is external to the symbol system
• Represented in the symbol system by syntactic
  structures (S1,Sn)
• The system is good/useful/fruitful/meaningful
  if
• All truths in T may be represented by some Si
  (complete)
• Every Si entailed by the system represents some
  truth in T (sound)

2
LP Syntactic Structures

• Terms
• Constant, Variable, Functor, Term, Compound Term
• Instances
• Substitution, Instance
• Facts and Queries
• Facts and the rule of instantiation
• Queries and the rule of generalization
• Rules and Logic Programs
• Rules, Unification, Logic Programs

3
TermThe single data structure

• Constant
• letter, number, string of letters or numbers
  beginning with a lower case letter.
• Intended to represent entities, individuals,
  existent etc. dog, cat, alfred, r2d2, selmer,
  the number 2.
• Variable
• Capital letter, or string of letters or numbers
  beginning with a capital letter
• Intended to represent an yet to be
  identified/deduced constant.
• Operationally Write Once, Ready Many. Once
  found truth can not be unfound.
• Compound term
• Functor(A1,,An)
• Functor is any constant
• Ai is a term.
• Intended to represent relations or predicates
  about things father(selmer,r2d2).

4
SubstitutionA formal definition

• A finite set of pairs of the form xi tj, where
• xi is a variable
• ti is a term
• xi lt gt xj for every i lt gt j
• variables are bound only once in the substitution
  
• xi does not occur in tj, for any i and j.
• no recursive references in a binding
• Substitutions can be applied to terms
• foo(X,Y) Xselmer,Yr2d2 ? foo(selmer, r2d2).

5
InstancesA relationship between terms

• One term may be an instance of another term.
• A is an instance of B if
• There exists a substitution q such that ABq
• Think of a substitution as a set of variable
  bindings
• Examples
• 1 is an instance of X.
• The substitution is X1
• foo(bar,1) is an instance of foo(V,1)
• the substitution is VBar
• father(big_Tony,franky_three_shots) is an
  instance of father(X,Y)
• What is the substitution?
• A ground instance is an instance with NO
  variables
• foo(V,Y)Vbar) foo(bar,Y) is NOT ground.
• foo(V,Y)Vbar, Y1) foo(bar,1) is ground.

6
Queries

• A compound term preceded by -
• - functor(t1,,tn)
• Variables are existentially quantified
• Example
• LP - father(big_Tony,X)
• FOL ?x father(big_Tony, x)
• English
• Is Big Tony anyone's father?
• Does there exist a thing, X, such that Big Tony
  is the father of X?

7
Rule of Generalization

• An existential query P is a logical consequent of
  an instance of P, Pq, for any substitution q.
• Example
• - father(big_Tony, X) is a logical consequent of
  
• father(big_Tony, two_Ton_Tommy)
• Different ways of saying the same thing
• ?X p(X) is true if p(anything here) is true.
• The existential generalization is true if an
  instance of it is true.

8
A Fact

• Compound Term
• Functor(t1,,tn)
• Variables are universally quantified
• father(big_Tony, X) means
• English big Tony is everyones father
• FOL ?(X) father(big_Tony,X)

9
Rule of Instantiation

• From a universally quantified statement P (i.e.,
  a fact) deduce an instance of it, Pq, for any
  substitution q.
• From father(big_Tony,X) deduce
• father(big_Tony, two_Ton_Tommy),
• father(big_Tony, selmer),
• father(big_Tony, franky_Three_shots),
• father(big_Tony, r2d2)

10
A Rule

• Complex query composed of simpler queries
• Has a head (a term) and a body (a sequence of
  terms)
• H ? B1,,Bn
• A fact is a rule where n0
• Example
• LP grandmother(X,Z) ? mother(X,Y), mother(Y,Z).
• FOL ?(X,Y,Z) mother(X,Y) mother(Y,Z) ?
  grandmother(X,Z)
• English A person who is the mother of another
  person who is herself the mother of a third
  person is the grandmother of the third person
• Which representation do you prefer?

11
Rule of Universal Modus Ponens

• From
• A ? B1,,Bn ? (B1,,Bn)
• where for all i between 1 and n, Bi is an
  instance of Bi
• A is an instance of A
• Deduce
• A
• From
• grandfather(X,Z) ? father(X,Y), father(Y,Z)
• father(big_Tony, little_Tony), father(little_Tony,
  tiny_Tony)
• Deduce
• grandfather(big_Tony, tiny_Tony)

12
A Logic Program

• A logic program is a finite set of rules.
• Example Logic Program The Family Tree
• father(big_Tony, little_Tony).
• father(little_Tony, tiny_Tony).
• boss(quick_Nick, franky_three_shots).
• boss(buddy_the_button, mikey_the_mop).
• boss(big_tony,Y) ? boss(Y,Z).
• grandfather(X,Z) ? father(X,Y), father(Y,Z).
• Example Queries
• - boss(big_Tony, quick_Nick)
• yes
• - boss(big_Tony, r2d2)
• no.why? Whats the intended meaning of the
  boss(big_tony_Y) rule above?

Facts are rules where the body is empty.
Rules with bodies
13
More on Semantics

• Declarative and Operational

14
Declarative SemanticsWhat it means is what it is.

• How a the syntactic structure of a program
  relates to its intended meaning
• The meaning of a logic program is the set of all
  the statements it entails
• The meaning of a logic program P is the minimal
  model for P

• where the minimal model for P is the intersection
  of all models of P,
• where a model of P is an interpretation, I, for P
  where, for every ground instance of a clause A ?
  B in P, A is in I if B is in I
• where an interpretation is a subset of the
  Herbrand Base
• where the Herbrand Base is the set of all ground
  goals that can be formed (syntactically) form the
  predicates in P and the terms in the Herbrand
  Universe
• where the Herbrand Universe is the set of all
  ground terms that can be formed from the
  constants and function symbols appearing in P.

15
Operational SemanticsWhat it means is what a
procedure produces.

• The meaning of a logic program, P, is the set of
  ground goals that are instances of queries
  produced by P according to a LP computation.
• A computation of a program P is a procedure.
• The procedure starts with a query G, progresses
  via goal reduction and it either succeeds, fails
  or never terminates.
• If it succeeds then the instance of G produced is
  in the meaning of P.
• If it fails then no instance of G is in the
  meaning of P.
• If it never terminates, then we dont know if any
  instance of G is in the meaning of P.
• How does goal reduction produces statements
  (i.e., answers)?
• How it works is the operational semantics of
  logic programming.
• The meaning is the output of the procedure. The
  operational semantics is a formal description of
  the procedure for producing the output from the
  input.

16
Classic Programming Languages

• What are the declarative semantics?
• Scott-Strachey approach to programming language
  theory
• Denotational Semantics (book by Joseph Stoy)
• Lambda Calculus (dates back to Lisp in the
  1950s)
• Concept Given a program, P and the environment,
  E (contents of the registers of the machine or of
  variable/value bindings), there is a formal
  theory for logically deducing the next
  environment -- the environment that is entailed
  by P E.
• What are the operational semantics?
• How the compiler processes the program to
  determine the assignment of variables to values.

17
Goal Reduction

• The computational core of LP

18
Elements of Goal Reduction

• Unification Pattern Matching
• Resolution Inference
• Chronological Backtracking Search

19
Unification

• A constructive pattern matching procedure
• Determines the most general unifier (MGU) of two
  terms.
• Using the MGU constructs the most general common
  instance of two terms
• Used to compute the resolution inference
• Resolution is used to iteratively reduce a goal
  generating its proof from the program if possible
  (i.e., goal reduction)

20
Most General Unifier(MGU)

• A common instance of A and B is an instance of
  both A and B.
• foo(1,2) is a common instance of foo(1,Y) and of
  foo(X,2)
• A unifier of two terms is a substitution that
  renders the terms identical
• X1,Y2 applied to foo(1,Y) and to foo(X,2)
  produces identical terms
• The MGU of two terms is a unifier that produces
  the most general common instance of the two
  terms.
• Which are instances of both foo(X,Y), foo(1,Y)?
  What is the MGU?
• bar(1, 2)
• foo(A, 1)
• foo(A, B)
• foo(1, 2)
• foo(1, B)

None, None XA, Y1, None XA, YB,
None X1,Y2, Y2 X1,YB, YB
21
Unification Procedure

• Computes the MGU of two terms if it exists else
  fails.
• unify(X,1) X,1
• unify(a(X,1), a(1,Y)) X1, Y1)
• Produces the most general common instance
• If two terms unify it is NOT necessarily the case
  that one is an instance of the other
• a(X,1) is not an instance of a(1,Y)
• a(1,Y) is not an instance of a(X,1)

22
Unification Examples

• Fact Schema Supports(Video_Card, Display_Type,
  Number_of_Colors)
• Example 1 Neither A nor B entails the other
• Facts (A and B) supports(V,b14,C),
  supports(nvidia,D,256)
• MCI supports(nvidia, b14, 256)
• MGU Vnvidia, Db14, C256)
• Example 2 B is an instance of A
• Facts (A and B) supports(V,b14,C),
  supports(rage,b14,16)
• MCI supports(rage, b14, 16)
• MGU Vrage, C16
• Example 3 A and B are not unifiable
• Facts (A and B) supports(V,b14,16),
  supports(nvidia,D,256)
• MCI None
• MGU None

23
Resolution

• Enables a reduction of two rules into one
• Eliminates a common term from the consequent of
  one rule and from the antecedent of another
• Efficient application depends on the constructive
  unification procedure
• General Form
• Rule 1 A ? (B M)
• Rule 2 (C N) ? D
• Resolution Inference (A C)q ? (B D) q
• where q is the MGU of M and N.

24
Resolution Example

• Rule 1
• affinity(Y,Z) - likes(Y,Z), even(Z).
• Rule 2
• basic_affinity(Y) - affinity(Y,2)
• Inference
• basic_affinity(Y) - likes(Y,2), even(2).
• The inference allowed the
• elimination of affinity(Y,Z) and
• reduction to a single rule through finding
• the MGU of affinity(Y,Z) and affinity(Y,2) and
• applying it to the body of rule 1.
• What fact would make - basic_affinity(r2d2) true?

25
Chronological Backtracking

• A search procedure for finding unifying terms
• Conducted over an explicit set of clauses
• Upon failure (a dead-end) previous variable
  bindings are chronologically unwound and the
  search begins again until all possible bindings
  are exhausted
• Note
• Fruitful bindings may be unrelated to chronology
• Can therefore be terrible inefficient
• Must be aware of how this works to write
  efficient LPs

26
Backtracking Example

• - plugsIn(X,Y)
• outPort(o5)
• inPort(in1)
• compatible(o5,in1)
• FAIL and Backtrack
• inPort(in2)
• compatible(o5,in2)
• FAIL and Backtrack
• inPort(in3)
• compatible(o5,in3)
• Success(1). plugsIn(o5,in3).
• inPort(Y)
• FAIL and Backtrack
• outPort(X)
• FAIL

• plugsIn(X,Y) -
• outPort(X),
• inPort(Y),
• compatible(Y,Y).
• inPort(in1).
• inPort(in2).
• inport(in3).
• outPort(o5).
• compatible(o5,in3).

Search order matters. Put compatible first in the
plugsIn rule?
27
Practical Introduction to Prolog

• Basics

28
Programming Excursions

• Exploring Unification
• Basic Constructs
• Arithmetic, Recursion and Lists
• Database Programming
• Trees and Parsing

29
Practical Introduction to Prolog

• Negation and the Cut