SP4 Quantum Simulation and Control

1
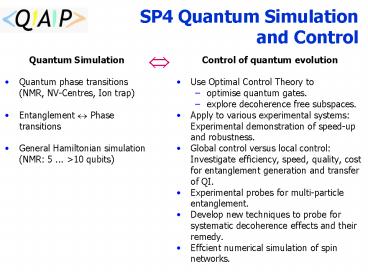
SP4 Quantum Simulation and Control
?

• Quantum Simulation
• Quantum phase transitions (NMR, NV-Centres, Ion
  trap)
• Entanglement ? Phase transitions
• General Hamiltonian simulation (NMR 5 ... gt10
  qubits)

• Control of quantum evolution
• Use Optimal Control Theory to
• optimise quantum gates.
• explore decoherence free subspaces.
• Apply to various experimental systems
  Experimental demonstration of speed-up and
  robustness.
• Global control versus local control Investigate
  efficiency, speed, quality, cost for entanglement
  generation and transfer of QI.
• Experimental probes for multi-particle
  entanglement.
• Develop new techniques to probe for systematic
  decoherence effects and their remedy.
• Effcient numerical simulation of spin networks.

2
Quantum Simulation and ControlWP 4.1 Rare Earth
Ion-Doped Crystals, S. Kröll, Lund
Pr doped yttrium silicate crystal

• Hyperfine qubits
• Dipole interaction for cond. dynamics

3
Quantum Simulation and ControlWP 4.1, Rare Earth
Ion-Doped Crystals, S. Kröll, Lund

• Objectives within QAP (1st year)
• Develop laser light source for coherent qubit
  operation.
• Test single ion readout.

4
Quantum Simulation and ControlWP 4.1, Rare Earth
Ion-Doped Crystals, S. Kröll, Lund

• Achievements
• Laser source coherence time of 100 ?s (initially
  projected 10 ?s).

Free induction decay Beat signal between laser
and Pr ions (PrYSO)
5
Quantum Simulation and ControlWP 4.1, Rare Earth
Ion-Doped Crystals, S. Kröll, Lund

• Achievements
• Laser source coherence time of 100 ?s (initially
  projected 10 ?s).

Free induction decay Beat signal between laser
and Pr ions
6
Quantum Simulation and ControlWP 4.1, Rare Earth
Ion-Doped Crystals, S. Kröll, Lund

• Achievements
• Laser source coherence time of 100 ?s (initially
  projected 10 ?s).

Laser phase drift lt5? over 10 ms Coherence time
gt 100 ms
7
Quantum Simulation and ControlWP 4.1, Rare Earth
Ion-Doped Crystals, S. Kröll, Lund

• Achievements
• Laser source coherence time of 100 ?s (initially
  projected 10 ?s).

Laser frequency drift ? 0.3 kHz/sec
8
Quantum Simulation and ControlWP 4.1, Rare Earth
Ion-Doped Crystals, S. Kröll, Lund

• Milestones
• 4.1.1 Agreement with TU Munich on what pulses to
  test for qubit operations
• Delayed due to laser development
• 4.1.2 Fluorescence detection of qubits
• Coherent readout technique preferredexcellent
  signal-to-noise, detection limit needs to be
  determined.
• Deliverables
• 4.1.1 Two-qubit operations tested with pulses
  derived using optimal control theory
• Delayed due to laser development
• Status and Outlook Delays, however no show
  stoppers in sight.
• All exp. parts developed and tested perform as
  anticipated or better.Single ion readout
  Scalable QC scheme (quant-ph/0601141).
• Single ion readout ion is under test.

9
Quantum Simulation and ControlWP 4.2, NV Defects
in Diamond, J. Wrachtrup, Stuttgart
Use single electron spin as read-out for
nuclear/electron spin cluster Use nuclear spins
for simulations
T. Gaebel et al., Nature Physics 2, 408 (2006)
10
Quantum Simulation and ControlWP 4.2, NV Defects
in Diamond, J. Wrachtrup, Stuttgart
readout
polarization
Initialization and readout (optical)
echo (t1t2)
Manipulation (microwaves)
t2
t1
time
T. Gaebel et al., Nature Physics 2, 408 (2006)
11
Quantum Simulation and ControlWP 4.2, NV Defects
in Diamond, J. Wrachtrup, Stuttgart

• Coherent coupling to 4 different nuclei is
  demonstrated.
• To come coherent control in 3 spin system

Hyperfine coupling to 13C and 15N
13C
A
B
15N
L. Childress et al., Sciencexpress, Sept. 2006
12
Quantum Simulation and ControlWP 4.2, NV Defects
in Diamond, J. Wrachtrup, Stuttgart

• Milestones
• 4.2.1 Generation of defect centre pairs with
  magnetic dipole interaction of 10 MHz and
  dephasing times larger than 0.1 ms.
• Strong magnetic coupling gt 10 MHz of NV-N . Phase
  memory 0.35 ms (Nature Physics 2, 408, 2006).
• 4.2.2 Observation of ground state spin coherence
• Achieved.
• Deliverables
• 4.2.1 Creation of defect centre pairs with
  distances less than 10 nm.
• Pairs with ? 3 nm have been implanted
• 4.2.2 Observation of ground state spin
  entanglement
• Entanglement between two electron and a single
  nuclear spin.
• Status and Outlook Coherent coupling to 4
  different nuclei demonstrated.Two and three-spin
  systems will be investigated Determine phase
  memory. Entangle single electron spin with single
  nuclear spin.

13
Quantum Simulation and ControlQuantum
Compilation a Control Problem
14
Quantum Simulation and ControlWP 4.3, Optimal
Control of Qu. Systems in Finite Dim.

• Milestones
• 4.3.1 Numerical simulation and optimal control of
  superconducting devices.
• Capacitively coupled superconducting Josephson
  charge qubits Q. optimal control provides
  shaped pulses that reduce the error rate of CNOT
  and TOFFOLI by two orders of magnitude with
  5-fold speed-up (quant-ph/0504202).
• 4.3.2 Numerical simulation and optimal control of
  quantum gates in ion traps.
• Single qubit gates implemented with trapped ions
  (see WP 4.6)
• Deliverables
• 4.3.1 Computer programmes tailored to
  experimental techniques other than NMR
• MATLAB interface to optimal-control based GRAPE
  algorithm can be adapted to experimental settings
  of QAP partners.
• Outlook Adapt optimisation tools to other
  experimental techniques
• Extend optimal control to cond. dynamics, e.g.,
  with ion traps.

15
Quantum Simulation and ControlWP 4.4, Modelling
QC with 5 and more than 10 Qubits

• Milestones
• 4.4.1 Numerical simulations on spin systems with
  5 qubits.
• 4.4.2 Test on spin systems with 5 qubits and
  numerical simulations for 10 qubits.
• Experimental tests up to 5 NMR spin qubits are
  successful.
• Parallelised optimal-control-based GRAPE
  algorithm with speed-up gt500 fold for 10 qubits
  on cluster of 128 CPUs compared to 1 CPU on same
  cluster (Proceedings EUROPAR 2006, LNCS 4128,
  751, 2006).
• Deliverables
• 4.4.1 Restricted test bed for quantum
  computational control on few-qubit systems.
  Extension of hardware beyond 10 qubits.
• The synthetic work for NMR spin-system hardware
  beyond 10 qubits has faced unexpected chemical
  difficulties.
• Status and Outlook Future improvements beyond
  the goals of QAP will depend on progress in
  computer science.

16
Quantum Simulation and ControlWP 4.5,
Hamiltonian Simulation and DFS

• Treated logical qubits embedded in a larger
  Liouville space of physical qubits by optimal
  control theory tailored to open dissipative
  systems.
• Extended gradient-flow algorithm (GRAPE) to
  superoperators such as to find best
  approximations to a unitary target gate in the
  presence of dissipation (quant-ph/0609037).
• Milestones
• 4.5.1 Establish limits on controllability in 2
  and 3 qubits for ZZ, XY and XYZ coupling.
• Controllability investigated up to systems of 4
  physical qubits, e.g. in the KANE setting of
  nucleus-electron-electron-nucleus.
• 4.5.2 Establish limits on controllability in 3
  qubits under Redfield-type relaxation.
• Optimal control algorithms developed to give best
  approximations to unitary target modules in open
  systems.

17
Quantum Simulation and ControlWP 4.5,
Hamiltonian Simulation and DFS

• CNOT with dissipation
• optimal control trace fidelity gt 95
  traditional methods, Trotter lt 15

• Complete classification of locally reversible
  interaction Hamiltonians

18
Quantum Simulation and ControlWP 4.6 Trapped Ion
Spin Molecule, Chr. Wunderlich, Siegen

• Qubits Hyperfine states
• Conditional quantum dynamics

• Combine advantageous features of two
  experimental wolrds NMR and Trapped Ions.

19
Quantum Simulation and ControlWP 4.6 Trapped Ion
Spin Molecule, Chr. Wunderlich, Siegen

• Milestones
• 4.6.1 Ion Trap designed and built
• Partially
• 4.6.2 Magnetic field generating elements designed
  and built.
• Partially.
• Deliverables
• 4.6.1 A new ion trap with magnetic field elements
  ready.
• Likely at the end of true month 12.
• Status and Outlook Progress roughly according
  to schedule
• Progress far ahead of schedule.

20
Quantum Simulation and ControlWP 4.6 Trapped Ion
Spin Molecule, Chr. Wunderlich, Siegen
Achievements

• Isotope selective, nearly deterministic loading
  of ion trap.

21
Quantum Simulation and ControlWP 4.7
Entanglement Generation/Propagation, Phase
Transitions V. Buzek, J. Eisert, S. Huelga, J.I.
Latorre, M. Plenio

• Understand the static and dynamic entanglement
  properties of quantum many body systems.

• Transfer of QI Long distance photons.
  Short distance e.g., condensed matter systems.
• transfer speed, quality ? spectral gap between
  ground and excited states
• ? use as q. channel or as probe for spectral
  properties.
• M. Hartmann, M. Reuter and M.B. Plenio, New J.
  Phys. 8, 94 (2006)

22
Quantum Simulation and ControlWP 4.7
Entanglement Generation/Propagation, Phase
Transitions V. Buzek, J. Eisert, S. Huelga, J.I.
Latorre, M. Plenio

• Understand the static and dynamic entanglement
  properties of quantum many body systems.

• Using only global control on a Ising-coupled
  spin chain
• Protocol for perfect transport of an unknown
  quantum state
• Protocol for perfect quantum mirroring of the
  state of the chain about its middle.
• Add local control to ends of chain execute
  universal quantum computing on spins encoded onto
  the chain.J. Fitzsimons J. Twamley, PRL 97,
  090502 (2006)

1 qubit mirror
Demonstrated in NMR J. Fitzsimons et al
quant-ph/0606188
2 qubit gate
23
Quantum Simulation and ControlWP 4.7
Entanglement Generation/Propagation, Phase
Transitions V. Buzek, J. Eisert, S. Huelga, J.I.
Latorre, M. Plenio

• Understand the static and dynamic entanglement
  properties of quantum many body systems.

• Scaling laws for entanglement in general
  harmonic lattice system, andclassical
  correlations in a classical harmonic system.
• M. Cramer, J. Eisert, M.B. Plenio and J. Dreißig,
  Phys. Rev. A 73, 012309 (2006)

24
Quantum Simulation and ControlWP 4.7
Entanglement Generation/Propagation, Phase
Transitions V. Buzek, J. Eisert, S. Huelga, J.I.
Latorre, M. Plenio

• Understand the static and dynamic entanglement
  properties of quantum many body systems.

• Energy gap between ground and first excited
  state ? decay of correlation functions in
  harmonic lattice systems on general graphs. ?
  exponential decay of correlations for ground
  state and thermal states. M. Cramer and J.
  Eisert, New J. Phys. 8, 71 (2006)

25
Quantum Simulation and ControlWP 4.7
Entanglement Generation/Propagation, Phase
Transitions V. Buzek, J. Eisert, S. Huelga, J.I.
Latorre, M. Plenio

• Understand the static and dynamic entanglement
  properties of quantum many body systems.

• Investigate dynamics of weakly driven chains of
  spin systems ? quantum correlations in steady
  state when the noise strength exceeds threshold.
  Stochastic resonance. S. Huelga, M.
  Plenio,quant-ph/0608164

26
Quantum Simulation and Control WP 4.7
Entanglement Generation/Propagation, Phase
Transitions V. Buzek, J. Eisert, S. Huelga, J.I.
Latorre, M. Plenio

• Understand the static and dynamic entanglement
  properties of quantum many body systems.

• Entanglement transfer from two modes onto two
  atoms via local Jaynes-Cummings model
• Consider relation between entanglement,
  mixedness and energyMcHugh, Ziman, Buzek, PRA
  74, 042303 (2006)

• Dependence on initial value of entanglement and
  initial energy of the photon field

entanglement between atoms
initial energy
initial entanglement
27
Quantum Simulation and ControlWP 4.7
Entanglement Generation/Propagation, Phase
Transitions V. Buzek, J. Eisert, S. Huelga, J.I.
Latorre, M. Plenio

• Understand the static and dynamic entanglement
  properties of quantum many body systems.

Deliverable 4.7.1. Simulation of a quantum
algorithm on large quantum many body systems
with up to 100 qubits. J.I Latorre
28
Quantum Simulation and ControlWP 4.8 Protecting
Quantum Memories

• Laser cooling scheme for trapped ions based on
  the dynamical Stark shift gate.
• ? Fast cooling to low final temperatures.A.
  Retzker and M.B. Plenio, quant-ph/0607199

29
Quantum Simulation and ControlWP 4.9 Simulating
q.phase transitions in ion traps, circuit QED,
and optical lattices, V. Buzek, G. Milburn

• Milestones
• 4.9.1 Specification of ion-trap models that can
  simulate quantum phase transitions.
• Jahn-Teller like quantum phase transition with a
  single trapped ion, subject to a periodic
  impulsive force (Milburn et al., submitted).
• Polaritons in array of cavities (photonic crystal
  or coupled micro-cavities) strongly interacting
  many body system, has potential as a quantum
  simulator (Imperial).
• 4.9.2 Specify experimental scheme for
  demonstrating a Jahn-Teller quantum phase
  transition in a circuit QED and ion trap.
• Capacitive coupling of mechanical oscillator to a
  superconducting circuit (Milburn et al.,
  submitted).
• Deliverables
• 4.4.1 Develop ion trap schemes as analogue
  devices for obtaining information on the
  multipartite entanglement in the ground state of
  systems that undergo quantum phase transitions.

30
Quantum Simulation and ControlWP 4.10 Q.State
and Process Estimation, V. Buzek, S. Glaser,
J.Twamley, J. Wrachtrup,

• Problem programmability of quantum devices in
  performance of quantum operations (CP maps), or
  quantum measurements (POVM).
• Programs encoded in states of quantum system
  (program register)
• Questions universality, optimality, efficiency
  of deterministic, probabilistic and approximative
  devices.
• Existence of universal programmable unambigous
  quantum state discriminator Bergou, Bužek,
  Feldman, Herzog, Hillery, Phys.Rev.A 73, 062334
  (2006),
• Buzek, Hillery, Ziman, and Rosko, Quantum
  Information Processing 5, 313-420 (2006)

31
Quantum Simulation and ControlWP 4.10 Q.State
and Process Estimation, V. Buzek, S. Glaser,
J.Twamley, J. Wrachtrup,
Work shop on Q. Process EstimationBudemerice,
Slovakia, 28 Sept 1. Oct. 2006
32
Quantum Simulation and Control
NMR
Ion Trap
Ion doped Crystal
Theory