Start with a unit length - PowerPoint PPT Presentation
1 / 26
Title:
Start with a unit length
Description:
Department of Mathematics. Start with a unit length ... as a true work of genius, laying the foundations of modern pure mathematics ... – PowerPoint PPT presentation
Number of Views:87
Avg rating:3.0/5.0
Title: Start with a unit length
1
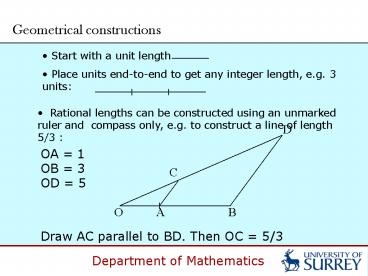
Geometrical constructions
- Start with a unit length
- Place units end-to-end to get any integer
length, e.g. 3 units
- Rational lengths can be constructed using an
unmarked ruler and compass only, e.g. to
construct a line of length 5/3
D
OA 1 OB 3 OD 5
C
O A B
Draw AC parallel to BD. Then OC 5/3
2
Geometrical constructions
- AB x, BC 1
- How long is BD?
- D
- A x
B 1 C
By similar triangles,
BD2 x BD ? x
3
Solving equations
x 2 4 can be solved for x in the natural
numbers x 4 2 can be solved for x in the
integers 2x 1 4 can be solved for x in the
rational numbers x2 2 can be solved for x in
the real numbers x2 2 0 can be solved for x
in the complex numbers The solutions of all but
the last of these equations can be shown as
points on a line, the real number line
-7 -6 -5 -4 -3
-2 -1 0 1 2
3 4 5 6 7
4
Duplicating the cube
Now consider the equation x3 2 x is the side of
a cube whose volume is 2 cubic units 1 x
From classical times, people tried to construct
the cube root of 2 by straight-edge and compass
only (duplicating the cube) This was finally
proved impossible in the 19th century
5
Quadratic equations
- Classical Greek mathematicians could solve
quadratics, but there was no algebraic
formulation until about 100 AD - They didnt believe in negative numbers, so x2
ax b was a different type of equation from x2
ax b - Solutions were geometrical, e.g. to solve x2
2ax b - ?b
- a x
6
Quadratic equations
- As we do believe in negative numbers, we just
need to solve x2 2bx c 0 - Complete the square (x b)2 b2 c 0
- (x b)2 b2 c
- x b ?(b2 c)
- x b ?(b2 c)
- We have solved the quadratic by radicals
- Can higher-order equations be solved by radicals?
7
Cubic equations
- ax3 bx2 cx d 0
- Some cubics are easy to solve. x3 x 0 has
roots - 1, 0, 1 (where the graph cuts the x-axis)
- A cubic equation can have up to three distinct
real roots - We can find them approximately. A computer
algebra program will solve x3 x2 2x 1 0
to give - x 1.801937736, x 0.4450418679, x
1.246979604 - This gives no insight into where the solutions
come from
8
Reducing a cubic
- To solve the general quadratic we completed the
square - Perhaps completing the cube will help with the
cubic - Solve x3 12x2 42x 49 0 ()
- Note that (x 4)3 x3 12x2 48x 64
- so equation () is (x 4)3 6x 15 0
- (x 4)3 6(x 4) 9 0
- y3 6y 9 0, where y x 4
- In this way we can always get rid of the
squared term
9
Getting over the next hurdle
- Now we need to solve y3 6y 9 0 ()
- Suppose we cant spot a solution by inspection
- Split y into two parts write y u v, so
- y3 (u v)3 u3 3u2v 3uv2 v3
- Equation () is u3 v3 9 3(u2v uv2 2u
2v) 0 - Solve these two equations for u and v
u3 v3 9 0, u2v uv2 2u
2v 0 - This still involves cubes, so is it any easier?
10
We can solve cubics!
u2v uv2 2u 2v 0 gives (u v)(uv 2)
0, so v u or v 2/u v u is not
consistent with u3 v3 9 0, so v 2/u u3
v3 9 0 then gives
Multiply through by u3 to get u6 9u3 8
0 (u3 1)(u3 8) 0 u3 1 or u3 8, so u
1 or u 2 y u 2/u, so y 3, so x 7
11
That wasnt too bad ...
- We can easily check that this is correct, but is
it complete? - Equation () has only one real root, which we
have found - Lets try another one solve x3 3x2 12x 18
0 - Substitute x y 1 to get y3 15y 4 0
- Put y u 5/u to get u6 4u3 125 0
- Put z u3 so z2 4z 125
0 - (z 2)2 121 0 z 2 ?(121) 2 11i
12
Continuing with the cubic
z 2 11i where z u3 Let u a bi , so (a
bi)3 2 11i Now (2 i)3 2 11i and
(2 i)3 2 11i Also (2 i) (2 i) 5 y
u 5/u so y (2 i) (2 i) 4 Finally x
y 1, so x 3 To get a real solution we had to
go via complex numbers. What about the other two
real roots?
13
The cubic formula
- Niccolo Fontana discovered this formula for
solving x3 px q where p and q are
positive
- This formula does not seem to find three
solutions, even when its clear that three exist - The quadratic formula works because 1 has two
square roots, 1 and 1 - So perhaps 1 should have three cube roots! What
are they?
14
From algebra to geometry and back
- Going from 1 to i (multiplying by i once)
corresponds to a 90º rotation about (0, 0) - Going from 1 to 1 (multiplying by i twice)
corresponds to a 180º rotation about (0, 0) - Two successive 180º rotations about (0, 0) take
us from 1 to 1, corresponding to the fact that
(1)2 1 - If multiplying by something three times in
succession takes us from 1 to 1, that thing is a
cube root of 1 - Going from 1 to 1 involves a 360º rotation about
(0, 0). One third of this is a 120º rotation
15
The cube roots of 1
The point 1/3 of the way round the unit circle
from 1 to 1 is
1 60o
As a complex number this is
16
There are three cube roots of 1
We call this complex number ? (omega) ?
?
It is easy to show that ?3 1 Also (?2)3 1
and ?2
The three cube roots of 1 are 1, ? and ?2
17
Completing the first example
Solving x3 12x2 42x 49 0, we used the
substitutions x y 4 and y u 2/u, and
found u3 1 It now follows that u 1, ? or
?2 so y 3, y ? 2?2 or y ?2 2? Thus x
7, x or x
It IS possible to solve cubics by radicals, but
its not always quite so easy!
18
Why stop there?
- What about quartic equations?
- Theyre not too bad. By a suitable substitution
we can always get rid of the cubed term - Example solve x4 2x2 8x 8 0
- Suppose it has two quadratic factors
- (x2 kx m)(x2 kx n) 0
- Expand and compare coefficients to find k, m, n
- (x2 ?2 x 2?2)(x2 ?2x 2?2) 0
- Solve two quadratics to get the four roots
19
Again, why stop there?
- Methods for solving quadratic, cubic and quartic
equations by radicals were known by the 17th
century AD - By radicals means starting with the
coefficients and using only addition,
subtraction, multiplication, division and taking
roots (square roots, cube roots, etc), including
complex roots - Quintic (fifth degree) equations resisted all
attempts to solve them by radicals - In 1824 the Norwegian mathematician Niels Abel
proved that there is no general formula for
solving a quintic by radicals - However, clearly SOME quintics are solvable by
radicals, e.g. the solutions of x5 1 are just
the five 5th roots of 1
20
Look for patterns!
- To solve the quartic equation x4 2x2 8x 8
0 we factorised it as (x2 ?2 x 2?2)(x2
?2x 2?2) 0 - The four solutions are
- x
- There is some symmetry to these solutions
- Roughly speaking, a symmetry of the roots of an
equation is a way of swapping them round (a
permutation) so that if an equation with integer
coefficients is satisfied by the roots, it is
still satisfied after the roots are permuted
21
A very simple example
The quadratic equation x2 4x 2 0 has roots
x1 2 ?2 and x2 2 ?2 Any polynomial
equation satisfied by x1 is also satisfied by x2
Swapping x1 and x2 is a symmetry of the roots.
Call it s Not swapping them is clearly also a
symmetry. Call it n The table shows how n and s
combine when one is followed by another
22
A brief introduction to group theory
- In the early 19th century, mathematicians were
starting to study groups of permutations - A group is not just a set of objects. It also
has a structure - Sets with structure are what abstract algebraists
study - A group has an operation defined on it (e.g.
addition, multiplication, composition of
functions) such that if you combine two elements
of the group using this operation, you get an
element of the group. Also - There is an identity element (0 for , 1 for x)
- Every element has an inverse (a for , 1/a for
x) - The operation is associative a(bc) (ab)c
23
To cut a long story short ...
- The symmetries of the roots of an equation form
a group - Does this group give us any information about
the roots of the equation? - The answer turns out to be yes!
- A polynomial equation is solvable by radicals
if, and only if, the group of symmetries of its
roots is a solvable group - Solvable means that the group splits up into
smaller pieces in a particular way - The general quintic (and higher order) equation
does not have a solvable group, so it is not
solvable by radicals
24
Do you know this man?
- Evariste Galois, born Paris 1811
- Not a great success at school! Grew bored and
rebellious - Read books on Maths and tried to do original
work, but this was disorganised and not
appreciated - Got involved in republican politics
- Challenged to a duel
- Died 2nd June 1832, aged 20
25
Galoiss legacy
- Galoiss work was eventually appreciated as a
true work of genius, laying the foundations of
modern pure mathematics - He explained the connection between symmetry
groups and solvability of equations. This topic
is now known by the name of Galois Theory - In doing this he made big advances in group
theory, which has since been used to analyse
symmetry in geometrical figures, crystals, atomic
particles, etc - Work on solvability of equations led to some
significant geometric results, e.g. impossible to
trisect an angle by ruler and compass, or square
the circle.
26
Explore further
- There is an article on Galois Theory on the
NRICH website - http//nrich.maths.org
- (Type Galois in the search box)