12'10 Two and threedimensional representations of fMRI data'

1
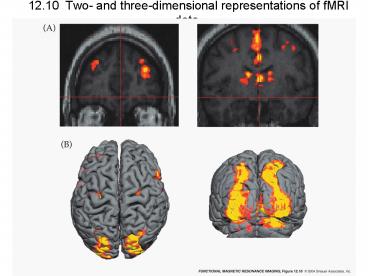
12.10 Two- and three-dimensional representations
of fMRI data.
2
12.12 Flat map views of the brain surface. (Part
1)
3
12.12 Flat map views of the brain surface. (Part
2)
4
12.11 Glass-brain views of fMRI data.
5
12.1 Statistical maps of fMRI data.
6
Statistics, more than most other areas of
mathematics, is just formalized common
sense. Paulos (1992)
7
What is statistics?

• Data require reduction and interpretation
• What do we do with a bunch of numbers?
• Statistics allow us to summarize and interpret
  data.
• Descriptive statistics
• Central tendency
• Variability
• Inferential statistics
• Significance testing
• Confidence intervals

8
Variability

• How spread out are the data?
• Tendency for the scores to differ from the
  central tendency

9
8.1 How fMRI data are organized.
10
7.11 The first use of BOLD fMRI for functional
mapping of the human brain. (Part 2)
11
11.9 Within-conditions and between-conditions
variability in blocked fMRI data.
12
12.5 Conducting a t-test.
13
12.6 Assigning time points in a blocked design
to t-conditions.
14
7.12 Changes in BOLD activity associated with
presentations of single discrete events.
15
7.17(A) A sample fMRI time course from a single
voxel in the motor cortex.
16
7.17(B) Data from the individual trials that
make up Figure 7.17(A).
17
Sources of Variability ExampleFigure 11.15 (Part
2)
18
9.6 A map of noise across the brain.
19
10.6 Edge effects of head motion in fMRI
analyses.
20
9.7 Scanner drift.
21
12.4 The Students t-distribution.
22
12.2 Types of experimental errors.
23
Defining Probabilities
1- ?
?
?
1-?

• ? probability of a TYPE I error
• Type I when H0 is wrongly rejected
• ? probability of a TYPE II error
• Type II failing to reject H0 when it is actually
  false

24
Defining Probabilities

• Relationship between ? and ?

25
Defining Probabilities

• Think in terms of H0 and H1 distributions

26
Power

• Power to detect a difference if it exists
• If H1 is true, how likely are you to reject H0?
• How good is your decision rule?

27
Factors Affecting Power

• Value of H1 (?0 - ?1)
• Size of ?
• Sample size
• Error variance

28
Value of H1 (?0 - ?1)

• With ?, N, and ? kept constant

Critical value

• Power increases as distance between the ?0 and ?1
  increases

29
Effect of Changing ?

• With ?0 - ?1, N, and ? kept constant

• Power increases as ? increases

30
Effect of Variance

• With ?0 - ?1, ?, and N kept constant

Critical value

• Power increases as ? decreases

31
Effect of Sample Size

• With ?0 - ?1, ?, and ? kept constant

Critical value

• Power increases as N increases

32
Populations vs Samples

• Population--set of all possible members
• Every person/case that fits our interests is
  accounted for
• Equivalent to the space S
• Sample--subset of the population
• Selected group from the population
• Used to make estimates about the population

http//www.ruf.rice.edu/7Elane/stat_sim/sampling_
dist/index.html
33
Problems with samples

• Imperfect estimates of the population
• One must determine the quality of the estimate
  coming from the statistics
• Hence the 52 5
• This is where the theoretical distributions come
  in to play
• It is crucial to sample the population
  appropriately

34
What can you actually do?

• ? difference between ?0 ?1 NOT USUALLY
• The differences are occurring in the real world
• Use higher field magnet?

• ? ? MAYBE
• Do you have a principled reason?
• Cost/Benefits analysis?

• ? Sample size YEP
• Unless it is practically impossible

• UPSHOT? Most things are beyond your control, but
  you need to be aware of them

• ? Error variance MAYBE
• You might be able to tighten your design
• Increase number of trials?
• Use better measures?

35
Common Sense

• When we reject H0, we say the differences were
  significant
• What are significant results really telling us?
• Unlikeliness
• Surprise
• What is practically important?
• Why 0.05 or 0.01?

36
12.13 Basic principles of the general linear
model in fMRI.