Chapter 11: Rotational Dynamics and Static Equilibrium

1
Chapter 11 Rotational Dynamics and Static
Equilibrium

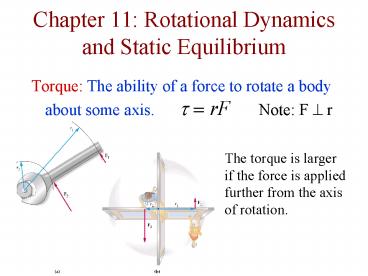
• Torque The ability of a force to rotate a body
  about some axis. t rF Note F ? r

The torque is larger if the force is applied
further from the axis of rotation.
2
Rotational Dynamics

• Which force has the greatest effect on the
  rotation of the door?

b
pivot
a
c
3

• By convention, the sign of torque is
• t lt 0 clockwise (cw)
• t gt 0 counter-clockwise (ccw)

4
General Definition of Torque

• Only the component of the force that is
  perpendicular to the radius causes a torque.
• r(Fsinq)
• Equivalently, only the perpendicular distance
  between the line of force and the axis of
  rotation, known as the moment arm r?, can be used
  to calculate the torque.
• t r?F (rsinq)F

5

• Each force that acts on an object may cause a
  torque.

F1

• In this figure, the three forces have equal
  magnitude.
• Which forces cause a torque?
• Which force causes the biggest magnitude
  torque?
• Which forces, if any, causes a positive torque?

When discussing torques, we must identify a pivot
point (or axis of rotation).
The net torque about a point O is the sum of all
torques about O St t1 t2 ...
6
Example 1
Calculate the net torque on the 0.6-m rod about
the nail at the left. Three forces are acting on
the rod as shown in the diagram.
7
Moment of Inertia

• Recall that mass (inertia) is an objects
  resistance to acceleration. Similarly an
  objects resistance to rotation (angular
  acceleration) is known as moment of inertia. For
  a point mass m
• I mr2
• I moment of inertia
• r distance from the axis of rotation
• For an extended object
• I Smiri2
• Mass near the axis of rotation resists rotation
  less than mass far from the axis of rotation.

8
Angular Position, q

• For circular motion, the distance (arc length) s,
  the radius r, and the angle ? are related by

q gt 0 for counterclockwise rotation from
reference line
Note that ? is measured in radians
1 rev 360 2p rad
9

• Consider a rotating disk

P
r
s
r
?
O
O
P
t gt 0
t 0
10
Angular Velocity, w

• Notice that as the disk rotates, ? changes. We
  define the angular displacement, ??, as
• ?? ?f - ?i
• which leads to the average angular speed wav

11
Instantaneous Angular Velocity

• As usual, we can define the instantaneous angular
  velocity as

• Note that the SI units of ? are rad/s s-1
• w gt 0 for counterclockwise rotation
• lt 0 for clockwise rotation
• If v speed of a an object traveling around a
  circle of radius r
• w v / r

12
Walker Problem 4, pg. 297
Express the angular velocity of the second hand
on a clock in the following units (a) rev/hr (b)
deg/min and (c) rad/s.
How long does it take for the second hand to
complete one revolution? How many degrees in one
revolution? How many radians in one revolution?
13
Period
The period of rotation is the time it takes to
complete one revolution.
T period
Rearranging we have
What is the period of the Earths rotation about
its own axis? What is the angular velocity of the
Earths rotation about its own axis?
14
Angular Acceleration, a

• We can also define the average angular
  acceleration aav
• and

The SI units of a are rad/s2 s-2 We will skip
any detailed discussion of angular acceleration,
except to note that angular acceleration is the
time rate of change of angular velocity
15
Torque and Angular Acceleration

• Recall Newtons Second Law F ma
• The net force on an object of mass m causes a
  (linear) acceleration a.
• Similarly, the net torque on an object with
  moment of inertia I causes an angular
  acceleration a.
• t Ia

16
Partial derivation of role of torque.
v
F
q
r

• Consider a point mass m constrained to
• move in a circle of radius r.
• The centripetal acceleration is ac v2/r
• The change in speed is determined by the
  component of force parallel to motion (and
  therefore perpendicular to the radius)
• ma F? Fsinq
• mr a F?
• a rF? / mr2 t / I
• In order for a system to not change its state of
  rotation, the external torque applied to the
  system must be 0.

17
Zero Torque and Static Equilibrium
Consider the wheel shown below. Two forces of
equal magnitude are acting on the wheel. Will
the wheel remain at rest? NO it will
rotate! The net force is zero, so there will be
no linear acceleration.
However, the sum of the torques is not zero, so
there will be an angular acceleration. The
wheel is not in static equilibrium.
18
Conditions for Static Equilibrium

• For true static equilibrium, two conditions must
  be satisfied
• For an object in equilibrium, the axis of
  rotation is arbitrary (But all torques must be
  evaluated about a common axis).

19
Walker Problem 30, pg 341
A rigid, vertical rod of negligible mass is
connected to the floor by an axle through its
lower end, as shown in the Figure. The rod also
has a wire connected between its top and the
floor. If a horizontal force F is applied at the
midpoint of the rod, find (a) the tension in the
wire, and (b) the horizontal and vertical
components of force exerted by the bolt on the
rod.
20
T
y
F
x
P
mg?0
Static Equilibrium SFx 0 F Tcos(180º45º)
Px 0 Sfy 0 PyTsin(180º45º)-mg 0 St
0 (-F)(L/2) (Tcos45º)L 0
T F / (2 cos45º) F / ?2 cos(180º45º) - 1/
?2 sin (180º45º) Px - F Tcos(180º45º)
- F (F/?2)(-1/ ?2) Px - F F/2 -F/2 Py
mg T/ ?2 mg F/2 ? F/2
21
Center of Mass and Balance
Recall that an object will hang with it center of
mass (CM) directly below the point of suspension.
Now we can understand why. If the objects CM
is not below the point of suspension, its weight
will cause a torque which rotates the object
until its CM is below the point of suspension.

• gravitational torque 0
• gravitational torque rotates the paintbrush

22
Base of Support
An object at rest on a surface is in equilibrium
(will not tip over) if its center of mass is
above the base of support. The base of support
is the area bounded by whatever is touching the
floor.
The shaded area between the legs of the table is
the base of support.
Why isnt it possible to touch your toes if you
are standing flush against a wall?
23
Walker Problem 38, pg. 342
A baseball bat balances 71.1 cm from one end. If
a 0.560-kg glove is attached to that end, the
balance point moves 24.7 cm toward the glove.
Find the mass of the bat.
24
Angular Momentum

• For linear momentum
• p mv
• For rotational motion, we define an angular
  momentum
• L r mv? mr2w Iw

The SI units of angular momentum are kgm2/s
25
Walker Problem 54, pg. 343
Two gerbils run in place with a linear speed of
0.45 m/s on an exercise wheel that is shaped like
a hoop. Find the angular momentum of the system
if each gerbil has a mass of 0.33 kg and the
exercise wheel has a radius of 9.5 cm and a mass
of 5.0 g.

• All mass is at radius r 0.095m
• I mr2 (0.005 20.33)kg (0.095m)2
• I 0.00600 kg m2
• L I w
• v/r (0.45 m/s) / (0.095 m) 4.74/s
• L (0.00600 kg m2) (4.74 /s)
• L 0.028 kg m2/s

V0.45 m/s
26

• Recall that FDt Dp
• For rotational motion
• tDt DL
• Conservation of Angular Momentum
• If then

• With zero external torque, Angular momentum is
  constant, even if internal forces cause a change
  in the distribution of mass.
• Elliptic orbits (chap. 12) L constant
• Ice skater moving arms in, radius shrinks,
  Moment of inertia I shrinks, L Iw constant, w
  increases.

27
Walker Problem 61, pg. 343
A student sits at rest on a piano stool that can
rotate without friction. The moment of inertia
of the student-stool system is 4.1 kgm2. A
second student tosses a 1.5-kg mass with a speed
of 2.7 m/s to the student on the stool, who
catches it at a distance of 0.40 m from the axis
of rotation. What is the resulting angular speed
of the student and the stool? Assume that the
velocity of the mass, before it is caught, is
tangential to a circle of radius 0.4 m from the
axis of rotation.
28
Walker Problem 63, pg. 343
A turntable with a moment of inertia of 5.4 ?
10-3 kgm2 rotates freely with an angular speed
of 33.33 rpm. Riding on the rim of the
turntable, 15 cm from the center, is a 1.3-g
cricket. (a) If the cricket walks to the center
of the turntable, will the turntable rotate
faster, slower, or at the same rate? Explain.
(b) Calculate the angular speed of the turntable
when the cricket reaches the center.
29
Kinetic energy of rotation
What is the kinetic energy of a mass m traveling
at speed v in a circle of radius r? K (1/2) m
v2 (1/2) mr2 (v/r) 2 (1/2) I w2 Kinetic
energy of rotation (1/2) I w2 L2 / (2I) This
is not a new form of energy, just a re-labeling
(or alternate formula) for kinetic energy.
30
Quiz

• Two masses, 1kg and 0.5 kg,are balanced on a
  fulcrum at radii 0.20m and 0.40 m, respectively.
• Now the lighter mass is hung from the same
  position as before, but with a string of length
  0.20 m.
• Choose the correct answer
• The 0.5 kg mass swings down (longer length
  larger torque)
• The system remains balanced (torques dont
  change)
• The 0.5 kg mass swings up (it is lighter than
  the 1kg mass).

1kg
0.5kg
1kg
0.5kg
31
Gravitational Torque
y

• Gravity generates a torque as if the entire force
  were concentrated at the Center-of-Mass.
• Consider a discrete set of point masses m1, m2,
• Gravitational torque acting on each mass is
  x-coordinate times force of gravity (since
  gravity is perpendicular to x-direction)
• Torque x1 m1 g x2 m2 g
• (x1 m1 x2 m2 )g XCM Mg

x
m1g
m3g
32
Gravitational Potential Energy

• Single mass U mgh
• Mass distribution U mgyCM
• Stability
• An object in equilibrium is stable, if any tiny
  displacement (rotation or translation) causes the
  center of gravity to rise.

33
Gravitational Stability

• Stable
• CM rises
• Unstable
• CM falls

34
Angular Momentum

• For linear momentum
• p mv
• For rotational motion, we define an angular
  momentum
• L r mv? mr2w Iw

The SI units of angular momentum are kgm2/s
35

• Recall that FDt Dp
• For rotational motion
• tDt DL
• Conservation of Angular Momentum
• If then

• With zero external torque, Angular momentum is
  constant, even if internal forces cause a change
  in the distribution of mass.
• Elliptic orbits (chap. 12) L constant
• Ice skater moving arms in, radius shrinks,
  Moment of inertia I shrinks, L Iw constant, w
  increases.

36
Kinetic energy of rotation
What is the kinetic energy of a mass m traveling
at speed v in a circle of radius r? K (1/2) m
v2 (1/2) mr2 (v/r) 2 (1/2) I w2 Kinetic
energy of rotation (1/2) I w2 L2 / (2I) This
is not a new form of energy, just a re-labeling
(or alternate formula) for kinetic energy.
37
Hurricane

• Coriolis Force Conservation of Angular Momentum
• Pressure differential
• (1000mBar outside-882 mBar inside Wilma)