Integration of Trigonometric Polynomials - PowerPoint PPT Presentation
1 / 9
Title:
Integration of Trigonometric Polynomials
Description:
General Trigonometric Polynomials. Mika Sepp l : Integration by Substitution ... If both m and n are even use the trigonometric formulae ... – PowerPoint PPT presentation
Number of Views:104
Avg rating:3.0/5.0
Title: Integration of Trigonometric Polynomials
1
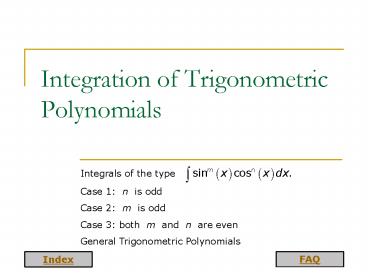
Integration of Trigonometric Polynomials
Integrals of the type
Case 1 n is odd Case 2 m is odd Case 3
both m and n are even General Trigonometric
Polynomials
2
Basic Trigonometric Monomials 1
Problem
Example
This computation was possible since the function
to be integrated was a (simple) polynomial in the
sine function times the cosine function.
3
Basic Trigonometric Monomials 2
Problem
Case 1 n is odd.
If n 2k1 is odd then one can rewrite the
function to be integrated using the formula
This can be reduced to an integral of a
polynomial by the substitution t sin(x).
4
Basic Trigonometric Monomials 3
Example
Solution
Rewrite
The last integral is easy to compute. One gets
5
Basic Trigonometric Monomials 4
Problem
Case 2 m is odd
If m 2k1 is odd then one can rewrite the
function to be integrated using the formula
This can be reduced to an integral of a
polynomial by the substitution t cos(x).
6
Basic Trigonometric Monomials 5
Example
Solution
Rewrite
The last integral is easy to compute. One gets
7
Basic Trigonometric Monomials 6
Problem
Case 3 m and n even
If both m and n are even use the
trigonometric formulae
to simplify the function to be integrated.
8
Basic Trigonometric Monomials 7
Example
Solution
Now use the same trig formula again!
9
Integration of Trigonometric Polynomials
Problem
Solution