Chapter%201:First%20order%20Partial%20Differential%20Equations

1
Chapter 1First order Partial Differential
Equations
Sec 1.2 The Linear Equation
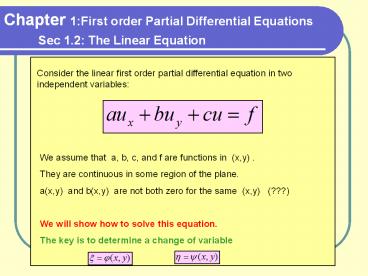
Consider the linear first order partial
differential equation in two independent
variables
We assume that a, b, c, and f are functions in
(x,y) . They are continuous in some region of the
plane. a(x,y) and b(x,y) are not both zero for
the same (x,y) (???) We will show how to
solve this equation. The key is to determine a
change of variable
2
Chapter 1First order Partial Differential
Equations
Sec 1.2 The Linear Equation
PDE
ODE
Consider the ODE
1st order linear ODE
Method
Find integrating factor K
Multiply equation by K
LHS
Integrate both sides
3
Chapter 1First order Partial Differential
Equations
Sec 1.2 The Linear Equation
Characteristic equation
Consider the linear 1st PDE
Find the general solution of thePDE
solution
4
Chapter 1First order Partial Differential
Equations
Sec 1.2 The Linear Equation
Consider the linear first order partial
differential equation in two independent
variables

1. Find the characteristic equation
2. Find the general solution of the characteristic
   equation and put it in the form
3. Use the transformation
4. To change PDE into this form

5
Chapter 1First order Partial Differential
Equations
Sec 1.2 The Linear Equation
Consider the linear 1st PDE
1
characteristic equation
Solution
2
transformation
3
4
6
Chapter 1First order Partial Differential
Equations
Sec 1.2 The Linear Equation
Consider the linear 1st PDE
characteristic curves
1
characteristic equation
C4
Solution
2
C1
transformation
3
C-4
4