Dripline Nuclei as Open Quantum Systems - PowerPoint PPT Presentation
1 / 15
Title:
Dripline Nuclei as Open Quantum Systems
Description:
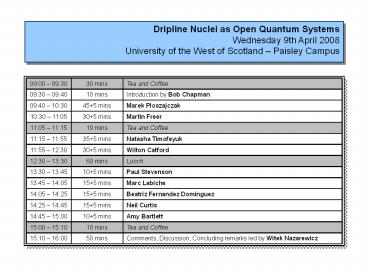
Comments, Discussion, Concluding remarks led by Witek ... Natasha Timofeyuk. 35 5 mins. 11:15 11:55. Tea and Coffee. 10 mins. 11:05 11:15. Martin Freer ... – PowerPoint PPT presentation
Number of Views:23
Avg rating:3.0/5.0
Title: Dripline Nuclei as Open Quantum Systems
1
Dripline Nuclei as Open Quantum Systems Wednesday
9th April 2008 University of the West of Scotland
Paisley Campus
2
Dripline nuclei as open quantum systems 9th April
2008, University of the West of Scotland (Paisley
Campus)
- BIG Questions
- How to unify structure and reaction aspects of
nuclei? - How to incorporate open-channels effects into
microscopic nuclear structure models? - How to incorporate structure information into
microscopic reaction models? - Crucial Questions
- How are cluster configurations born in nuclei?
What is the microscopic foundation of the Ikeda
diagram? - Is spectroscopic factor a useful concept?
- Can we extract useful spectroscopic information
from threshold effects ? - What is the structure of the many-body continuum?
- What is the interplay between continuum and
isospin-breaking effects?
3
Prog. Part. Nucl. Phys. 59, 432 (2007)
4
The nucleus is a correlated open quantum
many-body system Environment continuum of decay
channels
7162
6049
Spectra and matter distribution modified by the
proximity of scattering continuum
5
Physics of the large neutron excess
- Interactions
- Isovector (N-Z) effects
- Poorly-known components come into play
- Long isotopic chains crucial
- Configuration interaction
- Mean-field concept often questionable
- Asymmetry of proton and neutron Fermi surfaces
gives rise to new couplings (Intruders and the
islands of inversion) - New collective modes polarization effects
- Open channels
- Nuclei are open quantum systems
- Exotic nuclei have low-energy decay thresholds
- Coupling to the continuum important
- Virtual scattering
- Unbound states
- Impact on in-medium Interactions
6
(7.27)
(14.44)
(19.17)
(28.48)
(7.16)
(11.89)
(21.21)
(14.05)
(4.73)
Excitation energy
(13.93)
(9.32)
11Li
Mass number
7
Example Threshold anomaly
E.P. Wigner, Phys. Rev. 73, 1002 (1948), the
Wigner cusp G. Breit, Phys. Rev. 107, 923
(1957) A.I. Baz, JETP 33, 923 (1957) R.G.
Newton, Phys. Rev. 114, 1611 (1959). A.I. Baz',
Ya.B. Zel'dovich, and A.M. Perelomov, Scattering
Reactions and Decay in Nonrelativistic
Quantum Mechanics, Nauka 1966 A.M. Lane, Phys.
Lett. 32B, 159 (1970) S.N. Abramovich, B.Ya.
Guzhovskii, and L.M. Lazarev, Part. and Nucl. 23,
305 (1992).
- The threshold is a branching point.
- The threshold effects originate in conservation
of the flux. - If a new channel opens, a redistribution of the
flux in other open channels appears, i.e. a
modification of their reaction cross-sections. - The shape of the cusp depends strongly on the
orbital angular momentum.
aX a1X1 at Q1 a2X2 at Q2 anXn at Qn
aX
8
Threshold anomaly (cont.)
Studied experimentally and theoretically in
various areas of physics
- pion-nucleus scattering
- R.K. Adair, Phys. Rev. 111, 632 (1958)
- A. Starostin et al., Phys. Rev. C 72, 015205
(2005) - electron-molecule scattering
- W. Domcke, J. Phys. B 14, 4889 (1981)
- electron-atom scattering
- K.F. Scheibner et al., Phys. Rev. A 35, 4869
(1987) - ultracold atom-diatom scattering
- R.C. Forrey et al., Phys. Rev. A 58, R2645
(1998) - Low-energy nuclear physics
- charge-exchange reactions
- neutron elastic scattering
- deuteron stripping
The presence of cusp anomaly could provide
structural information about reaction products.
This is of particular interest for neutron-rich
nuclei
9
Coupling between analog states in (d,p) and (d,n)
C.F. Moore et al. Phys. Rev. Lett. 17, 926 (1966)
10
C.F. Moore et al., Phys. Rev. Lett. 17, 926 (1966)
11
The one-nucleon radial overlap integral
Spectroscopic factor
Complete set
12
The importance of the particle continuum was
discussed in the early days of the
multiconfigurational Shell Model and the
mathematical formulation within the Hilbert space
of nuclear states embedded in the continuum of
decay channels goes back to H. Feshbach
(1958-1962), U. Fano (1961), and C. Mahaux and H.
Weidenmüller (1969)
- unification of structure and reactions
- resonance phenomena generic to many small quantum
systems coupled to an environment of scattering
wave functions hadrons, nuclei, atoms,
molecules, quantum dots, microwave cavities, - consistent treatment of multiparticle correlations
Open quantum system many-body framework
Gamow (complex-energy) Shell Model
(2002 -) N. Michel et al, PRL 89
(2002) 042502 R. Id Betan et al, PRL 89 (2002)
042501 N. Michel et al, PRC 70 (2004) 064311 G.
Hagen et al, PRC 71 (2005) 044314
Continuum (real-energy) Shell Model
(1977 - 1999 - 2005) H.W.Bartz et al, NP A275
(1977) 111 R.J. Philpott, NP A289 (1977) 109 K.
Bennaceur et al, NP A651 (1999) 289 J. Rotureau
et al, PRL 95 (2005) 042503
13
Rigged Hilbert space Gamow Shell Model (2002)
One-body basis
J. Rotureau et al., DMRG Phys. Rev. Lett. 97,
110603 (2006)
non-resonant continuum
bound, anti-bound, and resonance states
Michel et al. Virtual states not included
explicitly in the GSM basis Phys. Rev. C 74,
054305 (2006)
14
N. Michel et al. PRC 75, 0311301(R) (2007)
WS potential depth decreased to bind 7He.
Monopole SGI strength varied
Overlap integral, basis independent!
Anomalies appear at calculated thresholds
(many-body S-matrix unitary) Scattering
continuum essential
see also Nucl. Phys. A 794, 29 (2007)
15
118Sn(t,p)120Sn
Large yield to ground state
superconducting Sn ground states