Propagation of Error

1

• Propagation of Error
• Calculation of error in an a calculated result
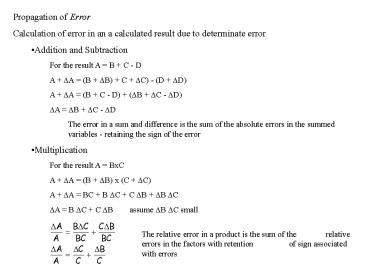
  due to determinate error
• Addition and Subtraction
• For the result A B C - D
• A ?A (B ?B) C ?C) - (D ?D)
• A ?A (B C - D) (?B ?C - ?D)
• ?A ?B ?C - ?D
• The error in a sum and difference is the sum of
  the absolute errors in the summed variables -
  retaining the sign of the error
• Multiplication
• For the result A BxC
• A ?A (B ?B) x (C ?C)
• A ?A BC B ?C C ?B ?B ?C
• ?A B ?C C ?B assume ?B ?C small

The relative error in a product is the sum of the
relative errors in the factors with retention
of sign associated with errors
2

• Propagation of Error
• Calculation of error in an a calculated result
  due to determinate error
• Quotients

The relative error in a
quotient is the difference between the relative
error in the numerator minus the relative
error in the denominator - retaining the
signs of the errors
3

• Propagation of Uncertainty
• The calculation of the accumulated uncertainties
  in a calculated result when indeterminate errors
  exists makes use of the calculation of the most
  probable value of the uncertainty in the result
• This will involve the standard deviation - either
  s or ?
• The value of s or ? must be known for each
  measurement

4
(No Transcript)
5
Propagation of Uncertainties For addition and
subtraction
The absolute standard deviation of a sum or
difference is the square root of the sum of the
variances of the numbers combined
Example Calculate the standard deviation in the
sum y (0.50 ? 0.02) (4.10 ? 0.03) - (1.97 ?
0.05) 2.63
6
Propagation of Uncertainty
For multiplication and division
The relative uncertainty in the result of a
multiplication or division is the square root
of the sum of the relative variances of the
factors in the calculation
7
Propagation of Uncertainty Multiplication and
division example
8
Propagation of Uncertainty For exponentiation
The relative uncertainty in the result of an
exponentiation is the product of the constant
exponent times the relative uncertainty in the
base number
Compare to exponentiation to multiplication
9
Propagation of Uncertainty For logs
For antlogs
The relative standard deviation in an antilog
of a measurement is 2.303 times the standard
deviation in the measurement
The standard deviation of the log of a measured
result is 0.434 times the relative standard
deviation in the measurement
10

• Propagation of Uncertainty
• Log and antilog examples
• ylog(2.00 ? 0.02) x 10-4 -3.6990??
• Yantilog1.200 ? 0.003 15.849 ? ?

11

• Significant Figures
• The significant figure convention reports
  measurements and the results of calculations to
  all the digits that are certain plus one
  uncertain digit
• The uncertain digit is the least significant
  digit
• If a mass is reported to 1.2345 g, the digits 1,
  2, 3, 4 are known with certainty and the 5 is
  uncertain
• A problem with the sig. fig. convention is that
  the uncertainty in the least sig. fig. is not
  stated
• Zeros in measurements or calculated results
• Leading zeros - zeros to the left of non-zero
  digits - are not significant
• 01234 0.01234
• Trailing zeros - zeros to the right of non-zero
  digits - that are to the left of a decimal point
  are not significant
• 1230 12300
• Trailing zers to the right of a decimal point are
  significant
• 123.0 12.3000
• Embedded zeros are significant
• 1203 12003 1.203 1230.0

12
Significant Figures To find the least sig. fig.,
propagate the uncertainties
This result fits the General Chemistry rules!
Another example
This result does not fit the General Chemistry
rules! The relative uncertainty for both results
is about the same, and since y is about the
same for both results, the absolute uncertainty
is about the same
13

• Significant Figures
• For logs and antilogs, it often occurs that the
  number of sig. figs. in the result is the same
  as in the argument of the log
• Example