Section 6.1 Circles and Related Segments and Angles - PowerPoint PPT Presentation
Title:
Section 6.1 Circles and Related Segments and Angles
Description:
... that joins two points of a circle is a chord. ... the inscribed angle with chords SR and ST as ... Theorem 6.15: congruent chords have congruent minor ... – PowerPoint PPT presentation
Number of Views:162
Avg rating:3.0/5.0
Title: Section 6.1 Circles and Related Segments and Angles
1
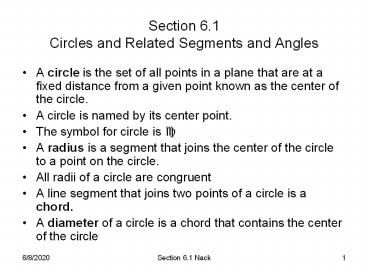
Section 6.1Circles and Related Segments and
Angles
- A circle is the set of all points in a plane that
are at a fixed distance from a given point known
as the center of the circle. - A circle is named by its center point.
- The symbol for circle is ?
- A radius is a segment that joins the center of
the circle to a point on the circle. - All radii of a circle are congruent
- A line segment that joins two points of a circle
is a chord. - A diameter of a circle is a chord that contains
the center of the circle
2
Segments of a Circle
- What are the radii of ?Q?
- QS, QW, QV, QT
- What is the diameter?
- WT
- What is the chord?
- SW, TW
- Congruent circles are two or more circles that
have congruent radii.
3
Relationships Concerning Circles
- An arc is a segment of a circle determined by two
points on the circle and all the points in
between. ABC is an arc denoted by ABC. - A semicircle is the arc determined by a diameter
- A minor arc is part of a semicircle.
- A major arc is more than a semicircle but less
than a circle
- Concentric Circles are coplanar circles that have
a common center. - Theorem 6.1.1 A radius that is perpendicular to
a chord, bisects the chord.
O
D
A
P
C
B
4
Angles and Arcs
- A central angle of a circle is an angle whose
vertex is the center of the circle and whose
sides are radii. - An intercepted arc is determined by the two
points of intersection of the angle with the
circle and all points of the arc in the interior
of the angle. - ?ROT is a central angle of ?O.
- RT is the intercepted arc.
5
Angle and Arc Relationships in the Circle
- Postulate 16 (Central Angle Postulate) In a
circle, the degree measure of a central angle is
equal to the degree measure of the intercepted
arc. - Congruent arcs are arcs with equal measure in
either a circle or congruent circles. - m?AOB m?COD
- m AEB mCFD
C
A
F
O
E
B
D
6
- Postulate 17 (Arc-Addition Postulate) If B
lies between A and C on a circle, then mAB mBC
mABC An arc is equal to the sum of its parts. - Inscribed angle of a circle whose vertex is a
point on the circle and whose sides are chords of
the circle. - ?RST is the inscribed angle with chords SR and
ST as the sides. - Theorem 6.1.2 The measure of an inscribed angle
of a circle is one-half the measure of its
intercepted arc. - Case 1 One chord is a diameter (below)
- Case 2 The diameter lies inside the inscribed
angle p. 281 a - Case 3 The diameter lies outside the
inscribed angle p. 281 b - Proof Case 1 Draw the radius RO. ?ROS is
isoceles - with m?R m ?S.
- Since ?ROT is the exterior angle of ?ROS then
- m?ROT m?R m ?S 2 m?S
- Therefore, m?S ½ m?ROT.
- Since the measure of the arc is equal to the
measure - of the angle then by substitution m?S ½ m
RT.
7
More on Chords and Arcs
- In a circle (or in congruent circles),
- Theorem 6.1.3 congruent minor arcs have
congruent central angles p. 282. - Theorem 6.1.4 congruent central angles have
congruent arcs. - Theorem 6.15 congruent chords have congruent
minor (major) arcs. - Theorem 6.16 congruent arcs have congruent
chords.
C
A
O
P
B
D
8
- Theorem 6.1.7 Chords that are at the same
distance from the center of a circle are
congruent. Figure 6.18 - Theorem 6.1.8 Congruent chords are located at
the same distance from the center of the circle.
Figure 6.18 - Theorem 6.1.9 An angle inscribed in a
semicircle is a right angle. Figure 6.19 p. 282 - Theorem 6.1.10 If two inscribed angles
intercept the same arc, then these angles are
congruent. Figure 6.20