Engineering Mathematics II - PowerPoint PPT Presentation
1 / 55
Title:
Engineering Mathematics II
Description:
Special functions are functions other than the elementary functions such as ... Hence deduce that. Mathematics II. 11. Show that. 12. Show that ... – PowerPoint PPT presentation
Number of Views:143
Avg rating:3.0/5.0
Title: Engineering Mathematics II
1
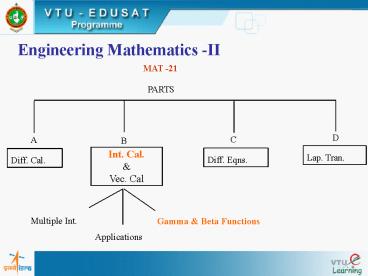
Engineering Mathematics -II
MAT -21
PARTS
D
A
C
B
Lap. Tran.
Int. Cal. Vec. Cal
Diff. Eqns.
Diff. Cal.
Multiple Int.
Gamma Beta Functions
Applications
2
Gamma and Beta Functions
Introduction
Special functions are functions other than the
elementary functions such as algebraic and
transcendental functions. They are defined as
integrals many times as improper integrals.
They arise as solutions of differential equations
and are very useful in physical problems.
Gamma and Beta are two of many such functions.
They were discovered by L. Euler and are referred
to also as Eulers integrals of the second and
first kind respectively. They are improper
integrals and are denoted by B(m,n) and ?(n)
where m and n are positive real numbers.
Gamma function is some times referred to as
factorial function as it emerged out of a search
for a function generalizing the factorial
expressions for natural numbers.
3
Gamma Function
Definition The improper integral
is defined as the gamma function. Here n is
a real number called the parameter of the
function. ?(n) exists for all real values of
n except 0, -1, -2, .. Its graph is shown
below
4
5
6
Gamma Function
Recurrence formula
integrating by parts.
7
Gamma Function
Applying the definition of Gamma function and
using
8
Note (1) ??n? is not convergent when n 0,
-1, -2, . (2) If ??n? is known for 0 lt n lt 1,
then its values for 1 lt n lt 2 can be
found using equation (2). Also, its
values for -1 lt n lt 0 can be got using equation
(1). (3) If n is a positive integer, using the
recurrence relation and ??1? 1, we get
??n? (n - 1) ??n - 1? (n - 1) (n - 2)
?(n - 2) and so on (n - 1) (n - 2) (n - 3)
.. 1 (n - 1) !
9
Gamma Function
Problems (1) Prove that
By definition,
10
Gamma Function
11
Gamma Function
Converting Cartesian (x, y) to polar (r, ?)
system, we get
12
Gamma Function
?
Taking square roots on both sides, we get
13
Gamma Function
(2) Show that
14
Gamma Function
(3) Find
using ln x -y, we get x e - y , dx
-e - y dy and y ranges from ? to 0 when x
0 to 1
interchanging the lower and the upper limits
15
Gamma Function
choosing 6y t
16
Gamma Function
(4) Prove that
using
dx -ae-t dt and t ? to 0 when x
0 to a
17
Gamma Function
18
Gamma Function
(5) Show that
. Hence show that
when 'n' is a positive integer and m gt
-1.
19
Gamma Function
Let logx - t ? dx -e-t dt
and t ? to 0 when x 0 to 1.
using
20
Gamma Function
choosing
the required result.
21
Beta function
Definition Beta function, denoted by B(m,n)
is defined by
where m and n are positive real numbers.
22
By a property of definite integral,
we have B(m,n) B(n,m)
23
Alternate Expressions for B(m,n)
and t ? to 0 when x 0 to 1.
24
25
26
Relation between Gamma and Beta functions.
Prove that
Proof We know that
27
in polar coordinates
?(mn) B(m,n) by definitions.
28
Note
We get ?(n) ?(1-n) ?(1) B (1-n,n)
using definition of Beta function.
?(n) ?(1-n) ? /sin n?, 0 lt n lt 1
29
Problems (1) Show that
and t 0 to 1 when x 0 to 1
30
31
- (2) Evaluate
B(11,19) B (19, 11)
32
(3) Evaluate
33
(4) Show that
34
(No Transcript)
35
(5) Prove that
36
37
(6) Prove the Duplication formula
Using the previous result,
38
(No Transcript)
39
40
(7) Prove that
By one of the definitions of Beta function, we
have
41
I1 I2 (say)
Substituting
42
Observe how Gamma and Beta functions are useful
in evaluating complicated integrals.
43
Multiple Choice
1. The value of
- ½
- (b) 7/3
- (c) 7/6
- (d) 7/2
44
2. The value of
- -1/12
- (b) -1/4
- (c) 0
- (d) -1/15
45
3. The value of
- 1
- (b) 0
- (c) 2
- (d) 8
46
4. The value of ?(-10) is
- 10!
- (b) 9!
- (c) -9!
- (d) Not defined
47
5. The value of ?(-5/2) is
(a)
(b)
(c)
(d) Not defined
48
- 0
- (10! 18!)/ 28!
- (9! 17!) / 27!
- (2! 9! 17!) / 27!
49
7.
(a)
(b) 2?
?
(c)
(d)
50
8. The value of B(1/2, 1/2) is
(a)
?
(b)
(c)
(d) 1
51
Practice questions
1.
2.
3.
52
(No Transcript)
53
7. If m and n are real constants gt -1,
prove that
8. Show that
Hint Write cos ax Real part of eiax
54
9. Show that
10. Show that
Hence deduce that
55
11. Show that
12. Show that
Hint use the substitutions x - a t and t
(b - a) y or x a (b -
a)t