ChiralOdd Twist3 Distribution Function ex

1
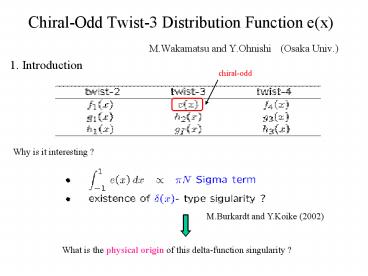
Chiral-Odd Twist-3 Distribution Function e(x)

• M.Wakamatsu and Y.Ohnishi (Osaka Univ.)

1. Introduction
2
The purpose of talk is twofold
(1) Physical Origin of -singularity
in
Nontrivial structure of QCD vacuum
(2) Theoretical predictions for e(x) in the
Chiral Quark Soliton Model (CQSM)
CLAS measurement of semi-inclusive DIS processes
3
2. Origin of delta-function singularity in e(x)
General definition of e(x)
with
4
Within the CQSM, we analytically confirmed this
behavior
M.W. and Y.Ohnishi, Phys. Rev. D67 (2003) 114011
Existence of this infinite-range correlation is
inseparably connected with
nontrivial vacuum structure of QCD
Spontaneous cSB and nonvanishing vacuum quark
condensate
5
Nucleon scalar quark density in the CQSM
total
6
This also dictates that
We thus conclude that
Nonvanishing quark condensate as a signal of the
spontaneous cSB of the QCD vacuum is the physical
origin of d(x)-type singularity in e(x)
7
3. Numerical study of e(x) in CQSM
General structure of e(x) in CQSM
Isoscalar part
in hedgehog M.F.
Isovector part
more complicated (double sum over levels)
8
Sophisticated numerical method to treat d(x)
contained in
Y,Ohnishi and M.W., Phys. Rev D69 (2004) 114002
We find that
where
with
9
1st moment sum rule for isoscalar e(x)
numerically
10
Isovector part of e(x)
total
valence
Dirac sea
regular behavior at x 0
11
Combining isoscalar- and isovector-part of e(x),
we can get any of
Comparison with CLAS data extracted by Efremov
and Schweitzer
12
4. Summary and Conclusion
(1) delta-function singularity in chiral-odd
twist-3 distribution e(x) is
Manifestation of nontrivial vacuum structure of
QCD in hadron observable
(2) Existence of this singularity will be
observed as
Violation of pN sigma-term sum rule of

need more precise experimental information on
this quantity in wider range of x