Root Locus and Lead Controllers

1
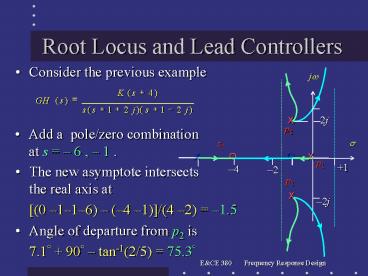
Root Locus and Lead Controllers

• Consider the previous example

• Add a pole/zero combinationat s 6 , 1 .

X
O

• The new asymptote intersectsthe real axis at
• (0 116) (4 1)/(4 2) 1.5

• Angle of departure from p2 is
• 7.1 90 tan-1(2/5) 75.3

2
Root Locus Lead Design

• Consider again the system defined by

Design Point

• Control design specifications

? ? 0.707
settling time ? 1.0 s
? ? ? ? 4
3
Root Locus Lead Design

• Can we force the root locus to go through the
  design point, s1 ?

• Consider the effect of adding a pole and zero
  combination (a lead compenstor).

X
4
Root Locus Lead Design

• Three effects of the pole zero combination
• modifies the real axis segments
• shifts the asymptote real axis intersection to
  the left
• modifies the angle criterion by
• ? ? ?z ? ?p

5
Root Locus Lead Design

• Design steps
• place the zero below the design point, s1
  (approx.)

• determine the angle difference, ? ? 90 ? ?p that
  will satisfy the angle criterion for the design
  point, s1 .

• locate the pole location from the angle, ?p and
  the location of the zero

6
Root Locus Lead Design

• calculate ?p

? ?135 ? 116.6 ? 180 ? ? 431.6 or 71.6 ? ?p
? 90 ? 71.6 ? 18.4
18.4
X
16

• calculate the pole location

tan(18.4) ? 4 / (? 4 ? p) ? p ? ?4/0.333 ? 4
? ? 16
7
Root Locus Lead Design

• The asymptote intersection

? ?a ? ? 7

• Determine the gain at the design point

K ? 5.56 4.47 12.65 / 4 ? 78.6
8
Root Locus Lead Design

• The final compensator is

• The final closed-loop systems is

Note the lead compensator ? ? 16/4 ? 4

• The compensated open-loops system is

9
(No Transcript)
10
Root Locus Lag Design

• Pole/zero placement
• The lag compensator is represented by a pole near
  the imaginary axis and a zero further to the left.

• Low frequency gain
• The compensator low frequency gain is

11
Root Locus Lag Design

• Desired effects of the lag
• increase the low frequency gain to achieve
  desired steady-state error specifications, the
  gain increase is ?? .

• Introduce very little effect on the path of the
  root locus, ie.

12
Root Locus Lag Design

• Relate the angle requirement and low frequency
  gain (note 2 0.035 rad.) By similar triangles

L
then
?
13
Root Locus Lag Design

• Design steps
• Determine a point, s1 on the uncompensated loci
  that satisfies the dynamic requirements.
• Find the gain at s1 and then the low frequency
  gain of the system.
• Determine the low frequency gain, ?? of the
  compensator required to meet the system
  steady-state error requirement.

• Calculate the compensator pole location using s1
  and ?? in the relationship

• Finally, calculate the compensator zero from

14
Root Locus Lag Design Example

• Consider again the system defined by

K 2 2 /1 4

• Control design specifications

? ? 0.50
ess ? 5 for a ramp input.
15
Root Locus Lag Design Example

• The velocity steady-state error constant is

• The compensator pole magnitude is

For ess ? 0.05, Kv ? 20
0.0128

• The compensator zero magnitude is

• Therefore the compensator low-frequency gain must
  be ?? ? 10 .

z ?? p 0.128
16
Root Locus Lag Design Example

• The final compensator is

• The final closed-loop systems is

• The compensated open-loops system is

• The roots of the final closed-loop systems are

s -0.9386 1.7000i s -0.1358
17
Root Locus of Final System
x
18
Step Responses
uncompensated
19
Ramp Response