Factoring Polynomials

1
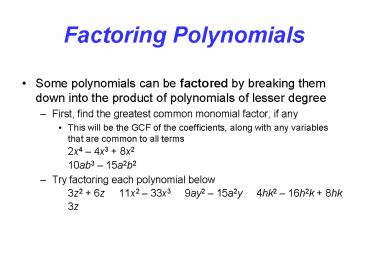
Factoring Polynomials

• Some polynomials can be factored by breaking them
  down into the product of polynomials of lesser
  degree
• First, find the greatest common monomial factor,
  if any
• This will be the GCF of the coefficients, along
  with any variables that are common to all terms
• 2x4 4x3 8x2 ? 2x2(x2 2x 4)
• 10ab3 15a2b2 ? 5ab2(2b 3a)
• Try factoring each polynomial below
• 3z2 6z 11x2 33x3 9ay2 15a2y
  4hk2 16h2k 8hk
• 3z (z 2) 11x2 (1 3x) 3ay (3y 5a)
  4hk (k 4h 2)

2
Factoring Polynomials

• Learn to recognize special patterns that can aid
  in factoring polynomials
• Difference of two squares
• Both terms are squares with one subtracted from
  the other
• a2 b2 ? (a b)(a b)
• For example x2 4 ? x2 22 ? (x 2)(x 2)
• Try factoring each polynomial below
• x2 16 9y2 1 4a2 9 16c4 81
• x2 42 (3y)2 12 (2a)2 32 (4c2)2 92
• (x 4)(x 4) (3y 1)(3y 1) (2a 3)(2a
  3) (4c2 9)(4c2 9)
• (4c2 9)(2c 3)(2c 3)

3
Factoring Polynomials

• The special pattern for factoring perfect square
  trinomials is a bit trickier, but it will be very
  important later on
• Learn to recognize the patterns for perfect
  square trinomials
• a2 2ab b2 ? (a b)2 or a2 2ab b2
  ? (a b)2
• The first and last terms are squared
• The middle term is twice the product of the
  square roots
• x2 6x 9 ? Try (x 3)2 ? v, 6x 2(x)(3) so
  (x 3)2 is right
• r2 8rs s2 ? Try (r s)2 ? v, 8rs ? 2(r)(s)
  so (r s)2 is wrong
• Try factoring each polynomial below
• x2 8x 16 9a2 6a 1 16c2 24c 25
• (x 4)2 ? (3a 1)2 ? (4c 5)2 ?
• 2(x)(4) 8x ? v 2(3a)(1) 6a ? v 2(4c)(5)
  24c ? No

4
Factoring Polynomials

• Some polynomials can be factored by grouping
  terms
• First, rewrite the polynomial to group terms with
  common factors
• 3xy 4 6x 2y ? 3xy 6x 2y 4
• Pull out the common factors in each group of
  terms
• 3xy 6x 2y 4 ? 3x(y 2) 2(y 2)
• Use the distributive property to rewrite the
  resulting expression
• 3x(y 2) 2(y 2) ? (3x 2)(y 2)
• Try factoring each polynomial below by grouping
  terms
• ax bx a b pq 2q 2p 4 4ab
  1 2a 2b
• x(a b) 1(a b) q(p 2) 2(p 2) 4ab
  2a 1 2b
• (x 1)(a b) (q 2)(p 2) 2a(2b
  1) 1(2b 1)
• (2a 1)(2b 1)

5
Factoring Polynomials

• Another pattern to recognize is the sum or
  difference of cubes
• Both terms of a binomial are cubes
• a3 b3 ? (a b)(a2 ab b2) Note the
  location of the sign
• a3 b3 ? (a b)(a2 ab b2) in both
  factorizations
• The binomial factor is the sum or difference of
  the terms being cubed
• The trinomial factor has their squares on either
  side
• The product in the middle has the opposite sign
  as the binomial
• Factor each polynomial below
• x3 8 (x 2)(x2 2x 4)
• y3 1 (y 1)(y2 y 1)
• 27z3 64 (3z 4)(9z2 12z 16)
• a6 b6 (a3 b3)(a3 b3) (a b)(a2 ab
  b2)(a b)(a2 ab b2)
• c6 d6 (c2 d2)(c4 c2d2 d4)
• r6 s6 (r2 s2)(r4 r2s2 s4) (r s)(r
  s)(r4 r2s2 s4)

6
Factoring Polynomials

• Always extract the greatest common monomial
  factor first, then see whether you can factor
  whatever is left
• 6x2 24 3x4 18x3 27x2 5x4 40x
• 6(x2 4) 3x2(x2 6x 9) 5x(x3 8)
• 6(x 2)(x 2) 3x2(x 3)2 5x(x 2)(x2 2x
  4)