Section 6.5 Conditional Probability Definitions

1
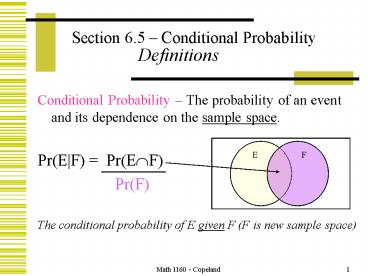
Section 6.5 Conditional ProbabilityDefinitions

• Conditional Probability The probability of an
  event and its dependence on the sample space.
• Pr(EF) Pr(E?F)
• Pr(F)
• The conditional probability of E given F (F is
  new sample space)

E
F
2
Section 6.5 Conditional ProbabilityExample

• Let E (had eggs for breakfast) and F (female) be
  events with Pr(E) .40, Pr(F) .30, and Pr(E ?
  F) .10
• Compute Pr(EF) Pr(E ? F) / Pr(F)
• 
  (.10) / (.30) 1/3
• Compute Pr(F/E) Pr(F ? E) / Pr(E)
• 
  (.10) / (.40) 1/4

3
Section 6.5 Conditional ProbabilityPage 312,
Problem 13a

• What is the probability
• that the person is not a
• democrat and opposes the
• school loan?
• Use the Venn diagram to find the region/s which
  correlate to D' ? F'. This part is NOT a
  conditional probability question.

.40
D
F
.30
.20
.10
4
Section 6.5 Conditional ProbabilityPage 312,
Problem 13b

• What is the conditional
• probability that the person
• favors the school loan given
• that he or she is a democrat?
• Pr(FD) Pr(F?D) .30 3 or .6
• Pr(D) .50 5

.40
D
F
.30
.20
.10
5
Section 6.5 Conditional ProbabilityPage 312,
Problem 13c

• What is the conditional
• probability that the person is
• a democrat given that he or
• she favors the school loan?
• Pr(DF) Pr(D?F) .30 3 or .75
• Pr(F) .40 4

.40
D
F
.30
.20
.10
6
Section 6.5 Conditional ProbabilitySample
Problems

• Pr(blond hair)? 60/140 Pr(female child
  brunette hair)? 10/60
• Pr(blond female)? 30/70 Pr(male child male)?
  20/70
• Pr(brunette adult)? 45/100 Pr(female brunette
  or red hair)? 40/80
• Pr(red hair child)? 10/40 Pr(male do not have
  red hair)? 60/120

7
Section 6.5 Independent EventsDefinitions

• Two events are independent if the occurrence of
  one has no effect on the likelihood that the
  other will occur.
• Pr(E?F) Pr(E) x Pr(F)
• OR
• Pr(E F) Pr(E)

8
Section 6.5 Independent EventsExample

• If E and F are two events such that Pr(E) .2,
  and the Pr(F) .36, and the Pr(E ? F) .09, are
  events E and F independent?
• Pr(E?F) Pr(E) x Pr(F)
• .09 ? .2 x .36

9
Section 6.5 Conditional ProbabilitySample
Problem

• A person tosses a coin 2 times. The sample space
  is HH, HT, TH, TT
• Event T a tail is thrown on the second toss
• Event H a head is thrown on the first toss
• Are events T and H independent?
• Pr(T?H) Pr(T) x Pr(H) OR Pr(T H) Pr(T)
• ¼ ½ x ½ OR ½ ½
  

10
Section 6.5 Conditional ProbabilitySample
Problems

• By examining the past driving records of 500
  randomly selected drivers over a period of 1
  year, the following data was obtained.

• Are events U and A independent?
• Are events U and N independent?
• Are events O and A independent?
• Are events O and N independent?