Section 6.4 Calculating Probabilities of Events Definition

1
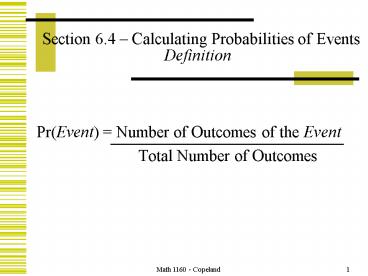
Section 6.4 Calculating Probabilities of
EventsDefinition

• Pr(Event) Number of Outcomes of the Event
• Total Number of Outcomes
  

2
Section 6.4 Calculating Probabilities of
Events Coin Tossing

• When tossing a coin 8 times, what is the
  probability of tossing exactly 6 tails?
• C( 8, 6 ) (6 tails in
  8 tosses)
• 28 (Total
  outcomes possible)
• 28 7
• 256 64

3
Section 6.4 Calculating Probabilities of
Events Having Children

• If a family has 8 children, what is the
  probability it has exactly 6 girls?
• C( 8, 6 ) (6 girls out
  of 8 children)
• 28 (Total
  outcomes possible)
• 28 7
• 256 64

4
Section 6.4 Calculating Probabilities of
Events Coin Tossing

• When tossing a coin 8 times, what is the
  probability of tossing at least 6 tails?
• C( 8, 6 ) C(8, 7) C(8, 8) (6 tails or 7
  tails or 8 tails)
• 28
  (Total outcomes possible)
• 37
• 256

5
Section 6.4 Calculating Probabilities of
Events Having Children

• If a family has 8 children, what is the
  probability it has at least 6 girls?
• C( 8, 6 ) C(8, 7) C(8, 8) (6 girls or 7
  girls or 8 girls)
• 28
  (Total outcomes possible)
• 37
• 256

6
Section 6.4 Calculating Probabilities of
EventsSample Problems

• Pr (blond hair)?
• Pr (female)?
• Pr (male and brunette)?
• Pr (male or brunette)?
• Pr (female and not blond)?

7
Section 6.4 Calculating Probabilities of
EventsPage 301, Problem 8

• Choose 2 white balls out of 6 Choose 2
  red balls out of 5
• C(6,2) x C(5,2) 150
  5
• C(11,4)
  330 11
• Total balls available (6 white 5 red)
  Sample Size

8
Section 6.4 Calculating Probabilities of
EventsThe Complement Rule

• The Complement Rule relates the probability
  of an event E to the probability of its
  complement E'.
• Pr(E) 1 Pr(E')
• It is beneficial to use this formula when
  calculating Pr(E') is easier than calculating
  Pr(E).

9
Section 6.4 Calculating Probabilities of
EventsPage 301, Problem 8 Extended

• The probability that at least one of the balls is
  red?
• (Calculate the complement ? all are white ?and
  subtract this value from 1)
• C(6,4) x C(5,0) 4 white balls and 0 red
  balls
• C(11,4) Combinations of 11
  taken 4 at a time
• 15 1 ? 1 (1/22)
  21/22
• 330 22