Collision Theory

1
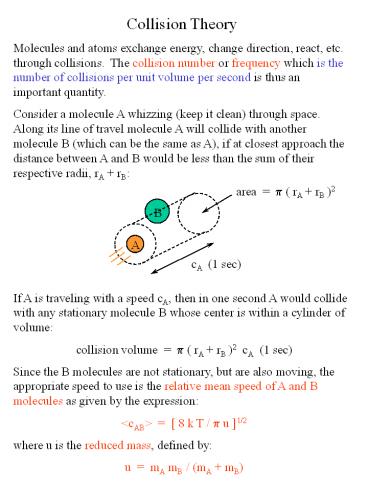
Collision Theory Molecules and atoms exchange
energy, change direction, react, etc. through
collisions. The collision number or frequency
which is the number of collisions per unit volume
per second is thus an important
quantity. Consider a molecule A whizzing (keep it
clean) through space. Along its line of travel
molecule A will collide with another molecule B
(which can be the same as A), if at closest
approach the distance between A and B would be
less than the sum of their respective radii, rA
rB
If A is traveling with a speed cA, then in one
second A would collide with any stationary
molecule B whose center is within a cylinder of
volume collision volume p ( rA rB )2 cA
(1 sec) Since the B molecules are not stationary,
but are also moving, the appropriate speed to use
is the relative mean speed of A and B molecules
as given by the expression ltcABgt 8 k T / p
u 1/2 where u is the reduced mass, defined
by u mA mB / (mA mB)
2
Multiplying this collision volume by the number
density of B molecules will give the total number
of collisions between a single A molecule and all
the B molecules present in the volume collision
volume (NB / V) p ( rA rB )2 ltcABgt (1
sec) (NB / V) Dividing this expression by the
volume and by the time interval of 1 second
will give zAB, the collision number for a single
A molecule, which is the total number of
collisions per unit volume per second between a
single A molecule and all the B molecules.
zAB collision volume (NB / V) (1 / V) (1 / 1
sec) p ( rA rB )2 ltcABgt (NB / V) (1 /
V) If the collision number for a single A
molecule is multiplied by all the A molecules
present we get the ZAB, the collision number for
A molecules colliding with B molecules, which is
the total number of collisions per unit volume
per second that all A molecules make with all B
molecules ZAB zAB NA p ( rA rB
)2 ltcABgt (NB / V) (NA / V) No2 p ( rA rB
)2 ltcABgt A B No2 p ( rA rB )2 8 k T
/ p u 1/2 A B Can you justify the steps in
this derivation? Note that in the above
expressions the lower case z in zAB implies the
number of collisions a single A molecule makes
with all B molecules, while the upper case Z in
ZAB implies the number of collisions all A
molecules makes with all B molecules, per unit
volume per second in both cases.
3
These expressions can be reduced even further, if
only A molecules are present. For zA, the
collision number for a single A molecule, which
is the total number of collisions per unit volume
per second between a single A molecule and all
the other A molecules we have zA p
(rArA)2 (8kT/(p (mAmA / (mAmA))))1/2 (NA/V)
(1/V) 25/2 p rA2 ( 8 k T / (p mA))1/2 (NA /
V) (1 / V) 25/2 p rA2 ltcAgt (NA / V) (1 /
V) In developing a similar expression for ZAA,
the collision number for A molecules colliding
with A molecules, which is the total number of
collisions per unit volume per second that all A
molecules make with all other A molecules, we
have to divide by 2 to avoid counting the
collisions twice. Derive an expression for ZAA.
4
The mean free path, l, is defined as the average
distance a molecule travels between collisions
and is calculated for molecules of a given type,
e.g., A, by dividing the molecules mean speed,
ltcAgt, or the distance the molecule would on
average travel in 1 sec by the collision number
for a single molecule times the volume of the
container, zA V, or the number of collisions that
a single A molecule would make on average with
other A molecules in 1 sec lA ltcAgt
/ (zA V) ltcAgt / ( 25/2 p rA2 ltcAgt ( NA / V )
) k T / ( 25/2 p rA2 P ) Could you justify
the steps in this derivation? In a closed
constant volume container how does the mean free
path vary with temperature? What is the mean
free path of O2 (g) molecules at 25.0 oC and
0.200 atm? The van der Waals radius for O2 (g)
is 0.188 nm.
5
Another useful quantity is Zsurface, the
collision number for molecules with a surface,
which is equal to the number of collisions of
molecules with the surface per unit area per
second. Consider a molecule in a rectangular box
traveling toward the right wall of area A with
component of speed in the x direction of cx
If this molecule is within a distance of cx times
1 sec of the right wall it will strike the wall
within 1 sec. Since in the collection of
molecules there is a distribution of speeds, on
average all molecules with a component of
velocity in the plus x direction will strike the
wall within 1 sec if they are within the
volume A (1 sec) ltcxgt A (1 sec) 0 ? oo cx P
(cx) A (1 sec) 0 ? oocx (m / 2 p
k T)1/2 e - m cx2 / 2 k T dcx A (1
sec) ( k T / 2 p m )1/2 In this derivation P
(cx) is the one-dimensional distribution of
molecular speeds or the probability that a
molecule has a speed between cx and cx dcx.
6
If the concentration of molecules is N / V, then
the number of molecules colliding with the
surface per unit area per second is Zsurface
(N / V) ( k T / 2 p m )1/2 P / ( 2 p m k T
)1/2 What happens to Zsurface as the
temperature of a gas in a closed constant volume
container is increased?
7
Calculate the average amount of time in seconds
that a water molecule exists on the liquid
surface of water in equilibrium with water vapor
at 25.0 oC. At 25.0 oC the equilibrium vapor
pressure of water is 23.76 mm Hg. Assume that a
water molecule at the liquid surface occupies an
area of 0.1000 nm2. Further assume that every
molecule of water vapor that collides with the
liquid surface enters the liquid phase.
8
Kinetic molecular theory can be used to develop a
molecular interpretation of pressure. Starting
with the ideal gas law P n R T / V ( n /
V ) ( R T )1/2 ( R T )1/2 and substituting a
result for the average molar energy of a gas,
ltEgt 3 /2 R T, derived from the assumptions of
kinetic molecular theory we get P ( n / V )
( R T )1/2 (2/3)1/2 ltEgt1/2 Now writing the
average molar energy in terms of the average
kinetic energy per molecule, ltEgt1/2 lt No (m c2
/ 2) gt1/2 gives P ( n / V ) ( R T )1/2
(2/3)1/2 lt No (m c2 / 2) gt1/2 multiplying by
(m/m)1/2 yields P ( n / V ) ( No R T / 3
m)1/2 lt (mc)2 gt1/2 Since lt(mc)2gt1/2 is the
root mean squared momentum, ltp2gt1/2, of the gas
molecules, we can use n N / No and R No k and
recognize that the pressure is P
( (N / No) / V ) ( No (No k) T / 3 m)1/2
lt(p)2gt1/2 (2 p / 3)1/2 (N / V) (k T / (2
p m))1/2 lt(p)2gt1/2 (2 p / 3)1/2 Zsurface
lt(p)2gt1/2 proportional to the product of the
average number of collisions per second with a
unit area of the container surface and the root
mean squared momentum of those collisions.