Some remarks about Lab 1 - PowerPoint PPT Presentation
1 / 29
Title:
Some remarks about Lab 1
Description:
Some remarks about Lab 1. image(parana.krige,val=sqrt(parana.krige$krige.var) ... likfit(parana,nugget=470,cov.model='gaussian',ini.cov.pars=c(5000,250) ... – PowerPoint PPT presentation
Number of Views:64
Avg rating:3.0/5.0
Title: Some remarks about Lab 1
1
Some remarks about Lab 1
2
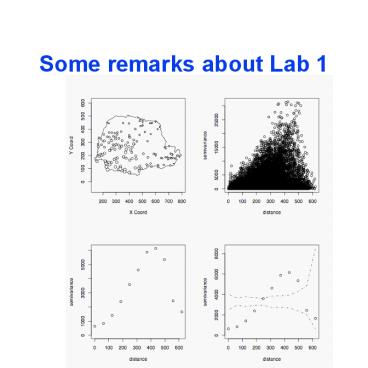
- image(parana.krige,valsqrt(parana.krigekrige.var
)) - contour(parana.krige,locloci,addT)
3
(No Transcript)
4
- likfit(parana,nugget470,cov.model"gaussian",ini.
cov.parsc(5000,250)) - kappa not used for the gaussian correlation
function - --------------------------------------------------
------- - likfit likelihood maximisation using the
function optim. - likfit estimated model parameters
- beta tausq sigmasq phi
- " 260.6" " 521.1" "6868.9" " 336.2"
- Practical Range with cor0.05 for asymptotic
range 16808.82 - likfit maximised log-likelihood -669.3
5
(No Transcript)
6
(No Transcript)
7
(No Transcript)
8
Nonstationary variance
- Let ?(x) be a Gaussian process with constant mean
?, constant variance ??????, and correlation
. - f is the same deformation as for the covariance
modelling. - Define the variance process
- Its distribution at gauged sites is
9
Moments of the variance process
- Mean
- Variance
- Covariance
- Correlation
10
Priors
- N(?,?)
- The full conditional distributions are then of
the same form (Gibbs sampler). - To set the hyperparameters we use an empirical
approach Let Sii be the sample variance at site
i.
11
Method of moments
- Setting the sample moments (over the sites) equal
to the theoretical moments we get - and let that be the prior mean. The prior
variance is set appropriately diffuse.
12
French precipitation
- Constant variance Nonconstant
- variance
13
Prediction vs estimation
- Leave out 8 stations, use remaining 31 for
estimation - Compute predictive distribution for the 8
stations - Plot observed variances (incl. nugget) vs.
estimated variances - and against predictive distribution
14
(No Transcript)
15
Estimated variance field
16
Global processes
- Problems such as global warming require modeling
of processes that take place on the globe (an
oriented sphere). - Optimal prediction of quantities such as global
mean temperature need models for global
covariances. - Note spherical covariances can take values in
-1,1not just imbedded in R3. - Also, stationarity and isotropy are identical
concepts on the sphere.
17
Isotropic covariances on the sphere
Isotropic covariances on a sphere are of the
form where p and q are directions, ?pq the angle
between them, and Pi the Legendre polynomials
18
Some examples
- Let ai?i, o?lt1. Then
- Let ai(2i1)ri. Then
- Given C(p,q)
19
Global temperature
- Global Historical Climatology Network 7280
stations with at least 10 years of data. Subset
with 839 stations with data 1950-1991 selected.
20
Isotropic correlations
21
Spherical deformation
- Need isotropic covariance model on transformation
of sphere/globe - Covariance structure on convex manifolds
- Simple option deform globe into another globe
- Alternative MRF approach
22
A class of global transformations
- Deformation of sphere g(g1,g2)
- latitude def
- longitude def
- Avoid crossing of latitudes or longitudes
- Poles are fixed points
- Equator can be fixed as well
23
Simple latitude deformation
knot
Iterated simple deformations
24
Two-dimensional deformation
- Let b and ? depend on longitude ?
- Alternating deform longitude and latitude.
location
scale
amplitude
25
Three iterations
26
Resulting isocovariance curves
27
Comparison
- Isotropic Anisotropic
28
Assessing uncertainty
29
Another current climate problem
- General circulation models require accurate
historical ocean surface temperature records - Data from buoys, ships, satellites