Chapter 6 Expected Value

1
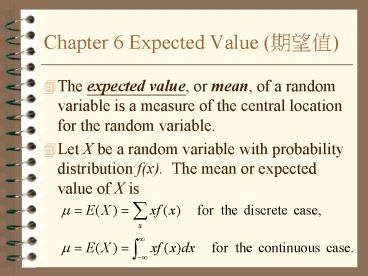
Chapter 6 Expected Value (???)

• The expected value, or mean, of a random variable
  is a measure of the central location for the
  random variable.
• Let X be a random variable with probability
  distribution f(x). The mean or expected value of
  X is

2
Example 6.1
3
Example 6.2

• Find the expected value of the density function
• Solution

4
Definition

• Let X be a random variable with probability
  distribution f(x). The mean or expected value of
  the random variable g(X) is

5
Note

• E(XY)E(X) E(Y)
• E(aXb)aE(X)b, where a,b is a constant.
• E(aX)aE(X)
• E(b)b
• If X and Y are two independent variables, then
  E(XY)E(X)E(Y)

6
Example 6.3

• Suppose that the number of cars, X, that pass
  through a car wash between 400pm and 500pm on
  any sunny Friday has the following probability
  distribution
• Let g(x)2x-1 represent the amount of money paid
  to the attendant. Find the expected earning of
  the attendant.

7
Solution 6.3
8
Definition

• Let X and Y be the random variables with joint
  probability distribution f(x,y). The mean or
  expected value of the random variable g(X,Y) is

9
Example 6.4
10
Note

• If g(X,Y)X, then we have

11
Note

• Similarly g(X,Y)Y, we have

12
Variance and Covariance

• When g(X)(x-m)2, the expected value Eg(X) is
  referred to as the variance of the random
  variable X. Is denote by Var(X) or s 2.
• The positive square root of the variance, s, is
  called standard deviation of X.

13
Definition

• Let X be a random variable with probability
  distribution f(x) and mean m. The variance of X
  is

14
Example 6.5

• Let the random variable X represent the number of
  defective parts for a machine when 3 parts are
  sampled from a production line and tested. The
  probability distribution is
• Find s2.

15
Solution 6.5
16
Theorem

• The variance of a random variable X is given by

17
Example 6.6
18
Note
19
Definition

• Let X and Y be the random variables with joint
  probability distribution f(x,y). The covariance
  of X and Y is

20
Theorem

• The covariance of two random variables X and Y
  with means mX and mY, respectively, is given by

21
Proof
22
Example 6.7

• The fraction X of male and the fraction Y of
  female numbers who complete marathon is described
  by the joint density function
• Find the covariance of X and Y.

23
Solution 6.7
24
Note