Theories of Solution

1
Theories of Solution
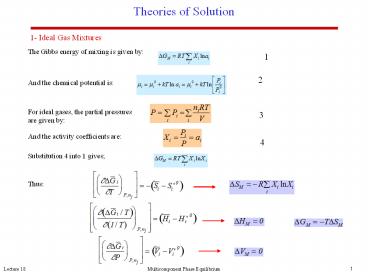
1- Ideal Gas Mixtures
The Gibbs energy of mixing is given by
1
2
And the chemical potential is
For ideal gases, the partial pressures are given
by
3
And the activity coefficients are
4
Substitution 4 into 1 gives
Thus
2
Ideal Gas Mixtures
?SM
?HM
?GM
XB
XB
0
XB
0
0
1
1
1
For systems with zero enthalpies of mixing, which
we generally call ideal mixtures, the entropy of
mixing completely determines G of mixing.
3
Ideal Gas Entropy of Mixing
For a binary ideal gas mixture we can plot the
entropy as a function of composition as shown on
the right (inunits of R).
The ideal entropy of mixing
In the case of a binary becomes
The slope of the entropy of mixing curve is
given by
This implies that it is impossible to completely
purify a material!
4
Raoults Law
If upon forming a mixture the partial pressures
of the vapor in equilibrium above the mixture is
such that
V
V
S
S
Then the pressure of the vapor will be a weighted
sum of the partial pressures, where the weights
are the mole fractions of each component
The solution is thus said to be Raoutian or Ideal
and P(Xi) for aRaoutian solution is plotted on
the right. Also note that for a Raoutian
solution
Since the activity and mole fraction are equal,
we have the same thermodynamics as the ideal gas
mixture. That is
5
Dilute Solutions
For a dilute solution, the Xi of one component is
very small, while Xi for the other component is
nearly one. In this case, the activity
coefficient ?i of the dilute component should be
composition independent since the components
environment is constant (it is surrounded by the
other component).
As the dilute component is added, the
probability of it having a like neighbor is
small and so its activity isconstant over a
range of dilute concentrations.
6
Dilute Solutions
Note that using the Gibbs Duhem equation for the
partial molar G, it can be shown that when B
obeys Henrys law, A obeys Raoults Law.
The infinitesimal change in the partial molar
Gibbs free energy
For A-B binary
Henrys Law for B dilute
Raoults Law for A
7
Excess Functions
Remember that
For an ideal solution
We define the excess function as the difference
between the actual value of the mixture and the
value for anideal mixture
8
Excess Functions
Lets take a closer look at the Gibbs free
energy of mixing usingthe concept of excess
mixing functions
The entropy of mixing is usually assumedto be
ideal so that the excess Gibbs free energyof
mixing is the excess enthalpy of mixing
The Gibbs free energy of mixing is then The
excess enthalpy of mixing minus T times the ideal
entropy of mixing.
9
Regular Solutions
The Regular Solution Model is a simple example of
a non-ideal solution.
Recall that for a mixture
The partial molar Gibbs free energyof mixing
(the difference betweencomponent is
contribution to G in the mixture versus pure i)
is relatedto the activity.
The Gibbs free energy of mixing is the weighted
sum of the contributions from each component.
The Gibbs free energy of mixingis then related
to the activities asshown.
In the ideal case the activities were just the
mole fractions
The excess Gibbs free energy of mixing is the
difference between the non-ideal and ideal G of
mixing
10
The Regular Solution Model
The Regular Binary Solution is defined as one
which has the following form for the
activity coefficients
Of course because the mole fraction of component
A is just one minus the mole fraction of B we
have
The excess Gibbs free energy of mixing is
And substituting the activity relationships for
the Regular solution gives
This can be manipulated to find
The excess Gibbs free energy of mixingof the
Regular Binary Solution.
11
Regular Solutions
The Gibbs free energy of mixing is the sum of the
excess and ideal Gibbs free energies of mixing
And substituting the Regular Solution excess G of
mixing
Notice that the first two terms are the negative
ideal entropy of mixing multiplied by T
Thus, the last term is the enthalpy of mixing
(and also the excess enthalpy of mixing since the
idealenthalpy of mixing is just zero)
The enthlapy of mixingof the Regular Binary
Solution with ? 10 J/mol.
12
Regular Solutions
Regular Solutions with ?10000J/mol.
13
Regular Solutions
Regular Solutions at T300K
14
Regular Solutions Atomistic Interpretation
The enthalpy of mixing is related to the
interactions between the atoms that make up the
mixture. If the solid has bond energies as
follows
EAA
EAB
EBB
The enthalpy of mixing is given by
Z is the coordination numberNT the total number
of atomsNA the number of A atoms
15
Regular Solutions Atomistic Interpretation
The enthalpy of mixing is determined as the sum
of the total interactions between the like
and unlike atoms in the mixture
Z is the coordination numberNT the total number
of atomsNA the number of A atoms
Then the enthalpy of mixing is
?A
?B
?B
?A(in A)
?B(in B)
16
Regular Solutions Atomistic Interpretation
Notice that the number of A atoms divided by the
total number of atoms is just the mole fraction
of A. Substituting in the mole fractions gives
This is the correct form for the Regular solution
where we make the definition
E
R
AA
The enthalpy of mixing of the Regular Binary
Solution is determined by the difference between
the AB bond energyand the average of the AA and
BB bond energies.
AB
BB
Various more complex models of solutions have
been developed with more complicatedexpressions
for the enthalpy of mixing, including next
nearest neighbor interactions, non-ideal
entropies of mixing, etc.
17
Solution of Defects
We could extend the principles of the
thermodynamics of mixtures between atoms to
mixtures between atoms and defects, such as
vacancies
Vacancies increase energy because they result in
broken bonds and the decrease in energy due to
the entropy they contribute from the uncertainty
of their placement in the solid.