Introduction to the Chain Rule

1
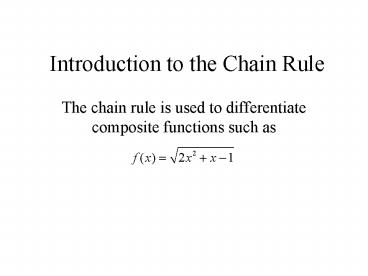
Introduction to the Chain Rule

• The chain rule is used to differentiate composite
  functions such as

2
Introduction to the Chain Rule

• Think of the composite function as the turning of
  a larger gear on a smaller one, and in turn that
  smaller gear has an effect on another, larger
  gear.
• When gear A makes x turns, gear B makes u turns,
  and gear C makes y turns. By counting the teeth
  on the gears, we might see, for example, that
  y2u and ux/3 so y2x/3. Thus dy/du2 and
  du/dx1/3 and dy/dx2/3(dy/du)(du/dx)

A x turns
C y turns
B u turns
3
Introduction to the Chain Rule

• To rephrase this a small change in x generates
  a small change in u which generates a small
  change in y, thus

Since dy/duf(u)_ and du/dx g(x), we can also
write
And substituting ug(x) we get
4
Course of Action on Using the Chain rule

• Identify which is the outer function and which is
  the inner function.
• The inner function, g(x) we now think of as u
• The outer function we call f(u)
• We can multiply the derivative of f(u) by u to
  find the derivative of f(g(x))

5
The Chain Rule

• To use the chain rule work from the outside to
  the inside. The formula says that we
  differentiate the outer function f at the inner
  function g(x) and then we multiply by the
  derivative of the inner function.

6
Practicing the Chain Rule

• Find the derivative of the following function

Try this using the chain rule, then check your
answer by expanding the polynomial and finding
the individual derivatives.
The outer function is The inner function is
7
Practicing the Chain Rule
8
Practicing the Chain Rule
9
Introduction to the Chain Rule
So if we apply this to our original problem, we
take the derivative of the outer function (dy/du)
and multiply this by the derivative of the inner
function (du/dx)