Free particle

1
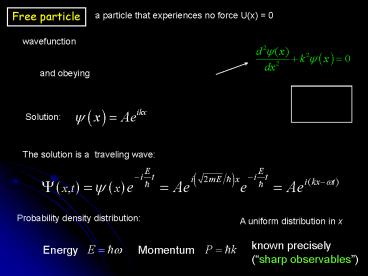
Free particle
a particle that experiences no force U(x) 0
wavefunction
and obeying
Solution
The solution is a traveling wave
Probability density distribution
A uniform distribution in x
known precisely (sharp observables)
Energy
Momentum
2
Example The average probability of finding the
particle per unit length along the x axis Assume
the particle is confined in the regime L ? x
? L
3
A particle in a box
a particle is confined in an infinite square well
A particle is confined in region 0 lt x lt L
E
Write
consider a particle having an energy E
Outside the well (x lt 0 or x gt L),
Inside the well (0 lt x lt L),
where
General solution
must be continuous at x 0 and x L
Requirement for wavefunction
4
Boundary conditions
Solution
Only discrete values are allowed !!
Energy quantization !!
n 1, 2, 3, . quantum number
wavefunction
The particle can never be at rest. E1 is the
zero-point energy
5
Spatial wavefunctions
n
?n
4 (1/2)L
3 (2/3)L
2 L
Quantization condition
1 2L
0
L
Cf. Standing waves on a string with fixed ends
Normalization
Full wavefunction
6
Probability density
For the nth quantum state, has n
maxima and n1 zeros
Pn(x)
7
Hydrogen atom
Particle in an infinite well
8
A particle in a finite square well
Consider a potential well with a finitely high
Finite Square Well and E lt U0 Introduces
the very important concept of barrier
penetration
x
Region II
Region I, III

• Need to solve Schrodinger wave equation in
  regions I, II, and III

9
Region II same as infinite square well U(x)
0 Solution
10
Regions I and III U(x) Uo
These regions are forbidden to classical
particles. E lt U0

Write the Schrodinger equation as
where
The general solution to this equation is
Region I
exponential solutions
Region III
(C1, C2, D1, and D2 to be determined from
boundary conditions)
11
For quantum particles, there is a finite
probability amplitude, ?, for finding the
particle inside a classically-forbidden region,
i.e., inside a barrier
12
As x ? ?, the wavefunction must vanish.
C2 0 and D1 0
Summarizing the solutions in the 3 regions
Region I
? gt 0
Region II
Region III
? must change smoothly across boundaries, i.e.,
both ? and its derivative must be continuous at
boundaries
Boundary conditions
and
At x 0
and
At x L
13
Finite square Well
Infinite square Well
14
y(x)
The wavefunctions look very similar to those for
the infinite square well except the particle has
a finite probability of leaking out of the well
Unlike classical particles !
Penetration depth
Allowed energies in the well
15
The fact that ? is nonzero at the walls
increases the de Broglie wavelength in the well
? lowers the energy and momentum of the particle
Cf, in the limit U0 ? ? (an infinite potential
well)
e.g., for an electron with U0 E 1 eV ? ?
0.2 nm
e.g, estimate the value of En for an electron in
a 1-nm box
(11/23/2009)
16
A particle in a harmonic oscillator potential
Simple harmonic oscillators describe many
physical situations springs, diatomic molecules,
atomic lattices, etc.
U??
U??
Substituting this potential energy into
Schrödinger equation
?
? 2
17
The wavefunction are
Hn(x) Hermite polynomials of order n
18
Substituting the ground-state wavefunction
into the Schrodinger equation
One finds
The particle can never be at rest !!
Similarly,
n 0, 1, 2, 3,
There is considerable penetration of the wave
into the classically forbidden regions. A
detailed analysis shows that the particle can be
found in these nonclassical regions about 16 of
the time
19
The energy is quantized !
bound states
n 0 Ground state zero point energy
20
Probability densities for a few states of the
quantum oscillator. The dashed curves represent
the classical probabilities corresponding to the
same energies
21
SUMMARY For a given potential, such as an
infinite square well, a finite square well and a
harmonic oscillator potential, The energy E ( lt
U ) is quantized There is a unique and specific
wavefunction associated with each allowed
energy Each state is labeled by a quantum number
n There is a small, but finite, probability for
the wavefunction to penetrate into the
classically forbidden regime (except for the case
of an infinite square well)
22
Expectation values
For a given wavefunction ?(x,t ), there are two
types of measurable quantities The energy E
for a stationary state is fixed by the quantum
number n labeling the wave. Every measurement
of E performed on the system described by ?
yields the same value The wavefunction ?
furnishes only the probabilities (average values)
of the position x of the particle
U(x,t) U(x)
quantum uncertainty in position ?
23
The average particle position
24
The measured values scatter about the average
value. The amount of scatter is measured by the
standard deviation
N the no. of data points
25
Expectation values
Probability density
wavefunction
The wavefunction can be used to find the
expectation value of any dynamical quantity
Two important things we can determine are
expectation values and uncertainties
Mathematically, an expectation value is an average
The average value of x is given by
Expectation value may be a function of time
except for stationary states
Average or expectation value for any function of x
26
Uncertainty
We define it as the standard deviation from
average
Quantum uncertainty in position
27
Example Location of a particle in an infinite
square well
28
Example. A particle in an infinite square well
Expectation value for position x
29
Quantum uncertainty in position
30
Average or expectation value for any function of
momentum P
The order of terms is of essential importance !!
??
Average value of the square of momentum P
Uncertainty in momentum
31
Observables and Operators (??)
An observable is any particle property that can
be measured
In quantum mechanics, an operator refers to
operation to be performed on whatever function
follows the operator Using this operator one can
calculate the average value of the corresponding
observable
Operator ? related observable
Operator Q operates on a wavefunction
Expectation value ?Q? predicts the average
value for Q
32
Physical property
symbol
Operator
1D system
x
position
r
r
P
momentum
Kinetic energy
KE
Potential energy
U
Hamiltonian
H
Total energy
E
33
Average potential energy
Average kinetic energy
Average total energy
Schrödinger equation
Hamiltonian
Energy
(11/30/2009)
34
For any observable
The quantum uncertainty
In general, ?Q gt 0 ? the observable is fuzzy
When ?Q 0 ? Q is a sharp observable
implying
q is a constant !!
For the operator Q, ?(x, t) is an
eigenfunction and q is an eigenvalue
35
Back to free particle
A plane wave is used to describe the quantum
state of a free particle
?P 0
?x ? ?
?t ? ?
?E 0
How about Heisenberg uncertainty principle ?
36
Infinite square potential revisited
(eigenfunction)
a constant (eigenvalue)
Definite a sharp observable for H with
uncertainty in energy ?E 0
37
Other observables
Position operator x
Momentum operator P
a spread and fuzzy observable
Both position and momentum have predicted values,
but uncertainties are non-zero as well
38
Correspondence Principle
Correspondence between classical and quantum
regimes for large n
Ball in a box
quantum
U(x)
E
x
Quantum particle is also distributed with equal
probability inside the box
Probability is equally distributed
39
Correspondence Principle
Correspondence between classical and quantum
regimes for large quantum number n
classical
Simple Harmonic Oscillator
Total energy E KE U(x)
Quantum particle is also more likely to be found
at the edges
More likely to spend time at the edges
40
SUMMARY Schrodinger equation Time-independent
Schrodinger equation Infinite and finite
square wells, simple harmonic oscillators Observa
bles, Operators, Expectation values, Eigenvalues
(12/2/2009)