1.3 Evaluating Limits Analytically

1
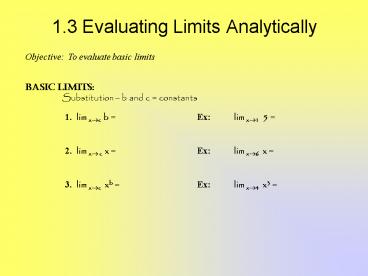
1.3 Evaluating Limits Analytically
Objective To evaluate basic limits
Basic Limits Substitution b and c constants
1. lim x?c b
Ex lim x?1 5
2. lim x? c x
Ex lim x?6 x
3. lim x?c xb
Ex lim x?4 x3
2

• Properties of Limits
• Scalar Multiplication limx?cb f(x) b
  limx?cf(x)
• Ex limx?2 3x 3 limx?2 x

• Sum/Difference - limx?c f(x) g(x) limx?c f(x)
  limx?c g(x)
• Ex limx?4 x2 x limx?4 x2 limx?4 x

• Product - limx?c f(x) g(x) f(c) g(c)
• Ex limx?p x2 cos x

3

• Powers limx?cf(x)b f(c)b
• Ex limx?34x2

• Polynomials limx?cP(x) P(c)
• Ex limx?4 2x2 x 5

Basic Trigonometric Limits
limx?c sin x sin c
4
Given limx?c f(x) 3 and limx?c g(x) -5
evaluate the following. ? limx?c 4 f(x) ?
limx?c f(x)g(x) ? limx?c g(x)3 ? limx?c
f(x) g(x) ? limx?c f(x)/g(x)
5
Last Page!!!!!
Note You can only drop limx???c after you
substitute in the value of c.
Assignment 1.3 day one this is on line 2.
We will have a short quiz over the graphs on
Tuesday.
6
1.3b More Basic Limits

• Limit Quiz 1

7
Questions from 1.3a??
Objective To evaluate different instances of
the indeterminate form of a limit.

• If it is a rational function factor, cancel and
  try the limit again.

8
? ? Simplify the fraction
9
Why does this work?????
10
The cosine limit that you need to know is
There is no reciprocal here since this equals 0.
11
Now try these
Assign 1.3b 41-53 odd, 54, 56, 57-67 odd, 68,
69, 71, 85
12
Anton Limits Worksheet

• Objective to cover more computational methods
  of limits including limits at infinity.

13
Anton Worksheet Day 1

• Questions
• Quiz after Anton and Review Worksheets

Review limx?a f(x) Is the function defined at
a? YES Use substitution NO /0 asymptote
dne 0/0 or ?/? ? Factor, cancel, try the
limit again ? Rationalize the
numerator/denominator ?Simplify complex
fractions ? Basic trigonometric properties
14
Limits at ? ? Use left/right behavior from
graphing rational functions.
15
Radicals at ? ?
16
2 more radicals ?
NOTE ? - ? is also an indeterminate form and
does not 0!!
17
What about piecewise functions?
Recall limx?c f(x) limx?c- f(x) L for the
limit to exist.?

• lim x?2 f(x)
• lim x?5 f(x)
• lim x?-4 f(x)

If each branch is continuous, a limit will exist
at every point on each branch. The only issue
you will need to check is the value where the
branches meet.
18
One more piecewise
?Lim x?-1 g(x) ?Lim x?-5 g(x) ?Lim x?-4 g(x)

Assign Anton day 1 1-23 odd and 24-47 all
(try all of the new ones)
19
Anton Worksheet Day 2
Questions??
What if you get ? - ? ???
20
Equations that agree at all but one point.
Dont forget you must have lim x?c on each step
until you substitute in the value of c and get
your answer. Otherwise, it will be one point off
per problem on the quiz/test.
21
Quiz Review
Limits ? from graphs ? definition of a
limit ? discuss when limits do not exist and
why! ? evaluate limits as x ?c and x??

• Substitution
• Factor, cancel and try again
• Rationalize the numerator/denominator
• Simplify complex fractions
• Trig
• Piecewise
• Limits at ?

22
(No Transcript)
23
1.4 Continuity

• Objective To understand the conditions for
  which a function is continuous and to identify
  the different types of discontinuities

24
A continuous curve can be sketched without
lifting your pencil
continuity uninterrupted flow of a graph from
left to right
Imagine an equations graph as a pipe full of
water? If the water can flow continually
through the pipe across the entire coordinate
plane without leaking or spilling, the graph is
continuous.
In order for a graph to be continuous at a
specified point c, the following 3 conditions
must ALL be met        
1. 2.3.  
Memorize these!
For the entire equation to be considered
continuous, these conditions must be met for
every x value.
25
Types of Discontinuities Removable
Holes typically caused by a denominator being
equal to zero you are able to cancel the factor
causing the problem using algebra.
condition violated f (c) is NOT defined
Removable
26
Types of Discontinuities Removable
Redefined holes usually result from a piecewise
function where the equation follows one rule for
every value except one at that one value, the
function is redefined in a place other than the
hole left by the rest of the graph.
Removable
The only type of removable discontinuity is the
hole. ?
27
Types of Discontinuities Non-removable
Asymptotes typically caused by a denominator
being equal to zero and you are unable to cancel
the factor causing the problem.
condition violated f (c) is NOT defined
E.G. is discontinuous at x c.
Non-removable.
28
Types of Discontinuities Non-removable
Undefined regions caused by certain x values
not being within the domain of the equation
condition violated f (c) is NOT defined
NOTE You can say that a graph is continuous for
all x values within a certain region. (In the
e.g., you can say the function is continuous for
x 0.)
Nonremovable
29
Types of Discontinuities Non-removable
Jumps usually result from a piecewise function
where the two pieces do not join together.
NOTE If the parts of the function are
continuous, the place in question is where the
function separates (in this case, at x 0).
Nonremovable
30
Continuity cont.
Also ? Polynomials are continuous everywhere.
(Why?) ? Rational functions are continuous
everywhere the denominator does not
equal 0. ? Radicals are continuous everywhere
the radicand 0. ? Sine and cosine curves are
continuous everywhere.
31

• Discuss the continuity of the function.
• 1. Give any x values where the function is
  discontinuous
• state the condition for continuity which is
  violated at that point
• describe what is happening on the graph at each
  point and
• state whether each continuity is removable or
  non-removable.

32
Summary

• 1. A discontinuity is REMOVABLE (hole) if
  the limit exists and it fails one of the
  other 2 conditions
• The hole may or may not be redefined
• A discontinuity is NON-REMOVABLE if it is a jump,
  asymptote, or out of the domain of the function.
• For piecewise functions, determine continuity on
  each branch and where the branches meet. (or do
  not meet.)
• A function is continuous at x c if

Assign 1.4a 5-23 odd, 12, 14, 27-30, 31-39 odd
33
1.4b Intermediate Value Theorem
Objective To understand and use the
IVT Questions from 1.4a???????
The IVT is an existence theorem. It will prove
that a value exists, but not how to find the
value. We will use this for future proofs.
IVT If f(x) is continuous on a, b and k is any
y-value between f(a) and f(b), then there exists
at least one x c such that f(c) k.
So to get from f(a) to f(b) you must cross y k.
What would it mean if f(a) and f(b) have opposite
signs??????
34
Bottom line ? all y values between f(a) and f(b)
exist.
Find the value guaranteed by this theorem for y
0.
35
Verify the IVT applies and find all values
guaranteed by the theorem.
MUST1. State f(x) is continuous on a, b.2.
Find f(a) and f(b)3. Find x c such that f(c)k
Ex1) f(x) x2 2x 1, 4 ? k 8
1-4b 41-45 odd, 52, 55-58, 61, 67-71, 73, 74,
75, 77, 79-82, 91,93