Water balance and evapotranspiration

1
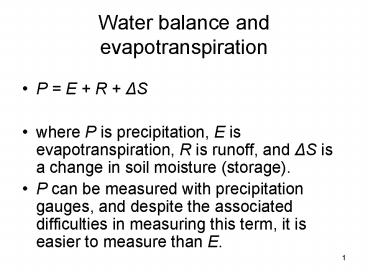
Water balance and evapotranspiration

• P E R ?S
• where P is precipitation, E is evapotranspiration,
  R is runoff, and ?S is a change in soil moisture
  (storage).
• P can be measured with precipitation gauges, and
  despite the associated difficulties in measuring
  this term, it is easier to measure than E.

2

• E combines evaporation from soil and water
  surfaces with transpiration from plants and
  trees.
• It can be measured using eddy correlations or
  profile approaches or estimated in a number of
  ways.
• Why is it important to find the water balance?
• water resources (hydro, irrigation, etc.)
• soil moisture (trees and plants, forest fires,
  etc.)

3

• Evapotranspiration also links the surface energy
  and water balances since QE LvE
• Recall that Q QH QG QE
• This can therefore be written as
• Q QH QG LvE
• Thus through this term, the surface energy and
  water budgets are intimately linked.

4
Oke (1987)
5
Déry et al. (2005)
6
Water budget of the Kuparuk River Basin
Déry et al. (2005)
7
Components of the water budget
8
Energy and Mass Exchanges

• Electricity analog to convective exchanges Ref
  Oke, pp. 70-71
• Flow of electricity in an electric circuit is an
  analogy to convective fluxes.
• Ohms law states that
• current (amps) potential difference (V)/ wire
  resistance (ohms)
• flux rate (concentration difference)/(resistanc
  e to flow)

9

• t - ?a ?u/ram
• QH - Ca KH ?T/raH
• E ??v/ raV
• FC ??c/ raC

10

• where r represents the system resistance (s m-1),
  and r-1 is called the conductance.
• Note that r is like 1/K in flux gradient
  relationships.
• Resistance represents a hindrance to flow.
• This analogy can simplify some calculations and
  helps meteorologists and engineers because all
  are familiar with it.

11

• Resistances are added either in series, i.e. r
  r1 r2, such as flow through a number of layers
  (two in this case), or in parallel such as
• 1/r 1/r1 1/r2

12
Combination model (Penman - Monteith) for
evapotranspiration

• Ref Oke, pp. 384-388
• This approach combines aerodynamic and energy
  balance principles to find evapotranspiration.
• The vapour gradient driving surface
  evapotranspiration from a saturated surface
  (relax this assumption later) is ?v0 - ?va

13

• where ?v0 is the saturation vapour density at
  the surface and ?va is the vapour density in the
  air above it.
• Thus QE Lv (?v0 - ?va)/rav LvE
• where rav is the aerodynamic resistance to water
  vapour transport.
• The key issue then is to find (?v0 - ?va) and
  rav (hopefully using simple observations).

14
Oke (1987)
15

• (?v0 - ?va) has two parts, one due to (?v0 -
  ?va) and the other due to (?va - ?va) wvdd
  (water vapour density deficit).
• By linearizing the ? vs T curve
• (?v0 - ?va) S (T0 - Ta) wvdda
• so, for a saturated surface
• QE (Lv S (T0 - Ta) Lv wvdda) / raV
• But QH Ca (T0 - Ta)/raH

16

• T0 - Ta QH raHCa
• Thus QE (Lv S QH raH)/(Ca raV) (Lv wvdda)/raV
• Before proceeding, let us define the psychrometer
  constant ? Ca/Lv and let us assume similarity
  between raH and raV.
• Recall also that QH Q - QG - QE
• QE S/ ? (Q - QG - QE) (Cawvdda/raH)/ ? 1
• This can be re-arranged to solve for QE

17

• QE S/ (S?) (Q - QG) (Cawvdda/raH)/ (S?)
• This is called the combination model for a
  saturated surface.
• The first term on the rhs is an energy term
  depending only on the temperature and energy
  available, whereas the second term is an
  advection or vapour deficit term combining
  dryness with aerodynamic resistance.
• Most of the terms in the Penman model are easily
  measured raH is slightly more challenging

18

• QH Ca (T0 - Ta)/raH -Ca ?2 z2 (?u/?z)(?T/?z)
• Solving for raH
• raH (T0 - Ta)/(?2 z2 ?u/?z)(?T/?z)
• And using the log mean height z (z2 -
  z1)/ln(z2/z1) then
• raH (ln(z2/z1))2/ ?2 ?u
• Therefore need wind speeds at two heights.
• In stable/unstable situations, need to make
  stability corrections.
• Assumes similarity.

19

• If air becomes saturated (e.g. over oceans) then
  wvdda 0 and
• QE S(Q - QG) /(S ?)
• This is called the equilibrium
  evapotranspiration rate.
• Over partly saturated surfaces, we modify
  combination model by using surface water vapour
  density deficit wvdd0 (?v0 - ?v0).

20

• QE S(Q - QG) Ca(wvdda - wvdd0)/raH/ (S
  ?)
• The moisture flux through the canopy (stomatal,
  etc.) is given by
• QE Lv wvdd0/rc
• where rc is the canopy resistance.
• If you solve here for wvdd0 and substitute into
  the equation above, recognizing that ? Ca/Lv
  then

21

• QE S(Q - QG) (Cawvdda )/raH/ (S ?)(1
  rc/raH)
• This is called the Penman-Monteith model.
• The difficulty is in the evaluation of canopy
  resistance rc (resistance to transpiration via
  stomatal averages for the entire canopy).

22
Clausius-Clapeyron Relationship
Stull (2000)
23
Stull (2000)