Distance in Coordinate Geometry

1
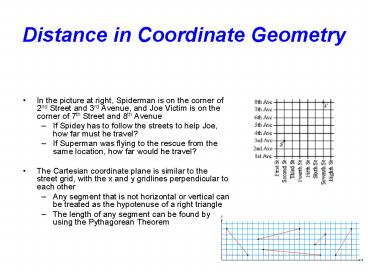
Distance in Coordinate Geometry

• In the picture at right, Spiderman is on the
  corner of 2nd Street and 3rd Avenue, and Joe
  Victim is on the corner of 7th Street and 8th
  Avenue
• If Spidey has to follow the streets to help Joe,
  how far must he travel?
• If Superman was flying to the rescue from the
  same location, how far would he travel?
• The Cartesian coordinate plane is similar to the
  street grid, with the x and y gridlines
  perpendicular to each other
• Any segment that is not horizontal or vertical
  can be treated as the hypotenuse of a right
  triangle
• The length of any segment can be found by using
  the Pythagorean Theorem

2
Distance in Coordinate Geometry

• The worksheet for this section contains a few
  practice problems finding distances on a
  coordinate plane
• In problem 1, find the distance between the
  plotted points
• In problem 2, plot the points and find the
  distance between them
• In problem 3, find the distance without plotting
  the points
• Generalize the approach in problem 3 to apply to
  any pair of points
• Let the coordinates of the points be A(x1, y1)
  and B(x2, y2)
• C-86 Distance Formula
• The distance between points A(x1, y1) and B(x2,
  y2) is given by (AB)2 (x2 x1)2 (y2
  y1)2 or AB v(x2 x1)2 (y2 y1)2

3
Distance in Coordinate Geometry

• The distance formula can be applied to find the
  equation for graphing a circle on a coordinate
  plane
• Let the center of the circle be at point C(h, k)
• Let (x, y) be any point on the circle
• The distance from point C to any point on the
  circle is the radius r of the circle
• The distance r can be found by substituting the
  variables x, y, h, and k into the distance
  formula
• The result is the general form for the equation
  of a circle
• C-87 Equation of a Circle
• The equation of a circle with radius r and
  center (h, k) is (x h)2 (y k)2  r 2

4
Distance in Coordinate Geometry

• Example 1 Using the Distance Formula
• Find the distance between points A(8, 15) and
  B(-7, 23)
• Plug the values into the distance formula (AB)2
   (x2 x1)2 ( y2 y1)2
• Evaluate the resulting equation (AB)2 (-7
  8)2 (23 15)2
• (AB)2 (-15)2 (8)2
• (AB)2 289
• AB 17, so the distance between the two points
  is 17 units
• Example 2 Using the Equation of a Circle
• Find the center and radius of the circle (x 2)2
  (y - 5)2 36
• Use the standard form for a circle (x h)2 (y
  k)2  r 2
• Rewrite the equation to fit the standard form (x
  (-2))2 (y - 5)2 62
• Identify h, k, and r
• The center is at (-2, 5) and the radius is 6