Modelling cohesive sediment transport Lecture 24

1
Modelling cohesive sediment transportLecture 24

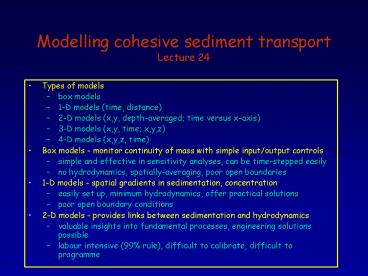
• Types of models
• box models
• 1-D models (time, distance)
• 2-D models (x,y, depth-averaged time versus
  x-axis)
• 3-D models (x,y, time x,y,z)
• 4-D models (x,y,z, time)
• Box models - monitor continuity of mass with
  simple input/output controls
• simple and effective in sensitivity analyses, can
  be time-stepped easily
• no hydrodynamics, spatially-averaging, poor open
  boundaries
• 1-D models - spatial gradients in sedimentation,
  concentration
• easily set up, minimum hydrodynamics, offer
  practical solutions
• poor open boundary conditions
• 2-D models - provides links between sedimentation
  and hydrodynamics
• valuable insights into fundamental processes,
  engineering solutions possible
• labour intensive (99 rule), difficult to
  calibrate, difficult to programme

2
creation of hypotheses formulation of scientific
questions
3
RALPH time-series
Sector scanning sonar imagery
Sedimentary peels from Box cores
Swath survey on Sable Island Bank
4
SEDTRANSA 0-D sediment transport numerical model

• ANSI standard Fortran programme
• 1-dimensional, interactive or batch mode
• cohesive or non-cohesive solutions
• steady currents
• wave motion
• waves and currents combined
• immediate output (screen and file)

5
SEDFLUX 3-D simulation

• Syvitski et al. Hwang Ho delta

• links to 3-D seismics lab facility

6
Fundamental equations

• Sediment continuity equation (3-D)
• Mass continuity equation (3-D) (Exner)
• where D is net deposition, E is net erosion, S is
  suspended sediment concentration, e is sediment
  porosity, ?b is sediment unit weight, h is bed
  elevation, Ws is mean settling rate, and U,V,W
  are the three components of mean flow. We can add
  external sources or sinks to the above by adding
  terms M1.Mx as follows..

(Closed system)
(Open to the bed)
7
Instrumentation development to calibrate
geodynamic models

• acoustical
• optical
• electro-magnetic/peizo-electric
• x-ray

• benthic landers (RALPH)
• benthic flumes (Sea Carousel)
• remote sensing tools (backscatter)
• self-contained packages (Zedhead)

Sediment concentration gradients (3-D) (6)
Velocity field (3-D) (2)
Mass settling rate (3)
time-series, usually from fixed location (1)
External sources/sinks (5)
Benthic flux (4)
The sediment continuity equation sediment budget
8
Morphological-dynamics and sediment dynamics
the links to Engineering

• (5i) Deposition (D) from cores (Pb210 or Cs137)
  or sediment traps or altimeter measures
• (5ii) Erosion (E) (closed systems) or acoustic
  profiling or long-term swath bathymetry
• (6) Sediment traps, geophysical surveys of
  bedform migration

(1) only possible in closed system !!!
(mesocosm) (2) bottom sampling (3) acoustic
velocity, resistivity, CT scanning (4) acoustic
altimeter, 2-axis sector scanning sonar,
repetitive swath bathymetry
Time-series of bed level change (4)
Benthic flux (1)
Sediment density (2)
Porosity (3)
Divergence in bedload transport rate (6)
Deposition(D) Erosion(E) (5)
The Exner equation
9
A box model for Cumberland Basin, Canada

• Cumberland Basin (upper Bay of Fundy)
• source terms
• river input versus time
• seabed erosion
• diffusion/advection from Chignecto B.
• ice breakup
• sink terms
• seabed sedimentation
• freeze-up
• diffusion/advection to Chignecto B.
• Output concentration (S) and mass balance (Tb)

Amos and Tee, 1989. J. Geophys. Res. 94(C10)
14,407-14,417
10
The governing equations

• Governing equations (1-D and box)
• calibration data - spatially integrated
  measurements of S with time
• Tb is measured independently (total suspended
  mass)

Mr - river influx Ms - sedimentation Me -
advective flux Mi - ice effects Md - diffusive
flux
11

• k - sediment concentration (S) decay constant
  along estuary (x) over time (t)

12
Sensitivity analysis of sediment flux rates at
open boundary

• S - sediment concentration dominates
• flux increases as S increases
• Ws - flux increases with increased settling rate
• Ud - deposition threshold
• flux increases as threshold increases
• Ue - erosion threshold
• flux increases as threshold increases
• NOTE S dominates the signal so should be the
  focus of measurement, input and calibration in
  model

13
The open boundary problem

• The open boundary offers the greatest difficulty
  to measure and to predict accurately
• note the change from importing to exporting with
  time
• import and export linked to S and more
  importantly to S(x)
• an estuary can change trapping efficiency
  depending on S gradient
• caused by wave resuspension
• dredging
• tidal variations
• construction, etc