Trigonometry: Graphing Application - PowerPoint PPT Presentation
1 / 8
Title:
Trigonometry: Graphing Application
Description:
In a certain national park the population of rabbits, R, varies sinusoidally as ... The voltage, E, for an electrical current is given by where t is time in seconds. ... – PowerPoint PPT presentation
Number of Views:126
Avg rating:3.0/5.0
Title: Trigonometry: Graphing Application
1
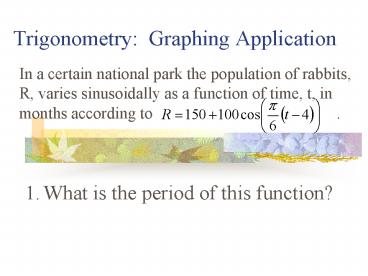
Trigonometry Graphing Application
- In a certain national park the population of
rabbits, R, varies sinusoidally as a function of
time, t, in months according to
.
- What is the period of this function?
2
Trigonometry Graphing Application
- In a certain national park the population of
rabbits, R, varies sinusoidally as a function of
time, t, in months according to
.
- What is the maximum number of
- rabbits in the park during the year?
3
Trigonometry Graphing Application
- In a certain national park the population of
rabbits, R, varies sinusoidally as a function of
time, t, in months according to
.
- During what month does this
- max occur? Why?
4
Trigonometry Graphing Application
- In a certain national park the population of
rabbits, R, varies sinusoidally as a function of
time, t, in months according to
.
4. What is the minimum number of rabbits in
the park during the year?
5
Trigonometry Graphing Application
- In a certain national park the population of
rabbits, R, varies sinusoidally as a function of
time, t, in months according to
.
5. During what month does this min occur?
Why?
6
Trigonometry Graphing Application
- The voltage, E, for an electrical current is
given by
where t is time in seconds.
- What is the amplitude of this
- function? Include units.
7
Trigonometry Graphing Application
- The voltage, E, for an electrical current is
given by
where t is time in seconds.
2. What is the period of this function?
Include units.
8
Trigonometry Graphing Application
- The voltage, E, for an electrical current is
given by
where t is time in seconds.
3. What is the frequency of this
function? Include units.