Last Time

1
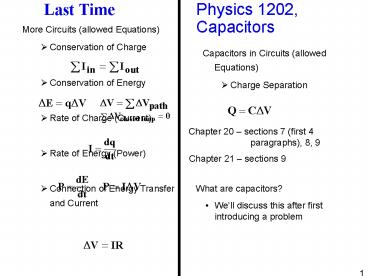
Last Time
Physics 1202, Capacitors

• More Circuits (allowed Equations)
• Conservation of Charge
• Conservation of Energy
• Rate of Charge (Current)
• Rate of Energy (Power)
• Connection of Energy Transfer and Current

• Capacitors in Circuits (allowed Equations)
• Charge Separation

Chapter 20 sections 7 (first 4 paragraphs), 8, 9
Chapter 21 sections 9

• What are capacitors?
• Well discuss this after first introducing a
  problem

2
First a Quick Review of Concepts
A capacitor is a device that stores charges,
seperated by some distance. In other words, it
stores energy.
A Force exerted over a distance
Energy
Examples

• Apply idea to charges
• Charges exert forces on each other. Farther
  away, less force
• Likes Repel. Unlikes Attract

- - - - -
Parallel Plate Capacitor

Outside axon cell
- - - - -

Axon
Inside axon cell

- - - - -
Not much energy here
Outside axon cell
Cell Membrane
A force is required to separate charges gt Energy
is stored. Check idea If we release the
charges, they will increase in velocity toward
each other P.E. converted to K.E.
Since the TOTAL charge on a capacitor is usually
zero, when we discuss charge on a capacitor we
are usually referring to the total amount of
extra charge.
3
Energy is related to Voltage
Call that propotionality constant something
special C gt capacitance.
There is a difference in voltage from () side of
the capacitor to the (-) side. So, lets try to
quantify capacitors in terms of voltage and how
much charge they have. More charge more
stored energy more voltage
CDV Q
Units of Capacitance?

• For the graphs shown, which has the largest
  capacitance?
• Capacitor A
• Capacitor B
• Charge and Voltage information are needed

Experimentally, we find that charge and voltage
for a capacitor are proportional
DV
Capacitor B
Capacitor A
Q
4
Lecture Question The charge on a capacitor is
doubled. What happens to the capacitance of that
capacitor?

• It stays the same
• It increases
• It decreases
• Information about the voltage is necessary to
  answer this question

5
Capacitors in Ciruits
Whats going on? Apply your knowledge of
physics to this situation.
What do we know about the behavior of a capacitor?
Put a single capacitor in a simple electrical
circuit.
Conservation of Charge
qf qi qin - qout
Current
Conservation of Energy
Ef Ei Ein - Eout
Close the switch.
What do you observe?
Voltage difference
Power
Can you explain it?
For a resistor DV IR
6
Explanation
Example
You are to evaluating the design of a portable
device to revive heart attack victims. The design
uses a battery as the energy source but needs to
deliver a voltage difference much larger than
that of the battery. The device works by first
charging a set of identical capacitors that are
each connected across the terminals of a battery.
When the capacitors are fully charged, a
mechanism disconnects them from the battery and
then connects them together so that each
positively charged plate is connected to a
negatively charged plate. The manufacturer
claims that the left over ends of the capacitor
chain have a voltage difference larger than that
of the battery. To see if this is true you
decide to calculate the case of 3 capacitors all
with the same capacitance and compare the
resulting voltage difference to that of the
battery.
Conservation of Charge
One side gets more negative.
One side gets more positive.
Bulb lights
Total charge of capacitor 0
Charge is separated. Current flows.
Conservation of Energy
Charge builds up on each side
Takes more energy for each new charge.
Bulb dims
Chemical reaction in battery does not give enough
energy to push another charge on the capacitor.
Bulb goes out
7
(No Transcript)
8
The circuit below has been hooked up for a long
time. Which capacitor has the most charge on it?
Please note that all of the capacitors have a
total of zero charge. And so Im asking you to
compare the amount of q on each capacitor

• a
• b
• c
• a, b, and c all have the same charge

9
Three identical capacitors are hooked to a
battery as shown. Which has the most charge?
Follow up question Which capacitor has the
largest voltage difference?
C
C
C
DV
Solution
Solution
Use Conservation of Charge. We know that
whatever charge comes out of the battery goes
back into the battery Imagine a small amount of
charge moving q
Since we want to find out something about
voltage, use Energy Conservation
-q
C
C
C
DV
-q
10
Three identical capacitors are hooked to a
battery as shown. Which has the most charge?
This means that a the right plate of the right
capacitor gets a extra q charge. And the left
plate of the left capacitor loses negative
charges and thus becomes q.
C
C
C
-q
q
DV
Solution
Use Conservation of Charge. Whatever charge
comes out of the battery goes back into the
battery Imagine a small amount of charge moving
q
Well, now consider the right capacitor again.
The q charge is going to repel a q charge
away from the other side of a capacitor. Thus it
becomes q charge and the middle capacitor gets
a q charge.
-q
-q
q
q
C
-q
C
C
DV
-q
11
Which capacitor has the largest charge? Again,
Im asking you to compare the q on each capacitor

• a
• b
• c
• a, b, and c

Follow Up Which has the largest voltage?
12
Current in a Capacitor Circuit
DVb
R
I

C
DV
DVc
-
path
What is the current, as a function of the
properties of the bulb, the battery, the
capacitor and time?
Approach
Use conservation of charge Current is the same
through battery, bulb, and capacitor.
Use conservation of energy System a charged
energy carrier (electron) Begin and end path at
same place
Assume that the bulb is ohmic
Use ohms law
13
Current in a Capacitor Circuit
Target I
DVb
R
Conservation of energy
I
DVbat - DVb- DVc 0

C
DV
1
DVc
-
path
2
Bulb DVb IR
3
Capacitor CDVc q
What is the current, as a function of the
properties of the bulb, the battery, the
capacitor, and time?
Sub 2 and 3 into 1
DVbat - IR - q/C
Approach
Use conservation of charge Current is the same
through battery, bulb, and capacitor.
q is still unknown
Current and charge are related
4
Use conservation of energy System a charged
energy carrier (electron) Begin and end path at
same place
Pulling these equations together gives
Assume that the bulb is ohmic
Use ohms law
14
DVb
R
Solve
I
The meaning of the equation

C
DV
q is a function of time. When you take the
derivative of that function with respect to time
you get the same function plus a constant.
DVc
-
path
Guess q aekt b
Put the guess into the equation
15
Choose an easy time.
How about t 0, assume no charge separation (in
other words, no extra charge on capacitor) at
that time.
Changes with time
Doesnt change with time
and
Solution
Solution
Maximum charge separation at t infinity
What is a?
It is a constant.
If you know it at one time, you know it at all
times.
16
Time Evolution of Circuit
Now get the current
DVb
R
I

C
DVc
DV
-
initially ( t 0)
No charge separation in capacitor
q 0
Does this make sense?
No voltage difference across capacitor
Current is maximum initially ( t 0)
DVc 0
As if capacitor were a wire
DVb DV
Current is maximum
Current goes to 0 after a long time ( t
infinity)
As if it were an open circuit
17
As time goes on ( t NOT 0)
Charge separation in capacitor increases
q gt 0
Voltage difference across capacitor increases
DVc gt 0
DVb lt DV
Current decreases
After a long time (t infinity)
Charge separation in capacitor maximum
q CDV
Voltage difference across capacitor max
DVc DV
DVb 0
Current decreases
I 0
18
Energy Stored in Capacitor
Energy per charge input to the capacitor is the
voltage difference across the capacitor.
Changing!
Close look at the capacitor
Initial uncharged
Final charged
Energy initial
Energy final
Changes with time
Energy final gt Energy initial
Energy per charge input to the capacitor
increases as the charge on a capacitor plate
increases.
Energy input to the capacitor from the battery
Capacitor has increased internal energy
E is a function of q. What function?
Energy stored in the capacitor
Guess from the equation
How much?
Take the derivative of the function with respect
to q to get q times a constant.
19
Energy per charge input to the capacitor is the
voltage difference across the capacitor.
Changing!
What function is E?

• E a e-bq
• E a ebq
• E aq
• E aq2
• E a sin(bq)

a and b are constants
Changes with time
Energy per charge input to the capacitor
increases as the charge on a capacitor plate
increases.
E is a function of q. What function?
Guess from the equation
Take the derivative of the function with respect
to q to get q times a constant.
20
What function is E?

• E a e-bq
• E a ebq
• E aq
• E aq2
• E a sin(bq)

21
You are working in a research lab investigating
the movement of ions across cardiomyocyte cell
membranes through ion channels. You have read
that such membranes can be modeled as RC circuits
where a capacitor represents the insulating lipid
bilayer, a battery the membrane potential across
this bilayer, a resistor the membrane's ion
channels, and another resistor the resistance to
the ions moving along the cell membrane from the
battery to the channels. You have been assigned
to calculate the movement of the sodium ions
across this membrane. Measurements show the
capacitor is about 100pF, the channel resistance
is about 109 W, the other is twice as large, and
the membrane potential is approximated as a
constant 100mV even though it actually changes as
sodium ions move into the cell. To get an idea
of the ion movement, you first calculate the
maximum number of sodium ions that go through the
channel and then the time it takes for half of
them to go through. The sodium ion charge is
1.6x10-19C
DV
22
You are working in a research lab investigating
the movement of ions across cardiomyocyte cell
membranes through ion channels. You have read
that such membranes can be modeled as RC circuits
where a capacitor represents the insulating lipid
bilayer, a battery the membrane potential across
this bilayer, a resistor the membrane's ion
channels, and another resistor the resistance to
the ions moving along the cell membrane from the
battery to the channels. You have been assigned
to calculate the movement of the sodium ions
across this membrane. Measurements show the
capacitor is about 100pF, the channel resistance
is about 109 W, the other is twice as large, and
the membrane potential is approximated as a
constant 100mV even though it actually changes as
sodium ions move into the cell. To get an idea
of the ion movement, you first calculate the
maximum number of sodium ions that go through the
channel and then the time it takes for half of
them to go through. The sodium ion charge is
1.6x10-19C
DV1
R1
DV2
DV
DV3
C
R2
Na 1.6x10-19C
C 100 pF R1 2 x 109 W R2 109 W DV 100 mV

• Question
• How many sodium ions give the maximum charge of
  the capacitor?
• How much time does it take to get ½ that charge?

DV
23
Approach
DV1
R1
Find the charge of the capacitor after a long
time and divide that by the charge of a sodium
ion.
DV2
DV
DV3
C
R2
After a long time, I3 0
Na 1.6x10-19C
I1 I2
C 100 pF R1 2 x 109 W R2 109 W DV 100 mV
DV3 DV2

• Question
• How many sodium ions give the maximum charge of
  the capacitor?
• How much time does it take to get ½ that charge?

Use conservation of energy. Follow the charge
carrier around a loop.
Loop start at top of battery, end at top of
battery
Final energy initial energy Einput from
battery Eoutput from both resistors
0 -DV1 DV2 DV
24
Approach
DV1
R1
Find the charge separation of the capacitor after
a long time and divide that by the charge of a
sodium ion.
DV
DV2
DV2
C
R2
After a long time, I3 0
I1 I2
For the resistors
DV1 I R1 DV2 I R2
DV3 DV2
q3 C DV2
Use conservation of energy. Follow the charge
carrier around a loop.
Target q3
Eliminate each unknown in turn.
Loop start at top of battery, end at top of
battery
Eliminate DV1 from all equations
0 -IR1 DV2 DV
Final energy initial energy Einput from
battery Eoutput from both resistors
Eliminate DV2 from all equations
0 -IR1 IR2 DV
0 -DV1 DV2 DV
q3 C IR2
25
DV1
Eliminate I from all equations
R1
q3 C IR2
DV2
DV
DV2
C
q3 / (CR2) I
R2
For the resistors
DV1 I R1 DV2 I R2
q3 C DV2
Target q3
Eliminate each unknown in turn.
Eliminate DV1 from all equations
0 -IR1 DV2 DV
Eliminate DV2 from all equations
Check units Put in numbers Evaluate
0 -IR1 IR2 DV
q3 C IR2
26
Eliminate I from all equations
Now for question 2
q3 C IR2
DV1
R1
q3 / (CR2) I
DV
DV2
DV3
C
R2
Na 1.6x10-19C
C 100 pF R1 2 x 109 W R2 109 W DV 100 mV

• Question
• How many sodium ions give the maximum charge of
  the capacitor?
• How much time does it take to get ½ that charge?

Check units Put in numbers Evaluate
27
Approach
Now for question 2
Find the separation of the capacitor as a
function of time and find where it is half the
maximum.
DV1
R1
DV
DV2
DV3
C
R2
Same rules as usual for circuits
Na 1.6x10-19C
C 100 pF R1 2 x 109 W R2 109 W DV 100 mV
Use conservation of current.
To set this up label currents
Use conservation of energy.

• Question
• How many sodium ions give the maximum charge of
  the capacitor?
• How much time does it take to get ½ that charge?

To set this up label voltages and choose voltage
loops
28
DV1
R1
Lecture Question
DV
DV2
DV3
C
R2
Use conservation of current. I1 I2 I3
Use conservation of energy
2 loops
Loop 1 (blue loop)
becomes
0 -DV1 DV2 DV
Using ohms law becomes
0 -I1R1 I2R2 DV
29
Growth of Streptococcus pneumoniae (20 minute
video frames)
Change in the number per second depends on how
many are there
http//www.cellsalive.com/cam2.htm
30
Cell Growth
The number of cells that divide each second
depends on how many are there
Suppose a cell divides at a constant rate.
For example once per second
Predict how many cells will exist at any time
16
8
2
4
1
2 s
3 s
4 s
5 s
64
32
6 s
7 s
128
8 s
The number of cells that divide each second
depends on how many are there
31
The rate of cell division depends on the number
of cells that are there
The number of cells that divide each second
depends on how many are there
What is N as a function of time?
What function when you take its derivative gives
the same function back?
N Aebt C
Guess
e 2.718...
Try it
32
The rate of cell division depends on the number
of cells that are there
Time dependent parts must be equal
and
Time independent parts must be equal
The guess works if
What is N as a function of time?
Time dependent part
What function when you take its derivative gives
the same function back?
and
0 C
Time independent part
Therefore
Guess
N Aebt C
N Aekt
e 2.718...
What is A?
Use initial conditions
Try it
When t 0, N No
At t0,
No Aek0 A
N Noekt
Equation for cell growth
33
Exponential Growth
Time dependent parts must be equal
and
N e0.69t
Time independent parts must be equal
The guess works if
N
Time dependent part
and
0 C
Time independent part
Time in seconds
Therefore
N Aekt
What is A?
Use initial conditions
When t 0, N No
At t0,
No Aek0 A
N Noekt
Equation for cell growth
34
Question 1 On average, every minute a certain
type of bacteria divides. The sample started
with 10 bacteria. After 5 minutes, how many
bacteria are there?
Question 2 On average, every minute a bacteria
divides You check on your bacteria culture
after about 40 minutes of letting it grow. You
dont know how much you began with but you count
about 2000 now. How long ago, was your sample
half that size?