EM

1
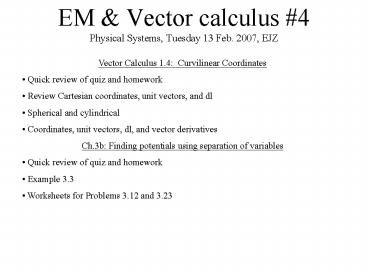
EM Vector calculus 4Physical Systems, Tuesday
13 Feb. 2007, EJZ

• Vector Calculus 1.4 Curvilinear Coordinates
• Quick review of quiz and homework
• Review Cartesian coordinates, unit vectors, and
  dl
• Spherical and cylindrical
• Coordinates, unit vectors, dl, and vector
  derivatives
• Ch.3b Finding potentials using separation of
  variables
• Quick review of quiz and homework
• Example 3.3
• Worksheets for Problems 3.12 and 3.23

2
Vector calculus HW Quiz review
Online solutions at http//192.211.16.13/curricula
r/physys/0607/solns/ HW VCsoln31.pdf,
EMsoln3a.pdf, EMsoln3b.pdf Quiz VCMidSoln.pdf,
EMmodMidSoln.pdf
3
Cartesian Coordinates
The infinitesimal displacement vector from
(x,y,z) to (xdx, ydy, zdz) is dl
4
Cylindrical Coordinates
5
Spherical Coordinates
6
Cylindrical Coordinates
Derive these (Problem 1.41)
7
Spherical Coordinates
Derive these (Problem 1.37)
8
Vector calculus HW due next week
Ch.1.4 Problems 1.37, 1.38, 1.41, 1.42
9
EM Ch.3b Separation of variables

• Quick review of quiz and homework
• When to use separation of variables?
• In charge-free regions
• With well-specified boundary conditions
• Without sufficient symmetry to use Gauss law
• How to use separation of variables?
• Guess form of solutions based on BC
• Separate variables, insert guessed solutions
  with constants
• Apply BC and solve for constants

10
Poisson and Laplace equations
Gauss
Potential combine to get Poissons eqn
Laplace equation holds in charge-free regions
Last week we found the general solutions to
Laplaces eqn. in spherical and cylindrical
coordinates for the case where V depends only on
r (Prob.3.3, p.116) ?
11
(No Transcript)
12
Solving Laplace w/ Separation of Variables
13
(No Transcript)
14
(No Transcript)
15
(No Transcript)
16
(No Transcript)
17
(No Transcript)
18
(No Transcript)
19
(No Transcript)
20
(No Transcript)
21
(No Transcript)
22
Worksheets for Problems 3.12 (136), 3.23 (145)
Homework due next week work through Ex.3.3, do
3.12 and 3.23. Extra credit 13, 24.