Heat of reaction DH0R
1 / 38
Title:
Heat of reaction DH0R
Description:
Heat of reaction DH0R. DH0R is positive exothermic. DH0R is negative endothermic ... DH0R is positive exothermic. DH0R is negative endothermic. Example: 2A 3B A2B3 ... –
Number of Views:137
Avg rating:3.0/5.0
Title: Heat of reaction DH0R
1
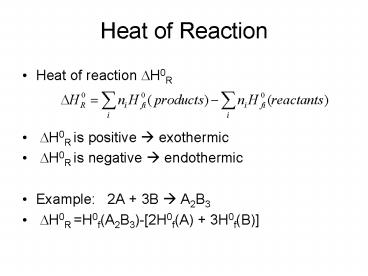
Heat of Reaction
- Heat of reaction DH0R
- DH0R is positive ? exothermic
- DH0R is negative ? endothermic
- Example 2A 3B ? A2B3
- DH0R H0f(A2B3)-2H0f(A) 3H0f(B)
2
Entropy of reaction
- Just as was done with enthalpies
- Entropy of reaction S0R
- When DS0R is positive ? entropy increases as a
result of a change in state - When DS0R is negative ? entropy decreases as a
result of a change in state
3
J. Willard Gibbs
- Gibbs realized that for a reaction, a certain
amount of energy goes to an increase in entropy
of a system. - G H TS or DG0R DH0R TDS0R
- Gibbs Free Energy (G) is a state variable,
measured in KJ/mol or Cal/mol - Tabulated values of DG0R are in Appendix F3-F5
4
G is a measure of driving force
- DG0R DH0R TDS0R
- When DG0R is negative ? forward reaction has
excess energy and will occur spontaneously - When DG0R is positive ? there is not enough
energy in the forward direction, and the BACKWARD
reaction will occur - When DG0R is ZERO ? reaction is AT equilibrium
5
Increasing energy with temp?
- The added energy in a substance that occurs as
temperature increases is stored in modes of
motion in the substance - For any molecule modes are vibration,
translation, and rotation - Solid ? bond vibrations
- Gases ? translation
- Liquid water complex function
6
Heat Capacity
- When heat is added to a phase its temperature
increases (No, really) - Not all materials behave the same though!
- dqCVdT ? where CV is a constant (heat capacity
for a particular material) - Or at constant P dqCpdT
- Recall that dqpdH then dHCpdT
- Relationship between CV and Cp
Where a and b are coefficients of isobaric
thermal expansion and isothermal compression,
respectively
7
Enthalpy at different temps
- HOWEVER ? C isnt really constant.
- C also varies with temperature, so to really
describe enthalpy of formation at any
temperature, we need to define C as a function of
temperature - Another empirical determination
- Cpa(bx10-3)T(cx10-6)T2
- Where this is a fit to experimental data and a,
b, and c are from the fit line (non-linear)
8
Does water behave like this?
- Water exists as liquid, solids, gas, and
supercritical fluid (boundary between gas and
liquid disappears where this happens is the
critical point) - Cp is a complex function of
- T and P (H-bond affinities),
- does not ascribe to Maier-
- Kelley forms
9
Volume Changes (Equation of State)
For Minerals
Volume is related to energy changes
Mineral volume changes as a function of T a,
coefficient of thermal expansion Mineral
volume changes as a function of P b, coefficient
of isothermal expansion
10
Volume Changes (Equation of State)
- Gases and liquids undergo significant volume
changes with T and P changes - Number of empirically based EOS solns..
- For metamorphic environments
- Redlich and Kwong equation
- V-bar denotes a molar quantity, aRw and bRK are
constants - EOS then equates energy (Helmholtz fee energy) to
P
11
- Now, how does free energy change with T and P?
- From DGDH-TDS
12
Phase Relations
- Rule At equilibrium, reactants and products have
the same Gibbs Energy - For 2 things at equilibrium, can investigate the
P-T relationships ? different minerals change
with T-P differently - For DGR DSRdT DVRdP, at equilibrium, DG0,
rearranging
Clausius-Clapeyron equation
13
V Vº(1-bDP)
DSR change with T or P?
- DV for solids stays nearly constant as P, T
change, DV for liquids and gases DOES NOT - Solid-solid reactions linear ? S and V nearly
constant, DS/DV constant ? slope in diagram - For metamorphic reactions involving liquids or
gases, volume changes are significant, DV terms
large and a function of T and P (and often
complex functions) slope is not linear and can
change sign (change slope to )
14
(No Transcript)
15
Example Diamond-graphite
- To get C from graphite to diamond at 25ºC
requires 1600 MPa of pressure, lets calculate
what P it requires at 1000ºC
16
Phase diagram
- Need to represent how mineral reactions at
equilibrium vary with P and T
17
Gibbs Phase Rule
- The number of variables which are required to
describe the state of a system - pfc2 fc-p2
- Where p of phases, c of components,
- f degrees of freedom
- The degrees of freedom correspond to the number
of intensive variables that can be changed
without changing the number of phases in the
system
18
Variance and f
- fc-p2
- Consider a one component (unary) diagram
- If considering presence of 1 phase (the liquid,
solid, OR gas) it is divariant - 2 phases univariant
- 3 phases invariant
19
Heat of Reaction
- Heat of reaction DH0R
- DH0R is positive ? exothermic
- DH0R is negative ? endothermic
- Example 2A 3B ? A2B3
- DH0R H0f(A2B3)-2H0f(A) 3H0f(B)
20
Entropy of reaction
- Just as was done with enthalpies
- Entropy of reaction S0R
- When DS0R is positive ? entropy increases as a
result of a change in state - When DS0R is negative ? entropy decreases as a
result of a change in state
21
J. Willard Gibbs
- Gibbs realized that for a reaction, a certain
amount of energy goes to an increase in entropy
of a system. - G H TS or DG0R DH0R TDS0R
- Gibbs Free Energy (G) is a state variable,
measured in KJ/mol or Cal/mol - Tabulated values of DG0R are in Appendix F3-F5
22
G is a measure of driving force
- DG0R DH0R TDS0R
- When DG0R is negative ? forward reaction has
excess energy and will occur spontaneously - When DG0R is positive ? there is not enough
energy in the forward direction, and the BACKWARD
reaction will occur - When DG0R is ZERO ? reaction is AT equilibrium
23
Free Energy Examples
- DG0R DH0R TDS0R
- H2O(l)-63.32 kcal/mol (NIST value
http//webbook.nist.gov/chemistry/) - Fe2 ¼ O2 H ? Fe3 ½ H2O
- -4120(-633200.5)--21870(39540.25)
- -67440--19893-47547 cal/mol
24
Chemical Potential
- Enthalpy (H), entropy (S), and Gibbs Free Energy
(G) are molal (moles/kg) quantities - Chemical potential, m, is the Gibbs free energy
per molal unit - In other words, the "chemical potential mi" is a
measure of how much the free energy of a system
changes (by dGi) if you add or remove a number
dni particles of the particle species i while
keeping the number of the other particles (and
the temperature T and the pressure P) constant
25
Mixing
- Putting two components into the same system
they mix and potentially interact - Mechanical mixture no chemical interaction
where X is mole fraction of A, B - ms XAmA XBmB
- Random mixture particles spontaneously (so m
must go down) orient randomly - Dmmixms mmechanical mixing
- Mixing ideal IF interaction of A-A A-B B-B ?
if that is true then DHmix0, so DSmix must be gt0
(because mmixlt0 (spontaneous mixing) - DSid mix -RSXilnXi
Rmolar gas constant Xmole fraction component i
26
Mixing, ideal systems
27
Mixing, real systems
- When components interact with each other
chemically and change the overall solution energy - Dmreg ?XAXB
- Particularly this formulation is important in
geochemistry for solid solutions of minerals,
such as olivine (ex Fo50Fa50)
28
Law of Mass Action
- Getting out of the standard state
- Accounting for free energy of ions ? 1
- mm0 RT ln P
- Bear in mind the difference between the standard
state G0 and m0 vs. the molar property G and m
(not at standard state ? 25 C, 1 bar, a mole)
GP G0 RT(ln P ln P0)
GP G0 RT ln P
29
Equilibrium Constant
- For a reaction of ideal gases, P becomes
- for aA bB ? cC dD
- Restate the equation as
- DGR DG0R RT ln Q
- AT equilibrium, DGR0, therefore
- DG0R -RT ln Keq
- where Keq is the equilibrium constant
30
Assessing equilibrium
- If DGR DG0R RT ln Q, and at equilibrium DG0R
0, then QK - Q ? reaction quotient, aka Ion Activity Product
(IAP) is the product of all products over product
of all reactants at any condition - K ? aka Keq, same calculation, but AT equilibrium
31
Saturation Index
- When DGR0, then ln Q/Keq0, therefore QKeq.
- For minerals dissolving in water
- Saturation Index (SI) log Q/K or IAP/Keq
- When SI0, mineral is at equilibrium, when SIlt0
(i.e. negative), mineral is undersaturated
32
Calculating Keq
- DG0R -RT ln Keq
- Look up G0i for each component in data tables
(such as Appendix B in your book) - Examples
- CaCO3(calcite) 2 H ? Ca2 H2CO3(aq)
- CaCO3(aragonite) 2 H ? Ca2 H2CO3(aq)
- H2CO3(aq) ? H2O CO2(aq)
- NaAlSiO4(nepheline) SiO2(quartz) ?
NaAlSi3O8(albite)
33
Application to ions in solution
- Ions in solutions are obviously nonideal states!
- Use activities (ai) to apply thermodynamics and
law of mass action - ai gimi
- The activity coefficient, gi, is found via some
empirical foundations
34
Ion Activity Product
- For reaction aA bB ? cC dD
- For simple mineral dissolution, this is only the
product of the products ? activity of a solid
phase is equal to one - CaCO3 ? Ca2 CO32-
- IAP Ca2CO32-
35
Solubility Product Constant
- For mineral dissolution, the K is Ksp, the
solubility product constant - Use it for a quick look at how soluble a mineral
is, often presented as pK (table 10.1) - DG0R RT ln Ksp
- Higher values ? more soluble
- CaCO3(calcite) ? Ca2 CO32-
- Fe3(PO4)28H2O ? 3 Fe2 2 PO43- 8 H2O
36
Activity
- Activity, a, is the term which relates Gibbs Free
Energy to chemical potential - mi-G0i RT ln ai
- Why is there now a correction term you might ask
- Has to do with how things mix together
- Relates an ideal solution to a non-ideal solution
37
Activity II
- For solids or liquid solutions
- aiXigi
- For gases
- aiPigi fi
- For aqueous solutions
- aimigi
Ximole fraction of component i Pi partial
pressure of component i mi molal concentration
of component i
38
Activity Coefficients
- Where do they come from??
- We think of ideal as the standard state, but
for dissolved ions, that is actually an
infinitely dilute solution - Gases, minerals, and bulk liquids (H2O) are
usually pretty close to 1 in waters - Dissolved molecules/ ions are have activity
coefficients that change with concentration (ions
are curved, molecules usually more linear
relation)