ENGINEERING MATHEMATICS II - PowerPoint PPT Presentation
1 / 49
Title:
ENGINEERING MATHEMATICS II
Description:
Also we shall discuss the application of differentiation to indeterminate forms ... determination of a function which are greatest or least in their neighbour hoods. ... – PowerPoint PPT presentation
Number of Views:2326
Avg rating:3.0/5.0
Title: ENGINEERING MATHEMATICS II
1
ENGINEERING MATHEMATICS - II
2
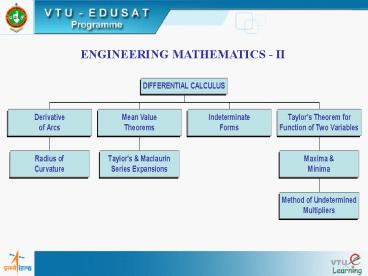
Differential Calculus
Introduction
We have already studied the Cartesian and polar
curves. In this chapter we will learn about
derivative of arcs and radius of curvature in
Cartesian, parametric and polar forms. In this
chapter we shall discuss, Rolle's theorem,
Lagrange's Mean value theorem. Cauchy's Mean
value theorem, Taylor's theorem, Taylor's and
Maclaurin series expansions of functions both in
single and two variables. Also we shall discuss
the application of differentiation to
indeterminate forms using L'Hospital rule, and
application of differential calculus to the
determination of a function which are greatest or
least in their neighbour hoods.
3
Derivatives of Arc
Derivative of the length of the arc for the
Cartesian curve y f(x). Let y f(x) be the
equation of the curve. A be a fixed point on the
curve. Let P(x, y) and
be two neighbouring points on the
curve such that arc AP s, arc PQ ds and
chord PQ dc. As on the curve
4
We have
5
- If the equation of the curve is x f(y) then
- If the equation of the curve is in parametric
form x x(t) and y y(t) - where t is the parameter then
6
Additional Results
We know that
again
7
Derivative of Arc Length in Polar Form
Let
be two neighbouring points on the curve
Let arc and chord
. As Q approaches P on the curve
8
From DONP since dq is very small,
?
?
Since dq is small
9
We have NQ OQ - ON
PQ2 PN2 NQ2
10
11
- we have
12
?
?
13
Radius of Curvature
14
Formula for radius curvature in Cartesian form
We have
Differentiate with respect to x.
15
Formula for radius of curvature in parametric
form
Let x x(t) and y y(t)
16
We have
17
Radius of curvature in pedal form
By definition
18
We have p r sin f
Differentiating with respect to r,
Comparing (1) and (2)
19
Radius of curvature in polar form
20
differentiating with respect to q
Dividing by -2r1
21
(No Transcript)
22
Problem 01
In the ellipse , show
that the radius of curvature at an end of the
major axis is equal to the semi latus rectum.
Solution
,
One end of major axis is (a, 0) in
Differentiating w.r.t x, we get, We have
23
24
Problem 02
Find the radius of curvature to the curve x a
(t - sin t), y a (1 - cos t) at any point t.
Solution
Let x a (t - sin t), y a (1 - cos t)
We have
25
(No Transcript)
26
Problem 03
Find the radius of curvature for the curve whose
pedal equation is given by pa2 r3.
Solution
Let pa2 r3 .....(1) Differentiate with
respect to p (1)
We have
27
Problem 04
Find the radius of curvature for the curve r
aeq cot a
Solution
Let r aeqcot a
We have
28
We get, p r sin a as the p-r equation
Differentiate with respect to p
29
Problem 05
With usual notations, prove that
Solution
30
From (1) and (2)
Differentiate with respect to y
31
Rolle's theorem
Statement If a function f(x) is (i) Continuous
in a, b (ii) Differentiable in (a, b)
and (iii) f(a) f(b)
32
Geometrical Interpretation
If the graph of f(x) be drawn between x a and x
b having a unique tangent at all points in
the above interval and f(a) f(b), then there
exits at least one point C on the curve
(corresponding to x c between x a and x b),
such that the tangent at C is parallel to x-axis.
33
Note 1 There may exists more than one at which
f'(x) vanishes.
34
Note 2 The three conditions of Rolle's theorem
are the sufficient conditions (but not
necessary) for f'(x) 0 for some
Note 3 Conclusion of Rolle's theorem does not
hold good for a function which does not satisfy
any of its conditions. Example consider the
function f(x) x in -1, 1
Observe that i) f(x) is continuous in -1,
1 ii) f(-1) 1 f(1)
35
But f(x) is not differentiable in (-1, 1) because
Since all the three conditions of Rolle's theorem
are not satisfied. Hence the conclusion is not
valid in -1, 1.
36
Lagrange's Mean value theorem (LMVT)
Let f(x) be a function such that i) Continuous
in a, b ii) Differentiable in (a, b)
37
Proof Construct a function F(x) such
that F(x) f(x) - Ax, where A is a constant
such that F(a) F(b) i.e,. f(a) - Aa f(b)
- Ab
i) Now F(x) is continuous in a,b
f(x), x is continuous ii) Since f(x), x is
derivable, F(x) is also derivable in (a,
b) (iii) Also F(a) F(b)
38
Hence F (x) satisfies all the conditions of the
Rolle's theorem.
Which proves the Lagrange's Mean Value Theorem.
39
Another form of LMVT
Let b - a h We have a lt c lt b
Note Rolle's theorem is a special case of LMVT.
40
Geometrical Interpretation of LMVT
Let the graph of f(x) be continuous between A(a,
f(a)) and B(b, f(b)). Let the curve have
tangents at all points between A and B then there
exists C(c, f(c)) on the curve between A and B
such that the tangent at C is parallel to the
chord AB.
41
Note c is not unique
42
Cor. then
f (x) is strictly increasing Let f(x) satisfy
the conditions of LMVT in a, b. Let x1, x2 be
any two points of a, b such that
x1ltx2 Applying LMVT to x1, x2
Since f'(c)gt0
\ f(x) is strictly increasing.
43
Cauchy's Mean Value theorem
Statement If f(x) and g(x) are any two
functions such that (i) f(x) and g(x) are both
continuous in a, b (ii) f(x) and g(x) are both
differentiable in (a,b) and
44
Proof Consider F(x) f(x) - Ag(x) where A is a
constant to be determined such that F(a)
F(b) (i) Since f(x) and g(x) is continuous in
a, b F(x) is also continuous (ii) Since f(x)
and g(x) are derivable in (a, b) F(x) is
also derivable in (a, b) (iii) F(a) F(b)
45
F(x) satisfies all the conditions of Rolle's
theorem
46
Physical Interpretation of CMVT
CMVT interprets that the ratio of actual rates of
increase of f(x) and g(x) at x c is equal to
the ratio of their average rate of increase of
the functions in the interval (a, b).
47
Taylor's Theorem (Statement Only)
Let f(x) be a function such that (i) f, f', f'',
,f(n-1) are continuous in a, b (ii) f(n-1)
is differentiable in (a, b) then
48
Another from of Taylor's
Put a h x then (2) becomes
49