7.3 Distributed Loads

1
7.3 Distributed Loads

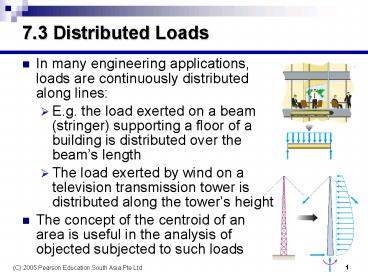
• In many engineering applications, loads are
  continuously distributed along lines
• E.g. the load exerted on a beam (stringer)
  supporting a floor of a building is distributed
  over the beams length
• The load exerted by wind on a television
  transmission tower is distributed along the
  towers height
• The concept of the centroid of an area is useful
  in the analysis of objected subjected to such
  loads

2
7.3 Distributed Loads

• Describing a Distributed Load
• Suppose that we pile bags of sand on a beam
• It is clear that the load exerted by the bags is
  distributed over the length of the beam that
  its magnitude at a given position x depends on
  how high the bags are piled at that position

3
7.3 Distributed Loads

• T describe the load, we define a function w such
  that the downward force exerted on an
  infinitesimal element dx of the beam is w dx
• With this function we can model the varying
  magnitude of the load exerted by the sand bags
• The arrows in the figure indicate that the load
  acts in a downward direction

4
7.3 Distributed Loads

• Loads distributed along lines, from simple
  examples such as the beams own weight to
  complicated ones such as the lift distributed
  along the length of an airplanes wing, are
  modeled by the function w
• Since the product of w dx is a force, the
  dimensions of w are (force)/(length)
• w can be expressed in newtons per meter (SI units)

5
7.3 Distributed Loads

• Determining Force Moment
• Assuming that the function w describing a
  particular distributed load is known
• The graph of w is called the loading curve
• The force acting on an element dx of the line is
  w dx

6
7.3 Distributed Loads

• The total force F exerted by the distributed load
  is determined by integrating the loading curve
  with respect to x
• (7.10)
• We can also integrate to determine the moment
  about a point exerted by the distributed load
• The moment about the origin due to the force
  exerted on the element dx is xw dx

7
7.3 Distributed Loads

• The total moment about the origin due to the
  distributed load
• (7.11)

• When you are concerned only with the total force
  moment exerted by a distributed load, you can
  represent it by a single equivalent force F

8
7.3 Distributed Loads

• For equivalence, the force must act at a position
  on the x axis such that the moment of F about
  the origin is equal to the moment of the
  distributed load about the origin
• Therefore the force F is equivalent to the
  distributed load if we place it at the position
• (7.12)

9
7.3 Distributed Loads

• The Area Analogy
• Notice that the term w dx is equal to an element
  of area dA between the loading curve the x
  axis (w dx is actually a force not an area)

10
7.3 Distributed Loads

• Interpreted in this way, Eq. (7.10) states that
  the total force exerted by the distributed load
  is equal to the area A between the loading
  curve the x axis
• (7.13)
• Substituting w dx dA into Eq. (7.12)
• (7.14)

11
7.3 Distributed Loads

• The force F is equivalent to the distributed load
  if it acts at the centroid of the area between
  the loading the x axis

12
Example 7.5 Beam with a Triangular Distributed
Load

• The beam in Fig. 7.14 is subjected to a
  triangular
• distributed load whose value at B is 100 N/m.
• (a) Represent the distributed load by a single
  equivalent force.
• (b) Determine the reactions at A B.

Fig. 7.14
13
Example 7.5 Beam with a Triangular Distributed
Load

• Strategy
• (a) The magnitude of the force is equal to the
• area under the triangular loading curve
  the
• equivalent force acts at the centroid of the
  
• triangular area.
• (b) Once the distributed load is represented by a
  
• single equivalent force, we can apply the
• equilibrium equations to determine the
  reactions.

14
Example 7.5 Beam with a Triangular Distributed
Load

• Solution
• (a) The area of the triangular distributed load
  is
• one-half its base times its height or
• The centroid of the triangular area is located
  at

15
Example 7.5 Beam with a Triangular Distributed
Load

• Solution
• We can therefore represent the distributed
  load by an equivalent downward force of 600-N
  magnitude acting at x 8 m

16
Example 7.5 Beam with a Triangular Distributed
Load

• Solution
• (b) From the equilibrium equations
• S Fx Ax 0
• S Fy Ay B ? 600 N 0
• S Mpoint A (12 m)B ? (8 m)(600 N) 0
• we obtain Ax 0, Ay 200 N B 400 N.

17
Example 7.5 Beam with a Triangular Distributed
Load

• Critical Thinking
• This analogy made it very straightforward to
  represent the triangular distributed load in this
  example by an equivalent force
• For comparison, you should determine the
  reactions at A B by using Eqs. (7.10) (7.11)
  to calculate the force moment exerted by the
  distributed load

18
Example 7.6 Beam Subjected to Distributed Loads

• The beam in Fig. 7.15 is subjected to 2
  distributed loads. Determine the reactions at A
  B.

Fig. 7.15
19
Example 7.6 Beam Subjected to Distributed Loads

• Strategy
• We can easily apply the area analogy to the
  uniformly distributed load between A B. We will
  treat the distributed load on the vertical
  section of the beam as the sum of uniform
  triangular distributed loads use the area
  analogy to represent each distributed load by an
  equivalent force.

20
Example 7.6 Beam Subjected to Distributed Loads

• Solution
• Draw the free-body diagram of the beam
  expressing the left distributed load as the sum
  of uniform triangular loads

21
Example 7.6 Beam Subjected to Distributed Loads

• Solution
• Represent the 3 distributed loads by
  equivalent forces
• The area of the uniform distributed load on
  the right is
• and the centroid is 3 m from B.

22
Example 7.6 Beam Subjected to Distributed Loads

• Solution
• The area of the uniform distributed load on the
• vertical part of the beam is
• and its centroid is located at y 3 m.
• The area of the triangular distributed load is
• And its centroid is located at

23
Example 7.6 Beam Subjected to Distributed Loads

• Solution
• From the equilibrium equations
• S Fx Ax 1200 N 2400 N 0
• S Fy Ay B ? 2400 N 0
• S Mpoint A (6 m)B ? (3 m)(2400 N) ? (2 m)(1200
  N)
• ? (3 m)(2400 N) 0
• we obtain Ax ?3600 N, Ay ?400 N B 2800 N.

24
Example 7.6 Beam Subjected to Distributed Loads

• Critical Thinking
• The area analogy is useful when a loading curve
  is sufficiently simple that its area the
  location of its centroid are easy to determine
• When that is not the case, you can use Eqs.
  (7.10) (7.11) to determine the force moment
  exerted by a distributed load

25
Example 7.7 Beam with a Distributed Load

• The beam in Fig. 7.16 is subjected to a
  distributed load, a force a couple. The
  distributed load is w 300x 50x2 0.3x4
  N/m. Determine the reactions at the fixed support
  A.

Fig. 7.16
26
Example 7.7 Beam with a Distributed Load

• Strategy
• Since we know the function w, we can use Eqs.
  (7.10) (7.11) to determine the force moment
  exerted on the beam by the distributed load. We
  can then use the equilibrium equations to
  determine the reactions at A.

27
Example 7.7 Beam with a Distributed Load

• Solution
• Isolate the beam show the reactions at the
  fixed
• support
• The downward force exerted by the distributed
• load is

28
Example 7.7 Beam with a Distributed Load

• Solution
• The clockwise moment about A exerted by the
• distributed load is
• From the equilibrium equations
• S Fx Ax 0
• S Fy Ay 4330 N 2000 N 0
• S Mpoint A MA ? 25000 N-m (20 m)(2000 N)
• 10000 N-m 0
• we obtain Ax 0, Ay 2330 N MA ?25000 N.

29
Example 7.7 Beam with a Distributed Load

• Critical Thinking
• When you use Eq. (7.11), it is important to be
  aware that you are calculating the clockwise
  moment due to the distributed load w about the
  origin x 0.

30
7.4 Centroids of Volumes Lines

• Definitions
• Volumes
• Consider a volume V let dV be a differential
  element of V with coordinates x, y z

• By analogy with Eqs. (7.6) (7.7), the
  coordinates of the centroid V are
• (7.15)
• The subscript V on the integral sign means that
  the integration is carried out over the entire
  volume

31
7.4 Centroids of Volumes Lines

• If a volume has the form of a plate with uniform
  thickness cross-sectional area A, its centroid
  coincides with the centroid of A lies at the
  midpoint between the 2 faces
• To show that this is true, we obtain a volume
  element dV by projecting an element dA of the
  cross-sectional area through the thickness T of
  the volume, so that dV T dA

32
7.4 Centroids of Volumes Lines

• The x y coordinates of the centroid of the
  volume are
• The coordinate by symmetry
• Thus you know the centroid of this type of volume
  if you (or can determine) the centroid of its
  cross-sectional area

33
7.4 Centroids of Volumes Lines

• Lines
• The coordinates of the centroid of a line L are
• (7.16)
• where dL is a differential
• length of the line with the
• coordinates x, y z.

34
Example 7.8 Centroid of a Cone by Integration
Fig. 7.20

• Determine the centroid of the cone in Fig.
  7.20.

Strategy The centroid must lie on the x axis
because of symmetry. We will determine its x
coordinate by using an element of volume dV in
the form of a disk of width dx.
35
Example 7.8 Centroid of a Cone by Integration

• Solution
• Let dV be the disk
• The radius of the disk is
• (R/h) x
• and its volume equals the
• product of the area of the
• disk its thickness

36
Example 7.8 Centroid of a Cone by Integration

• Solution
• To integrate over the entire volume, we must
• integrate with respect to x from x 0 to x h.
• The x coordinate of the centroid is

37
Example 7.8 Centroid of a Cone by Integration

• Critical Thinking
• Notice that we determined the centroid of the
  cone by using an element of volume of the form dV
  A dx, where A is the cones cross-sectional
  area
• You can use this approach to determine the
  centroids of other volumes of revolution
  pyramidal volumes

38
Example 7.9 Centroid of a Line by Integration

• The line in Fig. 7.21 is defined by the
  function y x2. Determine the x coordinate of
  its centroid.

Fig. 7.21
39
Example 7.9 Centroid of a Line by Integration

• Strategy
• We can express a differential
• element dL of a line in terms of dx
• dy

From the equation describing the line, the
derivative dy/dx 2x, so we obtain an
expression for dL in terms of x
40
Example 7.9 Centroid of a Line by Integration

• Solution
• To integrate over the entire line, we must
  integrate
• from x 0 to x 1.
• The x coordinate of the centroid is

41
Example 7.9 Centroid of a Line by Integration

• Critical Thinking
• Our approach in this example is appropriate to
  determine the centroid of a line that is
  described by a function of the form y f(x)
• In Example 7.10 we show how to determine the
  centroid of a line that is described in terms of
  polar coordinates

42
Example 7.10 Centroid of a Semicircular Line by
Integration

• Determine the centroid of the semicircular
  line in Fig. 7.22.

Fig. 7.22
43
Example 7.10 Centroid of a Semicircular Line by
Integration

• Strategy
• Because of the symmetry of the line, the
  centroid lies on the x axis. To determine , we
  will integrate in terms of polar coordinates. By
  letting ? change by the amount d?, we obtain a
  differential line element of length dL R d?.
  The x coordinate of dL is x R cos ?.

44
Example 7.10 Centroid of a Semicircular Line by
Integration

• Solution
• To integrate over the entire line, we must
  integrate
• with respect to ? from
  
• Critical Thinking
• Notice that our integration procedure gives the
• correct length of the line

45
7.4 Centroids of Volumes Lines

• Centroids of Composite Volumes Lines
• The centroids of composite volumes lines can be
  derived using the same approach we applied to
  areas
• The coordinates of the centroid of a composite
  volume are
• (7.17)

46
7.4 Centroids of Volumes Lines

• The coordinates of the centroid of a composite
  line are
• (7.18)

47
7.4 Centroids of Volumes Lines

• Determining the centroid of a composite volume or
  line requires 3 steps
• 1.Choose the parts try to divide the composite
  into parts whose centroids you know or can easily
  determine
• 2.Determine the values for the parts determine
  the centroid the volume or length of each part.
  Watch for instances of symmetry that can simplify
  the task
• 3.Calculate the centroid use Eqs. (7.17) or
  (7.18) to determine the centroid of the composite
  volume or line

48
Example 7.11 Centroid of a Composite Volume
Fig. 7.23

• Determine the centroid of the volume in Fig.
  7.23.

Strategy Divide the volume into parts (the
parts are obvious in this example), determine the
centroids of the parts apply Eqs. (7.17).
49
Example 7.11 Centroid of a Composite Volume

• Solution
• Choose the Parts
• The volume consists of a cone a cylinder, which
  
• we call parts 1 2, respectively.
• Determine the Values for the Parts

50
Example 7.11 Centroid of a Composite Volume

• Solution
• The information for determining the x coordinate
  of
• the centroid is summarized in Table 7.4

51
Example 7.11 Centroid of a Composite Volume

• Solution
• Calculate the Centroid
• The x coordinate of the centroid of the composite
  
• volume is
• Because of symmetry,

52
Example 7.11 Centroid of a Composite Volume

• Critical Thinking
• We will show in Section 7.7 that the center of
  mass of a homogenous object coincides with the
  centroid of its volume
• Therefore our solution in this example determines
  the location of the center of mass of a
  homogenous object that occupies this volume
• This is 1 of our principal motivations for
  introducing the concept of a centroid in statics

53
Example 7.12 Centroid of a Volume Containing a
Cutout

• Determine the centroid of the volume in Fig.
  7.24.

Fig. 7.24
54
Example 7.12 Centroid of a Volume Containing a
Cutout

• Strategy
• Divide this volume into the 5 simple parts
  shown. Notice that parts 2 3 do not have the
  cutout. It is assumed to be filled in, which
  simplifies the geometries of those parts. Part 5,
  which is the volume of the 20-mm-diameter hole,
  will be treated as a negative volume in Eqs.
  (7.17).

55
Example 7.12 Centroid of a Volume Containing a
Cutout

• Solution
• Choose the Parts
• Divide the volume into the 5 simple parts shown.
• Part 5 is the volume of the 20-mm-diameter hole.
• Determine the Values for the Parts
• The centroids for parts 1 3 are located at the
• centroids of their semicircular cross sections

56
Example 7.12 Centroid of a Volume Containing a
Cutout

• Solution
• The information for determining the x-coordinate
  of
• the centroid is summarized in Table 7.5. Part 5
  is a
• negative volume.

57
Example 7.12 Centroid of a Volume Containing a
Cutout

• Solution
• Calculate the Centroid
• The x coordinate of the centroid of the composite
  
• volume is

58
Example 7.12 Centroid of a Volume Containing a
Cutout

• Solution
• The z coordinates of the centroids of the parts
  are
• zero except
• Therefore the z coordinate of the centroid of the
  
• composite volume is
• Because of symmetry,

59
Example 7.12 Centroid of a Volume Containing a
Cutout

• Critical Thinking
• You can recognize that the volume in this example
  could be part of a mechanical device
• Many manufactured parts have volumes that are
  composites of simple volumes the method used in
  this example can be used to determine their
  centroids if they are homogenous, their centers
  of mass

60
Example 7.13 Centroid of a Composite Line

• Determine the centroid of the volume in Fig.
  7.25. The quarter-circular arc lies in the y-z
  plane.

Fig. 7.25
Strategy Divide the line into parts (in this
case the quarter-circular arc the 2 straight
segments), determine the centroids of the parts
apply Eqs. (7.18).
61
Example 7.13 Centroid of a Composite Line

• Solution
• Choose the Parts
• The line consists of a quarter-circular arc 2
• straight segments, which we call parts 1, 2 3

62
Example 7.13 Centroid of a Composite Line

• Solution
• Determine the Values for the Parts
• The coordinates of the centroid of the quarter-
• circular arc
• The centroids of the straight segments lie at
  their
• midpoints.
• For segment 2,
• and for segment 3,
• The length of segment 3 is

63
Example 7.13 Centroid of a Composite Line

• Solution
• The information is summarized in Table 7.6

Table 7.6 Information for determining the centroid
64
Example 7.13 Centroid of a Composite Line

• Solution
• Calculate the Centroid
• The coordinates of the centroid of the composite
• line are

65
Example 7.13 Centroid of a Composite Line

• Critical Thinking
• Reason for wanting to know the centroid (average
  position) of a line
• In Section 7.7 we show that the center of mass of
  a slender homogenous bar, which is the point at
  which the weight of the bar can be represented by
  an equivalent force, lies approximately at the
  centroid of the bars axis