What if s is unknown

1
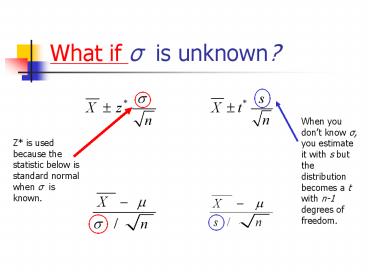
What if s is unknown?
When you dont know s, you estimate it with s but
the distribution becomes a t with n-1 degrees of
freedom.
Z is used because the statistic below is
standard normal when s is known.
2
(No Transcript)
3
Properties of the t-distn.

• For the Z (standard
• normal)
• Symmetric about 0
• By the Emperical Rule, it ranges approximately
  from -3 to 3

• For the t
• Symmetric about 0
• Ranges approximately from -5 to 5

4
Finding t

• You find t just as you find z except that you
  use Table B-3, you go to the row that corresponds
  to n-1 degrees of freedom.
• If you want to be 90 confident and n20, then
  .

t 1.729
5
7.2 Confidence interval for ? based on
when ? is unknown the t-interval.
The standard error of the estimate.(SE)
An estimate for µ
Depends on how confident you want to be.
6
Margin of Error

• The margin of error (ME ) is half the width of
  the confidence interval.

7
Steps to calculate CI for ?

• Calculate estimate and SE( )
• where s is the sample standard deviation
• Find the critical value t from the Table. Its
  the upper ?/2 critical value with dfn-1
• ME t SE
• (1-?)100 CI for ? is given by
• CI ME

8
Finding the sample size.

• For a given bound B on the margin of error, the
  sample size

9
Example 7.2 continued

• Compute a 90 confidence interval for the mean
  fuel capacity of this model of car based on a
  sample of 16 cars with sample mean 18 gallons and
  s3.5.

10

• (16.466,19.534)

11
Types of tests

• One-sided alternative like Ha ? gt ?0 Ha ?
  lt ?0
• focuses on deviations from the null hypothesis
  value in only one direction.
• Two-sided alternative like Ha ? ? ?0 concern
  the deviation from the null hypothesis in either
  direction.
• right-tailed test
• left-tailed test
• two-tailed test

12
P-value for right tailed test
p-value P(ZgtZobs)
For both, the p-value is the area to the right of
the TS.
TS
TS
Here, the p-value is less than a.
Here, the p-value is greater than a.
13
P-value for left tailed test
p-value P(ZltZobs)
TS
TS
For both, the p-value is the area to the left of
the TS.
14
P-value for Two Sided Test
Ha ? ? ?0
p-value 2P(Z gt Zobs)
P-value/2
P-value/2
TS
TS
For both, half of the p-value is the area to the
right of the Zobs. Zobs gt0
15
P-value for Two Sided Test
Ha ? ? ?0
p-value 2P(Z gt Zobs)
P-value/2
P-value/2
TS
TS
For both, half of the p-value is the area to the
left of the Zobs. Zobslt0
16
p-Value

• It depends on the right-tailed, left-tailed and
  two-tailed test.
• Left tailed test p-value P(ZltZobs)
• Right tailed test p-value P(ZgtZobs)
• Two tailed test p-value 2P(ZgtZobs)

17
Example 8.3

• A mathematician (John Kerrich) tossed a coin
  10,000 times to determine whether it was fair. He
  actually got 5067 heads.

18

• Simple Answer
• P-value0.1802gt0.05
• Based on the data, there is insufficient evidence
  to say that the coin is not fair.

19
Exercise 8.1

• The standard medication for a certain disease is
  effective in 60 of all cases. A drug company
  believes its new drug is more effective than the
  old treatment.
• Suppose that the drug company investigates 200
  cases and finds that the new drug is effective in
  134 cases. Is this enough evidence to reject Ho
  and say that ?gt.60?

20

• Ho ?.60 Ha ?gt.60
• P-value.0217lt0.05
• Reject H0

21
Possible errors

• Deciding against H0 when it is in fact true,
  this is called a Type I error.
• In the legal analogy, a Type I error means
  convicting an innocent person.
• Deciding to stick with H0 when Ha is in fact
  true, this is called a Type II error.
• In the legal analogy, a Type II error means
  acquitting a guilty person.

22
Example 8.4

• In medical disease testing,
• H0A person tested is healthy
• Ha The person has the disease we are testing for
• What would be the Type I and Type II error
• here? What type of error would the person
  consider more serious?

23

• Type I error A healthy person is diagnosed with
  the disease.
• Type II error An infected person is diagnosed as
  disease free.

24
Note

• Which of these errors seems more serious depends
  on the situation and your point of view.

25
4 Possibilities
OUR DECISION
THE TRUTH
Fail to Reject H0 (H0 is TRUE)
The ones in green are correct decisions No
Error. The ones in red are errors.
H0 is TRUE
Reject H0 (H0 is FALSE)
Fail to Reject H0 (H0 is TRUE)
H0 is FALSE
Reject H0 (H0 is FALSE)
26
Illustration of two types of error
The truth
a
Decision
ß
27
Probability of two types of error

• When H0 is true, only type I error probably
  happen, with probability
• a P(Type I Error)
• When H0 is false, only type II error probably
  happen, with probability
• ß P(Type II Error)
• Note Reducing ßcould increase a, vice versa.

28
Making a decision
Standard Normal
The Critical Value is where this shading starts.
If the test statistic falls in this region, well
reject H0. Otherwise, fail to reject H0.
a P(Type I Error) level of significance
29
One Sided Tests (Right and Left)
Ha p gt p0
Ha p lt p0
a
30
Two Sided Test

• All tests use the same test statistic.
• For all tests, Reject H0 when the observed test
  statistic is in the rejection region.

Ha p ? p0
a/2
31
Test Statistic
Test Statistic
In this case, the test statistic (TS) has not
gone into this red region (critical region) so we
fail to reject H0.
In this case, the TS is in the critical region,
therefore we will reject H0.
32
ß
33
Equivalence of Confidence Intervals and
Two-tailed Tests

• The null hypothesis Ho ??0 versus alternative
  Ha ???0 is rejected at an a level of
  significance if and only if the hypothesized
  value falls outside a
• (1-?)100 confidence interval for ?.

34
8.3 Testing a Population Mean µ

• The same general principles apply as they
• did for tests about p.
• We need a test statistic.
• We need to know the distribution of the test
  statistic.
• Compute the p-value and compare it to a.

35

• If s is known or ngt30, using Z-test. The test
  statistic is
• Use a Z (standard normal)
• to obtain p-values and
• critical values.

• If s is unknown, using T-test. The test statistic
  is
• Use a t distribution with n-1
• Degrees of freedom obtain
• p-values and critical values.

36
Note

• Whether you have a test about p or µ,
• you always reject H0 if p-value lta.

37
Example 8.5

• Suppose the researcher selects a random sample of
  100 county residents and finds that their average
  per capita income is 16,200. Suppose we
  know ?4,000, Is the evidence sufficient to
  suggest that the mean capita income of the
  country residents is greater than 15,000.

38

• P-value0.0013
• Based on the result of sample of 200 residents,H0
  is rejected. i.e. there is significant evidence
  that the mean capital income of the country
  residents is greater than 15000

39
Example 8.6

• A factory makes a certain computer part that,
  according to specifications, must have a mean
  length of 1.5 centimeters. In a random sample of
  16 parts from a shipment, the average length was
  found to be 1.56 centimeters and the sample
  standard deviation was 0.09 centimeter. Should
  this shipment be rejected based on the level of
  significance .05?

40

• P-value.0174lt0.05
• Based on a random sample 16 parts, reject H0 at
  the 5 level of significance. i.e. there is
  significant evidence that the shipment should be
  rejected.

41
Exercise 8.2

• The scores on a college placement exam in
  mathematics are assumed to be normally
  distributed with a mean of 70 and a standard
  deviation of 18. The exam is given to a random
  sample of 50 high school seniors who have been
  admitted to college. Their average score on the
  exam was 67. Is the evidence sufficient to
  suggest that the population mean score is lower
  than or equal to 70?

42

• P-value.1190
• Fail to reject H0,i.e. retain H0

43
Exercise 8.3

• To justify raising its rates, an insurance
  company claims that the mean medical expense for
  all middle-class families is at least 700 per
  year. A survey of 100 randomly selected
  middle-class families found that the mean
  medical expense for the year was 670 and the
  standard deviation was 140. Assuming that the
  tails of the distribution of medical expenses are
  not usually long, is there any evidence that the
  insurance company is misinformed?

44

• P-valuelt0.02
• Reject H0