Reciprocal Trigonometry Functions

1
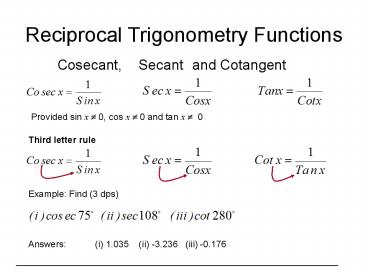
Reciprocal Trigonometry Functions
Cosecant,
Secant
and Cotangent
Provided sin x ? 0, cos x ? 0 and tan x ? 0
Third letter rule
Example Find (3 dps)
Answers
(i) 1.035 (ii) -3.236 (iii) -0.176
2
Graphs of cosec, sec and cot
The graphs of the reciprocal functions can be
found by taking the corresponding sine, cosine
and tangent graph and calculating the reciprocals
of each point on the graph.
3
Graphs of cosec, sec and cot
4
Graphs of cosec, sec and cot
5
Examples
Find the exact values of
Answers
6
Examples
Given that sin A 4/5, where A is obtuse, and
cosB ?3/2, where B is acute, find the exact
values of
Answers
7
Trigonometric Identities
8
Examples
Prove that (1 cos A)(1 sec A) ?? sin A tan A
L.H.S.
(1 cos A)(1 sec A) 1 sec A cos A Cos A
sec A
1 sec A cos A - 1
sec A cos A
sin A tan A
R.H.S.
9
Examples
Prove that cot A tan A ? sec A cosec A
L.H.S.
R.H.S.
10
Examples
R.H.S.
R.H.S.
11
Solving equations
Solve 2 tan2 x 7 sec x 8 0 for 0 ? x ?
360?
2 (sec2x 1) 7 sec x 8 0
2 sec2x 2 7 sec x 8 0
2 sec2x 7 sec x 6 0
(2 sec x 3)(sec x 2) 0
sec x 3/2 or sec x 2
cos x 2/3 or cos x ½
x 48.2 or x 60
or x 360 48.2 or x 360 - 60
complete solution x 48.2? or 60? or 300? or
311.8?
12
Solving equations
Solve 2 cos x cot x for 0 ? x ? 360?
2 cos x cos x/ sin x
2 cos x sin x cos x
2 cos x sin x cos x 0
cos x(2 sin x 1) 0
cos x 0 or sin x ½
cos x 0 ? x 90? or 270?
sin x ½ ? x 30? or 330?
complete solution x 30? or 90? or 270? or
30?
13
Solving equations
Solve 3 cot2 x 10 cot x 3 0 for 0 ? x
? 2?
(3 cot x - 1)(cot x 3) 0
cot x 1/3 or cot x 3
? tan x 3 or tan x 1/3
tan x 3 ? x 1.24c or 4.39c
tan x 1/3 ? x 0.32c or 3.46c
complete solution x 0.32c or 1.24c or
3.46c or 4.39c
14
Solving equations
Solve 5 cot2 x 2 cosec x 2 0 for 0 ? x
? 2?
5(cosec2 x 1) 2 cosec x 2 0
5cosec2 x 5 2 cosec x 2 0
5cosec2 x 2 cosec x - 3 0
sin x -5/3 not possible or sin x 1 ? x
?/2
15
Additional formulae
sin (A B) sin A cos B sin B cos A
sin (A - B) sin A cos B - sin B cos A
cos (A B) cos A cos B - sin A sin B
cos (A - B) cos A cos B sin A sin B
16
Examples
Find the exact value of sin 75?
sin (A B) sin A cos B sin B cos A
sin (30 45) sin 30 cos 45 sin 45 cos 30
17
Examples
Express cos (x ?/3) in terms of cos x and sin x
cos (A B) cos A cos B - sin A sin B
cos (x ?/3) cos x cos ?/3 - sin ?/3 sin x
18
Examples
L.H.S.
R.H.S.
19
Double angle formulae
sin (A B) sin A cos B sin B cos A
sin (A A) sin A cos A sin A cos A
sin 2A 2 sin A cos A
cos (A B) cos A cos B - sin A sin B
cos (A A) cos A cos A- sin A sin A
cos (A A) cos2A - sin2A
cos 2A cos2A - sin2A
cos 2A 2cos2A - 1
cos 2A 1 2sin2A
20
Double angle formulae
21
Examples
Given that cos A 2/3, find the exact value of
cos 2A.
cos 2A 2cos2A - 1
Given that sin A ¼ , find the exact value of
sin 2A.
sin 2A 2 sin A cos A
22
Solving equations
Solve cos 2A 3 4 cos A 0 for 0 ? x ? 2?
2 cos2A - 1 3 4 cos A 0
2 cos2A 4 cos A 2 0
cos2A 2 cos A 1 0
cos2A 2 cos A 1 0
(cos A 1)2 0
cos A - 1
? A ?
23
Solving equations
Solve sin 2A sin A for - ? ? x ? ?
2sin A cos A sin A
2 sin A cos A sin A 0
sin A(2 cos A 1) 0
? sin A 0 or cos A ½
sin A 0 ? A - ? or 0 or ?
cos A ½ ? A - ?/3 or ?/3
Complete solution A - ? or - ?/3 or 0 or ?/3
or ?
24
Solving equations
Solve tan 2A 5 tan A 0 for 0? x ? 2?
tan A 0 ? A 0 or ? or 2?
7 5tan2 A 0? tan A ? ?7/5 ? A 0.97 ,
2.27, 4.01 or 5.41c
Complete solution A 0.97 , 2.27, 4.01, 5.41c 0,
? or 2?
25
Harmonic form
If a and b are positive
a sin x b cos x can be written in the form R
sin( x ? )
a sin x - b cos x can be written in the form R
sin( x - ? )
a cos x b sin x can be written in the form R
cos( x - ? )
a cos x - b sin x can be written in the form R
cos( x ? )
26
Examples
Express 3 cos x 4 sin x in the form R cos( x -
? )
R cos( x - ? ) R cos x cos ? R sin x sin ?
3 cos x 4 sin x R cos x cos ? R sin x sin ?
R cos ? 3 1 R sin ? 4 2
12 22 R2 sin2 x R2 cos2 x 32 42
R2(sin2 x cos2 x ) 32 42
R2 32 42 25 ? R 5
2 ? 1 tan ? 4/3 ? ? 53.1
3 cos x 4 sin x 5 cos( x 53.1? )
27
Examples
Express 12 cos x 5 sin x in the form R sin( x
? )
R sin( x ? ) R sin x cos ? R cos x sin ?
12 cos x 5 sin x R sin x cos ? R cos x sin ?
R cos ? 12 1 R sin ? 5 2
12 22 R2 cos2 x R2 sin2 x 122 52
R2(cos2 x sin2 x ) 122 52
R2 122 52 169 ? R 13
2 ? 1 tan ? 5/12 ? ? 22.6
12 cos x 5 sin x 13 sin( x 22.6? )
28
Examples
Express cos x - ?3 sin x in the form R cos( x
? )
R cos( x ? ) R cos x cos ? - R sin x sin ?
cos x - ?3 sin x R cos x cos ? - R sin x sin ?
R cos ? 1 1 R sin ? ?3
2
12 22 R2 cos2 x R2 sin2 x 12
(?3 ) 2
R2(cos2 x sin2 x ) 12 3
R2 1 3 4 ? R 2
2 ? 1 tan ? ?3 ? ? 60
cos x ?3 sin x 2 cos( x 60? )
29
Solving equations
Solve 7 sin x 3 cos x 6 for 0? x ? 2?
R sin( x ? ) R sin x cos ? R cos x sin ?
7 sin x 3 cos x R sin x cos ? R cos x sin ?
R cos ? 7 1 R sin ? 3 2
R2 72 32 ? R 7.62
2 ? 1 tan ? 3/7 ? ? 0.405c
(Radians)
7 sin x 3 cos x 7.62 sin( x 0.405)
7.62 sin( x 0.405 ) 6 ? x 0.405
sin-1(6/7.62)
x 0.405 0.907 or 2.235
x 0.502c or 1.830c