Molecular Dynamics: Introduction

1
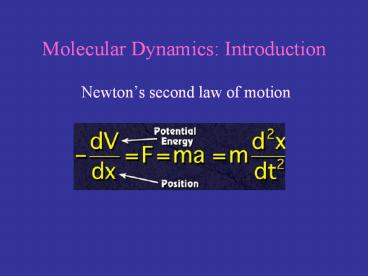
Molecular Dynamics Introduction

• Newtons second law of motion

2
Molecular Dynamics Introduction

• We need to know
• The motion of the
• atoms in a molecule, x(t)
• and therefore,
• the potential energy, V(x)

3
Molecular Dynamics Introduction

• How do we describe the potential energy V(x) for
  a
• molecule?
• Potential Energy includes terms for
• Bond stretching
• Angle Bending
• Torsional rotation
• Improper dihedrals

4
Molecular Dynamics Introduction

• Potential energy includes terms for (contd.)
• Electrostatic
• Interactions
• van der Waals
• Interactions

5
Molecular Dynamics Introduction

• Equation for covalent terms in P.E.

6
Molecular Dynamics Introduction

• Equation for non-bonded terms in P.E.

7
Molecular Dynamics Introduction

• Each of these interactions exerts a force onto a
  given atom of the molecule
• The total resulting force on each atom is
  calculated using the PE function

Knowing the force on an atom, its movement due to
the force is then calculated
8
Molecular Dynamics Introduction

• To do this, we should know
• at given time t,
• initial position of the atom
• x1
• its velocity
• v1 dx1/dt
• and the acceleration
• a1 d2x1/dt2 m-1F(x1)

9
Molecular Dynamics Introduction

• The position x2 , of the atom after time interval
  ?t would be,
• and the velocity v2 would be,

10
How a molecule changes during MD
11
Molecular Dynamics Introduction

• In general, given the values x1, v1 and the
  potential energy V(x), the molecular trajectory
  x(t) can be calculated, using,

12
DOCKING
13
DOCKING