Sect. 1 Basics of Thermodynamics

1
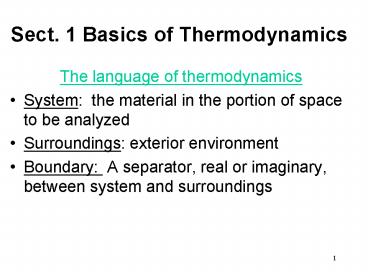
Sect. 1 Basics of Thermodynamics

• The language of thermodynamics
• System the material in the portion of space to
  be analyzed
• Surroundings exterior environment
• Boundary A separator, real or imaginary,
  between system and surroundings

2
Thermodynamic Systems

• Closed fixed mass (solid or fluid) within the
• Open (flow) A volume with partly solid
  boundaries and imaginary boundary sections
  through which fluid passes

surroundings
surroundings
2
3
Example of a more complex system gas-cylinder
blowdown
4
Boundary

• the boundary encloses the system
• Adiabatic (insulated) does not allow heat to pass
• Rigid cannot expand or contract (no work done)
• Isolated exchanges neither heat nor work with
  the surroundings (rigid and adiabatic)

Surroundings

• space outside the system boundary
• exchanges heat and work with system through
  boundary
• examples
• thermal reservoir exchanges heat with system
• work devices spring, piston, weight, atmosphere

5
What does a system consist of?

• Components distinct chemical species
• single component pure substance
• 2. two components binary system
• a pure substance can become a binary system
• steam is a single component but at very high T,
  it dissociates into H2, OH, and O2 and becomes a
  two-component system (H and O)
• a binary system treated as a single component
• air is O2 N2, but at low T, is effectively a
  single component because composition doesnt
  change
• At very high temperatures, reaction produces NOx
• air is now a binary, with components N and
  O

6

• Multicomponent system three or more components
• Steel is an alloy of Fe, Ni, and Cr this is a
  nonreacting ternary. The concentrations of the
  species are independent (but their atom fractions
  must add to unity)
• At low temperature, a mixture of H2, CO2 and O2
  is a nonreacting ternary at high temperature, it
  is a reactive ternary (H, C, and O) with many
  chemical reactions

7
Phases regions of a system with uniform
properties

• phases are solid, liquid, or gas
• solids and liquids are collectively called
  condensed phases
• liquids and gases are collectively called fluid
  phases
• a gas phase can be called a vapor if it is
  condensible (e.g., H2O)
• a phase can contain one or more components

8
Phases (cont)

• a homogeneous system consists of a single phase
• a heterogeneous system consists of two or more
  phases separated by sharp interfaces
• a system may contain more than one liquid or
  solid phases, but only one gas (vapor) phase
  examples
• solid liquid (e.g., ice and water)
• two solids (e.g., ?-Zr and ?-Zr)
• two solids and a gas (e.g., Fe, FeO, and O2)
• gas liquid (e.g., liquid water and steam)
• two immiscible liquids (e.g., oil and water)

9
(No Transcript)
10
Thermodynamic properties single-component
(pure substance),

• characteristics that fix the condition of a
  system
• microscopic properties average of the quantum
  numbers of all atoms or molecules in a system
  statistical thermodynamics
• macroscopic properties (also called state
  variables) a few characteristics that determine
  the measurable gross condition of the system
  classical thermodynamics

11
Classification of thermodynamic properties (for
a pure or 1-component substance)

• Fundamental p,T, V, U, S
• Auxiliary (derived from fundamental) H, F, G
• Absolute p, T, v, s, CP, CV
• Relative (to a reference state) U, H, F, G
• Extensive (? amount) V, S, U, H, F, G
• Intensive p, T (v, u, s, h, f, g)
• Derivative CP, CV, a, b

12
fundamental macroscopic properties

• pressure (p) momentum transferred to walls by
  molecular impacts
• temperature (T) molecular speeds (gas) or
  amplitudes of atomic vibrations (solids)
• volume (V)
• internal energy (U) kinetic and potential
  energy contained in molecules or atoms
• entropy (S) measure of the degree of order of a
  system (disorder high S)

13
Auxiliary properties

• enthalpy H ? U pV
• - like internal energy, but automatically
  accounts for pV work
• - ?H (change in enthalpy) is the heat added in a
  constant-p process
• - for an open (flow) system, H replaces U in the
  1st Law

14
Auxiliary properties Free Energies

• Helmholz free energy F ? U TS - F is the
  link between statistical and classical
  thermodynamics (F is rarely used in purely
  classical approach)
• Gibbs free energy G ?? H TS
• - G is the criterion of equilibrium in chemical
  reactions
• - DG is the maximum work done (or needed) in a
  flow process.

15
Absolute and relative properties

• p, T, V, and S are absolute zero values are
  unique
• - absolute zero temperature 0 K
• - The absolute zeros of p and V are obvious
• - CP and CV are derivatives of relative
  properties
• - The absolute value of S comes from the 3rd
  Law The entropy of a solid is zero at 0 K.
  (all substances are solid at
  this temperature)
• U, H, F, G are relative i.e., they must be
  assigned a zero value at an arbitrary reference
  state (the same state for all four)

16
Extensive and Intensive properties

• Extensive value proportional to amount in
  system V, U, S, H, F, G
• Intensive value independent of the amount of
  material p, T
• For a one-component system (pure substance)
  extensive properties can be made intensive by
  dividing by the amount (n moles of substance)
• - v V/n v molar volume, or
  reciprocal of the
  molar density
• - u U/n s S/n h H/n g G/n

17
Derivative Properties

• Specific heats (heat capacities)
• CP (?h/?T)P - constant-pressure
• CV (?u/?T)V - constant-volume
• Coefficients of expansion
• a (1/v)(?v/?T)P - thermal expansion
• a(Hg) used for mercury thermometer
• b -(1/v)(?v/?p)T - compressibility

18
Units of thermodynamic properties(SI, or metric)

• pressure Pascal(Pa) Newton(N)/m2 10-5 atm
  temperature Kelvins (K) or degrees Celsius oC
  K 273 (strictly, not SI)
• volume cubic meters (m3) length in meters (m)
• U, H, F, G Joules(J or kJ), calorie or kcal also
  used
• - 1 cal 4.184 J 1 kcal 4.184 kJ
• KE ½mv2 kg-m2/s2 but from F ma, N kg-m/s2
• ? kg-m2/s2 N-m J

19
Thermodynamic State of a pure substance

• all properties fixed if any two are specified.
  (why 2? see later)
• To know all properties, two Equations of State
  (EOS) are needed
• - v(p,T) volumetric EOS
• - CP(T,p) or CV(T,v) thermal (EOS) -
  fixes s, u, h, g (with specification of a ref.
  state)

20
Heat (Q)

• Energy exchanged between system and surroundings
  (reservoirs) due to a DT
• Mechanisms (not thermodynamic)
• - conduction molecular motion
• - convection bulk fluid movement
• - radiation electromagnetic fields
• Heat is not a thermodynamic property it causes
  changes in them

21
Work (W)

• Expansion (pV ) W F?x (F/A)(A?x) p?V
• Shaft rotation of a shaft by a moving fluid
• Electrical flow of electrons down a potential
  gradient
• External all F?x except p?V
• why? p?V is often not useful work just
  pushing back or being pushed by the surrounding
  atmosphere
• Work is not a thermodynamic property, but can
  change them
• All forms of work are theoretically
  interconvertible

22
Thermodynamic Processes

• Cannot infer Q from this diagram
• Path depends on how T varies with V.

• -Process - change in p-V-T state of system due to
  exchange of heat and/or work with the
  surroundings.
• - Plot process path on a
• pressure-volume graph
• state of system at 2 is
• independent of path (A or B)
• but, Q and W are different for each path

23
ISO processes

• In most processes, one property is constant

24
Reversible Processes

• Internal in the system
• External in the surroundings
• Work done by the system is the same as the work
  done on the surroundings
• For the same initial and final states, work done
  reversibly is always gt work done irreversibly
• Requirements of reversibility
• - very slow moves through equilibrium states
  
• - in fluids, no turbulence
• - no friction
• - infinitesimal T Tsurr for heat p psurr
  for work
• -

25
Example Reversible Isothermal compression of an
ideal gas

• add small weights (total mass m) so that descent
  of
• piston is gentle remove heat with very small T -
  Tsurr
• This is also Wsurr, the work done by the
  surroundings

26
Irreversible version

• add single block of mass m rapid descent
• violent bouncing of piston until final state
  reached
• Cannot integrate pdV

27
Work to compress irreversibly

• the work done by the surroundings can be
  calculated
• Wsurr psurr(Vo-Vf) mg (Vo-Vf)/A
• Force balance on final state pf psurr mg/A
• Combine
• Wsurr - pf (Vo-Vf) - pfVf(Vo/Vf - 1) -nRT
  (Vo/Vf - 1)
• For Vo/Vf 3
• The surroundings do less work in the reversible
  than in the irreversible process

28
Internal equilibrium (system)

• Single phase
• - no change of pressure, temperature or
  composition with time (mechanical, thermal
  chem. equilibrium)
• - no gradients of any properties, except at
  interfaces between phases
• Multiphase - each phase must have
• - same temperature (thermal equilibrium)
• - same pressure (mechanical equilibrium)
• - same chemical potential (chemical equilibrium)

29
External Equilibrium(between system and
surroundings)

• Both have the same pressure (unless the boundary
  is rigid)
• Both have the same temperature (unless the
  boundary is adiabatic)
• No work can be performed by or on the system (no
  Dp, DT or Dm)

30
Constraints and equilibrium

• Constraining a system means fixing two of its
  properties
• If U and V are fixed (isolated system),
  equilibrium occurs when the entropy is a maximum
  (mainly of theoretical importance)
• If p and T are fixed, equilibrium occurs when the
  Gibbs free energy is a minimum (useful for phase
  equilibrium and chemical equilibrium)

31
The First Law of Thermodynamics

• Cannot be derived from any fundamental principle
  (it is one)
• Has never failed an experimental test in 150
  years
• Comes in two versions
• - within a system the 1st law
• - between system and surroundings or two
  systems Law of Conservation of Energy

32
1st Law

• relates changes in system energy to heat and
  work
• ?U ?(PE) ?(KE) Q W WS Wel
• U internal energy
• PE potential energy
• KE kinetic energy
• Q heat (positive if added to the system)
• W expansion (pV) work if done by the system
• WS shaft work (rotation of a shaft)
• Wel electrical work (charging a battery)
• Heat and work are equivalent in the 1st law
• Even though Q and W are path-dependent, ?U is not

33
Conservation of Energy

• Heat and work are exchanged between the system
  and the surroundings
• DUsys (Q W)sys
• DUsurr -(Q W)surr
• Add the 1st Laws for system surroundings
• DUsys DUsurr 0
• This is the Law of Conservation of Energy

34
Where the 1st Law is inadequate

• Consider an isolated system consisting of two
  rigid subsystems of different temperatures that
  communicate thermally
• Energy conservation and the 1st Law yield
• ?U1 ?U2 Q1 Q2 0 or Q1 - Q2
• Either Q1 or Q2 must be negative (i.e., one of
  the two arrows must be reversed) the 1st Law
  cannot tell which one, but the 2nd Law can.

35
The Second Law of Thermodynamics

• The 2nd Law was discovered by Clausius from
  numerous observations showing that if a process
  is reversible, is
  path-independent.
• Any quantity whose change is independent of the
  path must be a thermodynamic property as in the
  1st Law, where Q W is path-independent
• Clausius called the property entropy, with the
  symbol S

36
Irreversible processes

• For irreversible processes, the equality no
  longer holds. Instead
• In irreversible processes, entropy is created!
• Other forms of the 2nd Law
• dS ?

37
law of entropy production

• Entropy production is to the 2nd Law as
• energy conservation is to the 1st Law
• add 2nd Law for system and surroundings
  (TsurrgtTsys)
• entropy can be produced but never
  destroyed

38
The direction of heat flow revisited

• begin with ?S1 ?S2 gt 0
• but DS1 Q1/T1 and DS2 Q2/T2 (heat flow to and
  from reservoirs is reversible)
• T1 ? T2, is the source of irreversiblity
• Q1/T1 Q2/T2 gt 0 from 1st Law Q1
  -Q2
• 
  
• If T1gtT2, then Q2 gt0
• and Q1lt0

39
How to calculate system entropy change for an
irreversible process

• Example doubling the volume of an ideal gas
• in an isolated system (DU 0)
• Irreversible version
• initial state (To) final
  state(To)
• Boltzmann eqn SnNAvklnW DSnRln(Wf/Wo) nRln2

40

• Reversible version

41

• DU 0 for the reversible process as well
• from the 1st Law, Q W
• work can be calculated for the reversible
  process only
• From the 2nd Law
• DS Q/T nRln(Vf/Vo) nRln2
• Since entropy is a thermodynamic property, the
  path used to compute it is immaterial
• ?S computed for the reversible process is the
  same as the ?S for the irreversible process
• (but, work was done in reversible process)

42
Entropy, Free Energy and Equilibrium

• for a closed system du ?q - ?w(PV) -?wext

For reversible process Tds pdv

• Electrical (battery)
• Chemical (ATP?muscle)

du Tds pdv - ?wext
d small increment of heat or work d small
increment of a thermodynamic property

• isolated system, dv du 0

• Equilibrium system cannot perform external work,
  or dwext 0

s
equilibrium state
State of system
At equilibrium with u v constant the entropy is
a maximum
43
Thermal equilibration of identical solids
energy conservation

T1
T2
Tf
Tf
Q
DUTot?U1?U2 0
CV(Tf T1) CV(Tf T2) 0
Tf ½(T1 T2)
?STot ?S1 ?S2
Eliminate Tf
T2/T1 ?STot/CV 0.9 0.0028
1.0 0 1.1 0.0023
Thermal equilibration results in an increase in
the entropy of the isolated system
44
Enthalpy
h ? u pv ? dh du pdv vdp ? use du
equation
dh Tds vdp - ?Wext
Helmholz free energy
f ? u Ts ? df du Tds sdT ? use du
equation
df - pdv sdT - ?Wext
Gibbs free energy
g ? h Ts ? dg dh Tds sdT ? use dh
equation
dg vdp sdT - ?Wext
45
Neglecting external work
du Tds pdv

• These were derived assuming reversible ?q and
  ?W(pv)

dh Tds vdp
df - pdv sdT

• However, since they involve only state functions
  (properties), they are valid for any process

dg vdp sdT

• Collectively, they are called
• Fundamental differentials, or
• Tds equations (first two), or
• Gibbs equations

46
Equilibrium at constant T and p
dg vdp sdT - ?Wext
with dT dp 0

• Reversible non-pv work at constant p and T

• System is at equilibrium when dwext 0

equilibrium state
g
State of system
At equilibrium with p T constant the Gibbs free
energy is a minimum
47
The Phase Rule

• Fixes how many properties can be varied for a
  given number of components (C) and phases (P)
• The allowable variable properties are called
  degrees of freedom (f)
• Single component (C 1) 1 phase (P 1) f 2
• Single component (C 1) 2 phases (P 2) f 1
• e.g. the vapor pressure of a condensed phase
  F(T)
• Single component (C 1) 3 phases (P 3) f 0
• e.g. the triple point where gas, liquid solid
  coexist
• Two components (C 2) 3 phases (P 3) ? 1
• e.g. a metal, its oxide and O2 gas at each T,
  there is a unique at which M and MOx
  coexist

f C 2 P - Nrxn
48
(No Transcript)