THE STATE UNIVERSITY OF NEW JERSEY

1
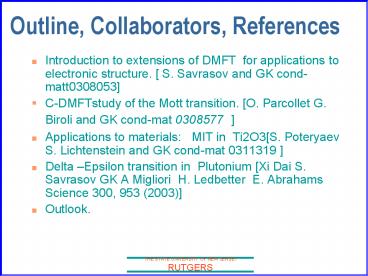
Outline, Collaborators, References

• Introduction to extensions of DMFT for
  applications to electronic structure. S.
  Savrasov and GK cond-matt0308053
• C-DMFTstudy of the Mott transition. O. Parcollet
  G. Biroli and GK cond-mat 0308577
• Applications to materials MIT in Ti2O3S.
  Poteryaev S. Lichtenstein and GK cond-mat 0311319
  
• Delta Epsilon transition in Plutonium Xi Dai
  S. Savrasov GK A Migliori H. Ledbetter E.
  Abrahams Science 300, 953 (2003)
• Outlook.

2

Dynamical Mean Field Theory (DMFT) Cavity
Construction A. Georges and G. Kotliar PRB 45,
6479 (1992).
3
DMFT Cavity Construction. A. Georges and G.
Kotliar PRB 45, 6479 (1992). First happy marriage
of atomic and band physics.
Reviews A. Georges G. Kotliar W. Krauth and M.
Rozenberg RMP68 , 13, 1996 Gabriel Kotliar and
Dieter Vollhardt Physics Today 57,(2004)
4
EDMFT H. Kajueter Rutgers Ph.D Thesis 1995
Si and Smith PRL77, 3391(1996) R. Chitra and G.
Kotliar PRL84,3678 (2000)
5
Site? Cell. Cellular DMFT. C-DMFT. G.
Kotliar,S.. Savrasov, G. Palsson and G. Biroli,
Phys. Rev. Lett. 87, 186401 (2001)
t(K) hopping expressed in the superlattice
notations.

• Other cluster extensions (DCA Jarrell
  Krishnamurthy, Katsnelson and Lichtenstein
  periodized scheme, Nested Cluster Schemes
  Schiller Ingersent ), causality issues, O.
  Parcollet, G. Biroli and GK cond-matt 0307587
  (2003)

6
Two paths for ab-initio calculation of electronic
structure of strongly correlated materials
Crystal structure Atomic positions
Model Hamiltonian
Correlation Functions Total Energies etc.
DMFT ideas can be used in both cases.
7
LDADMFT V. Anisimov, A. Poteryaev, M. Korotin,
A. Anokhin and G. Kotliar, J. Phys. Cond. Mat.
35, 7359 (1997). A Lichtenstein and M. Katsnelson
PRB 57, 6884 (1988).

• The light, SP (or SPD) electrons are extended,
  well described by LDA .The heavy, D (or F)
  electrons are localized treat by DMFT.
• LDA Kohn Sham Hamiltonian already contains an
  average interaction of the heavy electrons,
  subtract this out by shifting the heavy level
  (double counting term)
• Kinetic energy is provided by the Kohn Sham
  Hamiltonian (sometimes after downfolding ). The U
  matrix can be estimated from first principles of
  viewed as parameters. Solve resulting model
  using DMFT.
• Impurity Solvers QMC-IPT-NCA..

8
Functional formulation. Chitra and Kotliar Phys.
Rev. B 63, 115110(2001), Ambladah et. al. Int.
Jour Mod. Phys. B 13, 535 (1999) Savrasov and
Kotliarcond- matt0308053 (2003).
IrgtR, rgt
Double loop in Gloc and Wloc
9
Next Step GWEDMFT S. Savrasov and GK.(2001).
P.Sun and GK. (2002). S. Biermann F. Aersetiwan
and A.Georges . (2002). P Sun and G.K (2003)
W
EH
W
Implementation in the context of a model
Hamiltonian with short range interactions.P Sun
and G. Kotliar cond-matt 0312303 or with a
static U on heavy electrons, without self
consistency. Biermann et.al. PRL 90,086402
(2003)
10
Self-Consistency loop. S. Savrasov and G.
Kotliar (2001) and cond-matt 0308053
E
U
DMFT
11
How good is approach ?

• It becomes exact as the coordination number
  increases or in the limit of infinite dimensions
  introduced by Metzner and Vollhardt. PRL 62,34,
  (1989).
• How good is it in low dimensions ? Promising
  recent developments from theory and experiments.

12
Schematic DMFT phase diagram and DOS of a
partially frustrated integer filled Hubbard model
and pressure driven Mott transition.
S Lefebvre et al. PRL (2000)
13
Recent Experiments support qualitative single
site DMFT predictions
Limelette et. al.(2003) Ito et. al. (1995)
Mo et al., Phys. Rev.Lett. 90, 186403 (2003).
14
Theoretical issue is there a Mott transitionin
the integer filled Hubbard model, and is it well
described by the single site DMFT ?
YES! Parcollet Biroli Kotliar cond-matt 0308577
Study frustrated t t model t/t.9
15
Evolution of the k resolved Spectral Function
at zero frequency.

• Qualitative effect, formation of hot regions!
• D wave gapping of the single particle spectra as
  the Mott transition is approached.
• Very strong k dependece near the trasition.

U/D2
U/D2.25
Uc2.35-.05, Tc/D1/44
16
Ti2O3 Coulomb or Pauling

• LTS 250 K, HTS 750 K.

C.E.Rice et all, Acta Cryst B33, 1342 (1977)
17
Ti2O3.

• Isostructural to V2-xCrxO3. Al lot of the
  qualitative physics of the high temperature part
  of the phase diagram of V2O3 can be understood
  within single site DMFT. Is this true in Ti2O3?
• Band Structure Calculations good metal. L.F.
  Mattheiss, J. Phys. Condens. Matter 8, 5987
  (1996) .Unrestricted Hartree Fock calculations
  produce large antiferromagnetic gap. M. Cati,
  et. al. Phys. Rev. B. f55 , 16122 (1997).

18
2site-Cluster DMFT with intersite Coulomb
U 2, J 0.5, W 0.5 ß 20 eV-1, LT structure
U 2, J 0.5, W 0.5 ß 10 eV-1, HT structure
A. Poteryaev
19
Pauling and Coulomb Ti2O3S. Poteryaev S.
Lichtenstein and GK cond-mat 0311319
Dynamical Goodenough-Honing picture
20
Conclusion Ti2O3

• 2 site cluster DMFT describes the MIT in Ti2O3.
• Different from V2O3 where single site DMFT works
  well, and cluster corrections are small A.
  Poteryaev
• It requires the Coulomb interactions, and a
  frequency dependent enhancement of the a1g-a1g
  hopping, induced by the Coulomb interactions.
  Haldane Ph.D thesis, Q Si and GK 1993
  .Dynamical Pauling-Goodenough mechanism is able
  to trigger the MIT at low enough temperatures.
• Coulomb and Pauling synergistically cooperate.

21
Pu phases A. Lawson Los Alamos Science 26,
(2000)
LDA underestimates the volume of fcc Pu by
30 Predicts magnetism in d Pu and gives
negative shear Core-like f electrons
overestimates the volume by 30
22
The delta epsilon transition

• The high temperature phase, (epsilon) is body
  centered cubic, and has a smaller volume than the
  (fcc) delta phase.
• What drives this phase transition?
• Having a functional, that computes total energies
  opens the way to the computation of phonon
  frequencies in correlated materials (S. Savrasov
  and G. Kotliar PRL 90, 056401)

23
Total Energy as a function of volume for Pu
(after Savrasov, Kotliar, Abrahams, Nature
,410,793, (2001)
24
DMFT Phonons in fcc d-Pu
(after Dai, Savrasov, Kotliar,Ledbetter,
Migliori, Abrahams, Science, 9 May 2003)
(experiments from Wong et.al, Science, 22 August
2003)
25
DMFT Phonons in bcc e-Pu
26
Phonon entropy drives the epsilon delta phase
transition

• Epsilon is slightly more delocalized than delta,
  has SMALLER volume and lies at HIGHER energy than
  delta at T0. But it has a much larger phonon
  entropy than delta.
• Different from Cerium see Jeong et. al.
  cond-mat/0308416
• At the phase transition the volume shrinks but
  the phonon entropy increases.
• Estimates of the phase transition following
  Drumont and Ackland et. al. PRB.65, 184104
  (2002) (and neglecting electronic entropy).
  TC 600 K.

27
Outlook

• Dynamical mean field theory, local reference
  for correlated electron systems.
• Analogy to FLT, DFT. The need of simpler
  reference frames for thinking about complex
  problems.
• Future directions downfolding and RG,
  algorithmic speedups.
• While a general method is under construction,
  the extensions described in this talk, already
  allow to perform quantitative calculations and
  obtain quantitative insights.

28
Conclusion

• Introduction to DMFT and its extensions.
  Flexibility of a local approach.
• DMFT describes well the Mott transitions.
  Formation of hot and cold regions near FS.
• MIT in Ti2O3 cluster DMFT .Dynamical
  Pauling-Coulomb mechanism.
• Delta-Epsilon Plutonium. Correlations and phonon
  entropy.